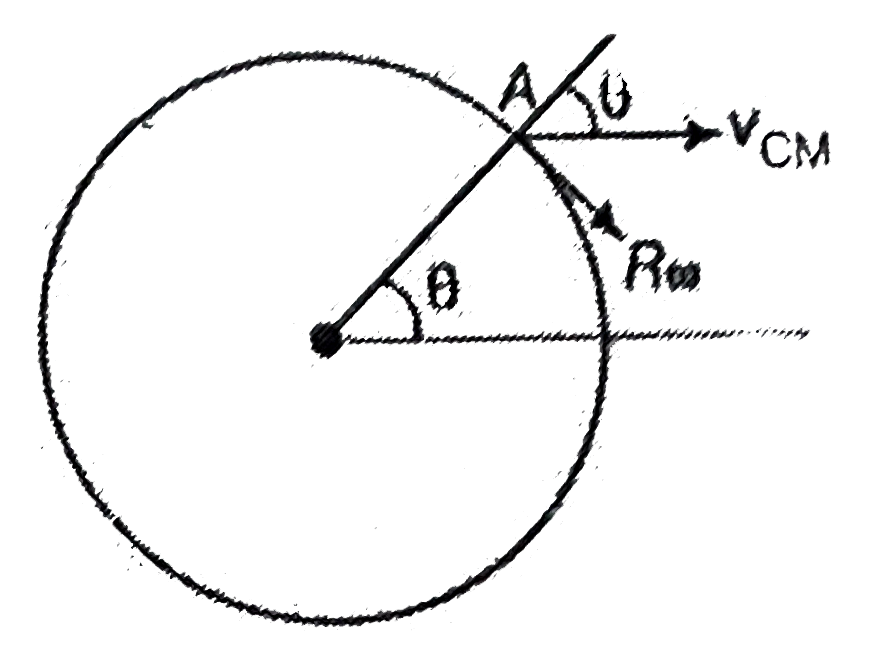
A sphere of radius R is rolling on a rough horizontal surface. The magnitude of velocity of A with respect to ground will be
√2vCM
2VCMsinθ
√2vCM√1+sinθ
No sufficient information
c) Here vCM=Rω
Therestant–––––velocityofp∮Ais<br>v_(A) = sqrt(v_(CM)^(2) + (Romega)^(2) + 2v_(CM)(Romega)cos(90-theta))<br>=sqrt(v_(CM)^(2) + v_(CM)^(2) + 2v_(CM)^(2)sintheta)<br>sqrt(2)v_(CM)sqrt(1+sintheta))

Topper's Solved these Questions
- ROTATIONAL MOTIONBOOK - BITSAT GUIDECHAPTER - ROTATIONAL MOTIONEXERCISE - BITSAT Archives
9 Videos
- NEWTONS LAWS OF MOTION AND FRICTIONBOOK - BITSAT GUIDECHAPTER - NEWTONS LAWS OF MOTION AND FRICTIONEXERCISE - BITSAT Archives
23 Videos
- SCALARS AND VECTORSBOOK - BITSAT GUIDECHAPTER - SCALARS AND VECTORSEXERCISE - All Questions
34 Videos
Similar Questions
Knowledge Check
A disc of radius R rolls on a rough horizontal surface. The distance covered by the point A in one revolution is
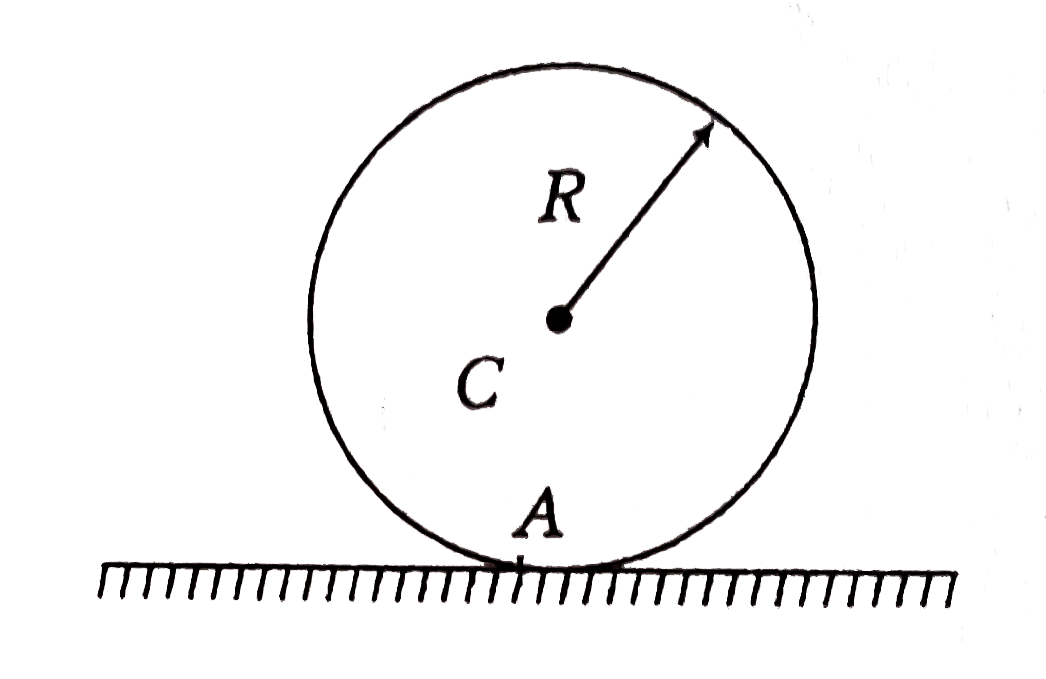 A2πRB2RC8RDπR
A2πRB2RC8RDπRA disc is rolling on a rough horizontal surface. The instantaneous speed of the point of contact during perfect rolling is zero with respect to ground.
The force of friction can help in achieving pure rolling condition.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are reason are true but reason is not the correct explanation of assertion.CIf assertion is true but reason is falseDIf both assertion and reason are false.A solid sphere of radius r r is rolling on a horizontal surface. The ratio between the rotational kinetic energy and total energy.
A57B27C12D17
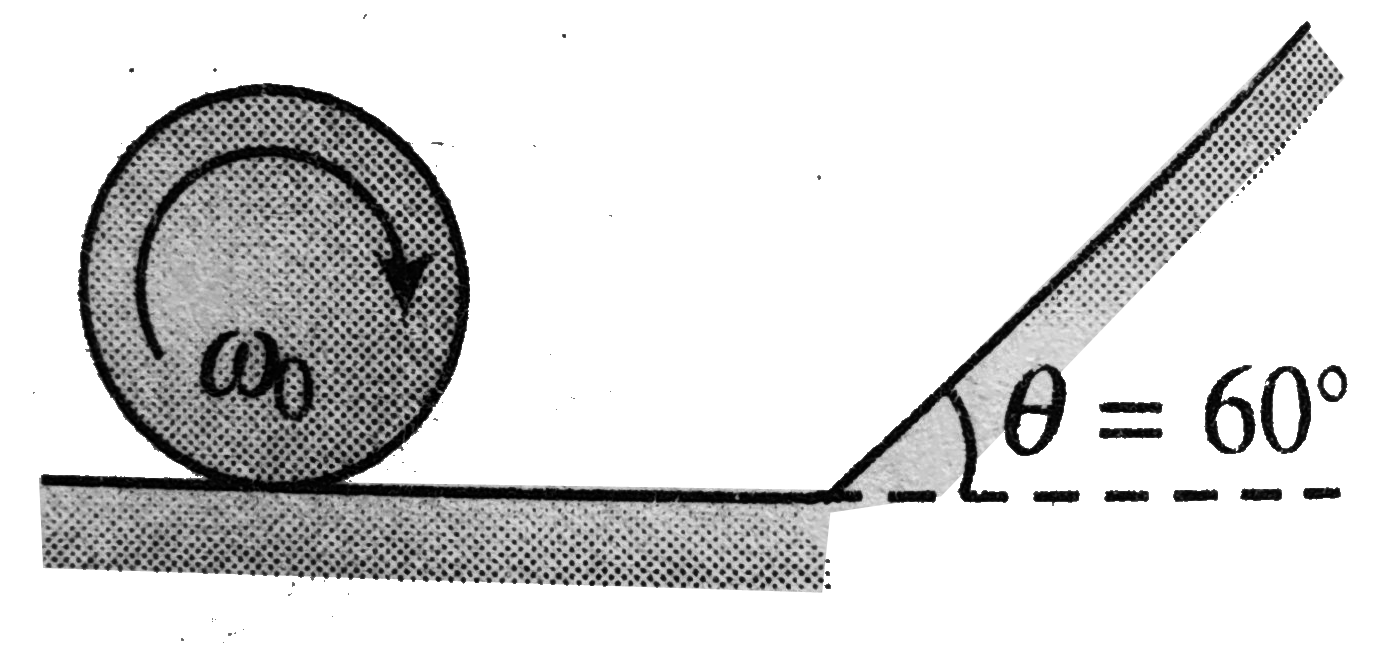
A uniform solid sphere of radius R, rolling without sliding on a horizontal surface with an angular velocity ω0, meets a rough inclined plane of inclination θ=60∘. The sphere starts pure rolling up the plane with an angular velocity ω Find the value of ω.
View SolutionA sphere is rolling down on a sufficiently rough inclined surface.
View SolutionA disc of radius R is moving on a rough horizontal surface with velocity v and angular speed ω at an instant as shown in figure. Choose the correct statement.

View SolutionA ring of radius R rolls without slipping on a rough horizontal surface with a constant velocity. The radius of curvature of the path followed by any particle of the ring at the highest point of its path will be :
 .
.
View SolutionA sphere is performing pure rolling on a rough horizontal surface with constant angular velocity. Frictional force acting on the sphere is zero.
Velocity of contact point is zero.
View Solution
