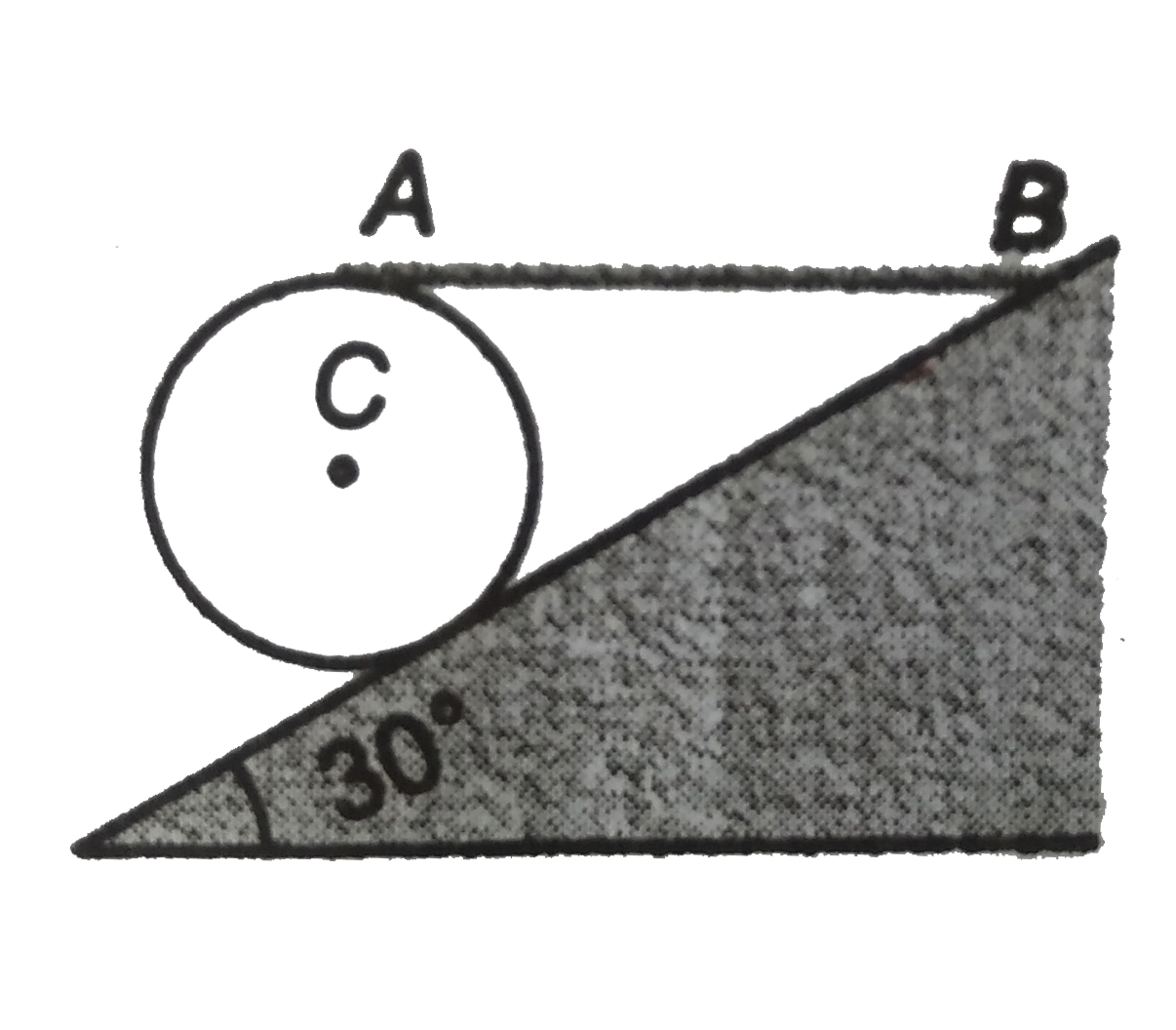
A sphere of weight w=100N is kept stationary on a rought inclined plane by a horizontal string AB as shown in figure. Find
(a)tension in the string,
(b) force of friction on the sphere and
(c)normal reation on the sphere by the plane.
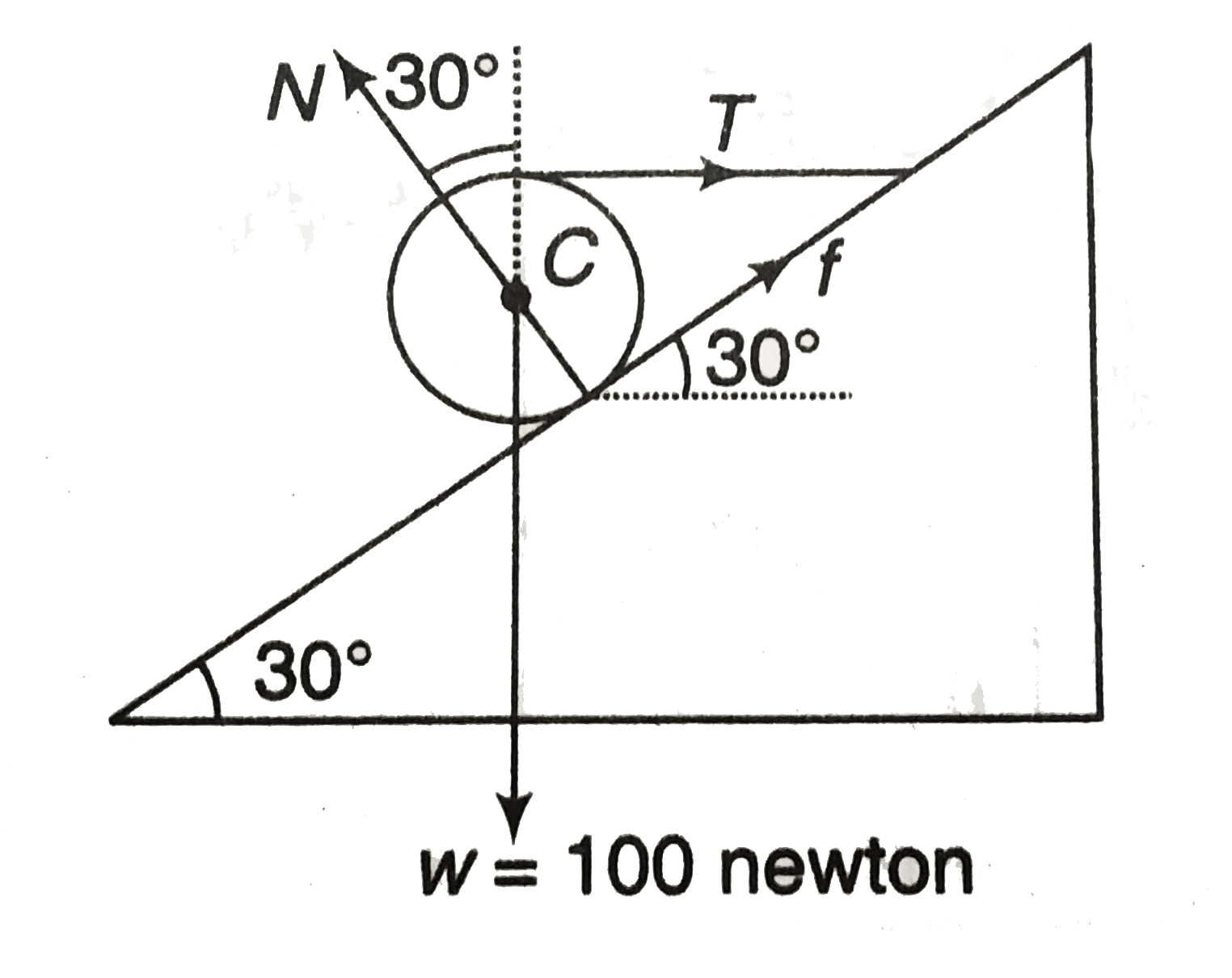
w=100N
∑ (force in horizontal direction) = 0
T+fcos30∘=Nsin30∘ ...(i)
∑ (force in horizontal direction) = 0
Ncos30∘+fsin30∘=100 ...(ii)
∑ (moment of all forces about C) = 0
∴(T)(R)=f(R)
T=f ...(iii)
On solving these three equation, we get
T=f=26.8N
N=100newton
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Exercise 8.2
9 Videos
- LAWS OF MOTIONBOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Exercise 8.3
8 Videos
- LAWS OF MOTIONBOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Miscellaneous Examples
9 Videos
- KINEMATICS 1BOOK - DC PANDEYCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
- LAWS OF THERMODYNAMICSBOOK - DC PANDEYCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Subjective
18 Videos
Similar Questions
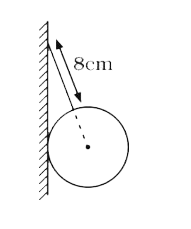
A uniform sphere of weight W and radius 5 cm is being held by string as shown in the figure. The tension in the string will be
View SolutionA uniform solid sphere of mass 1kg and radius 10 cm is kept stationary on a rough inclined plane by fixing a highly dense particle at B. Incination of plane is 37∘ with horizontal and AB is the diameter of the sphere which is parallel to the plane, as show in figure. Calculate
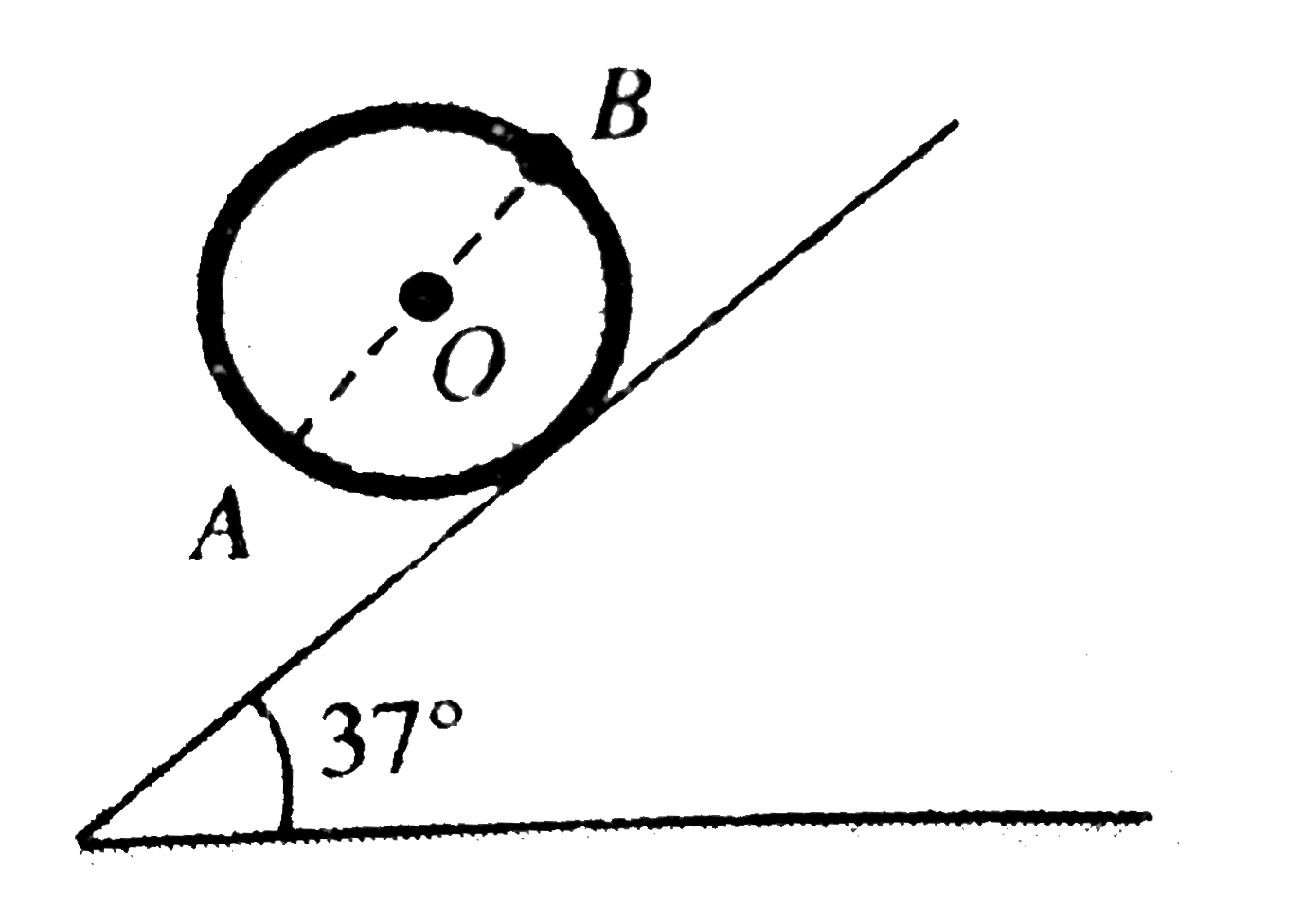
a. mass of the particle fixed at B
b. minimum required coefficient of friction between sphere and plane to keep sphere in equilibrium.
View Solution
Knowledge Check
A sphere of weight W=100N is kept stationary on a rough inclined plane by a horizontal string AB as shown in figure. Then :
 Atension in th string is 100 NBnormal reaction on the sphere by the plane is 100 NCtension in the string is 1002+√3NDfore of friction on the sphere I 1002+√3N
Atension in th string is 100 NBnormal reaction on the sphere by the plane is 100 NCtension in the string is 1002+√3NDfore of friction on the sphere I 1002+√3NAs shown in figure the tension in the horizontal cord is 30N. The weight W and tension in the string OA in Newton are
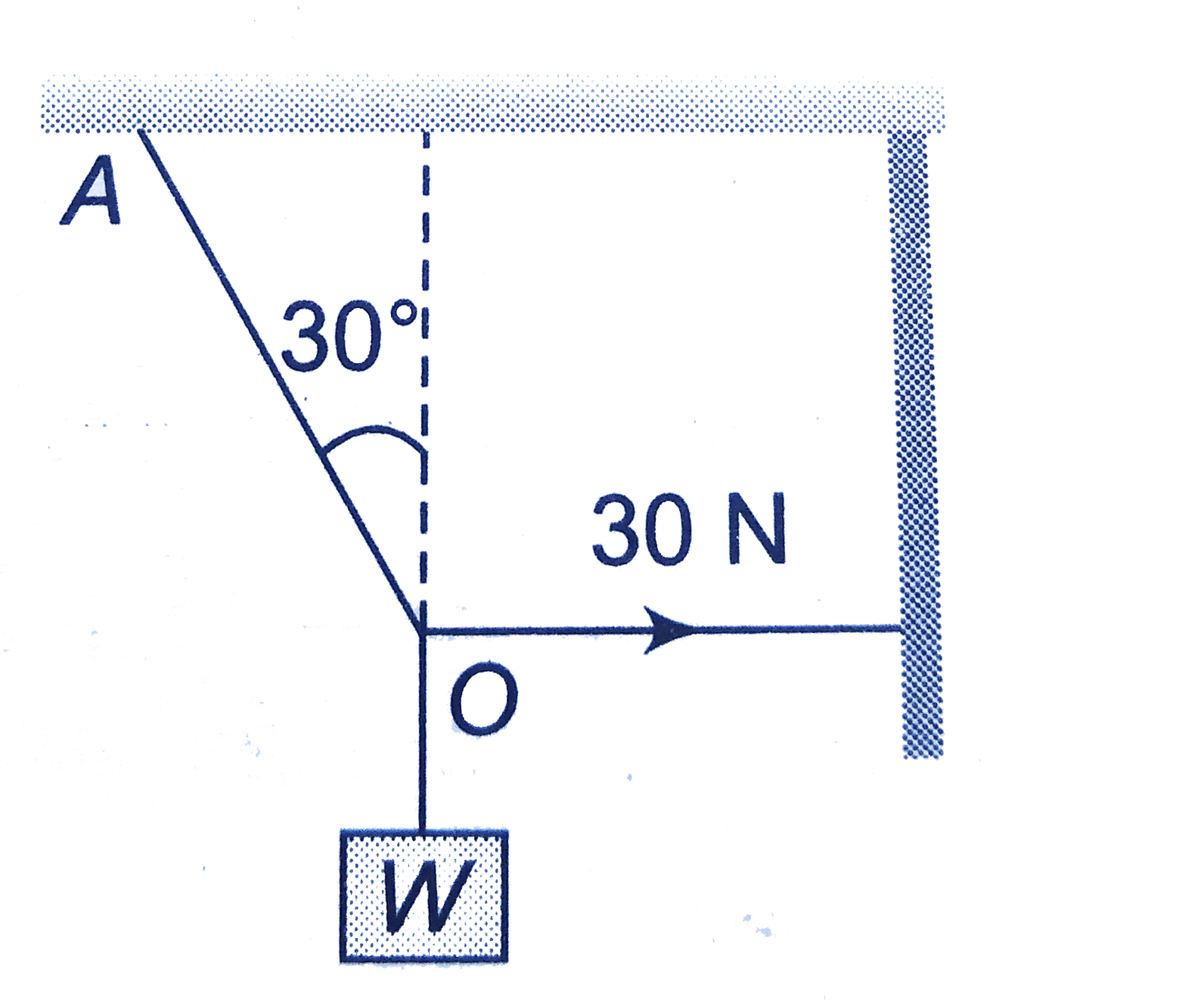 A30√3,30B30√3,60C60√3,30DNone of these
A30√3,30B30√3,60C60√3,30DNone of theseA weight W can be just supported on a rough inclined place by a force F either acting along the plane or horizontally. If θ is the angle of friction, then F/W is
AtanθBsecθCsinθDcosθ
A bob of weight 3N is in equilibrium under the action of two string 1 and 2 (figure) . Find the tension forces in the string.
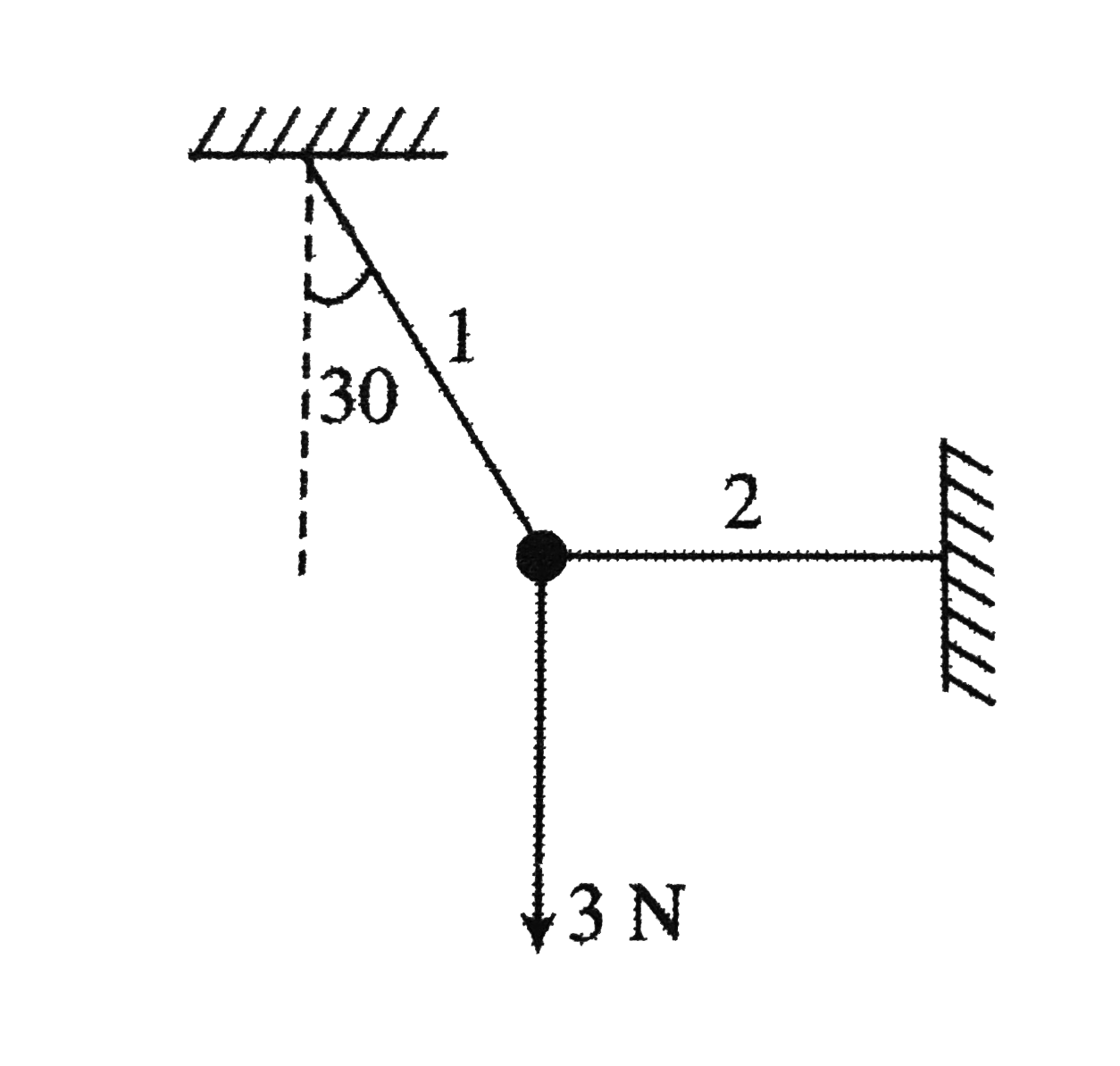
View SolutionA sphere of weight w is in equilibrium on a smooth plane of inclination α to the horizontal , being supported by a string which is in length equal to the radius and is fastened to two points , one on the sphere and the other on the plane . Show that the tension of the string is 23.√3wsinα
View Solution
A sphere kept on a rough inclined plane is in equilibrium by a string wrapped over it. If angle of inclination is θ, the tension in string will be equal to
View SolutionA uniform sphere of weight W and radius 3 m is being held by a frictionless wall as shown in the figure. The tension in the string will be:
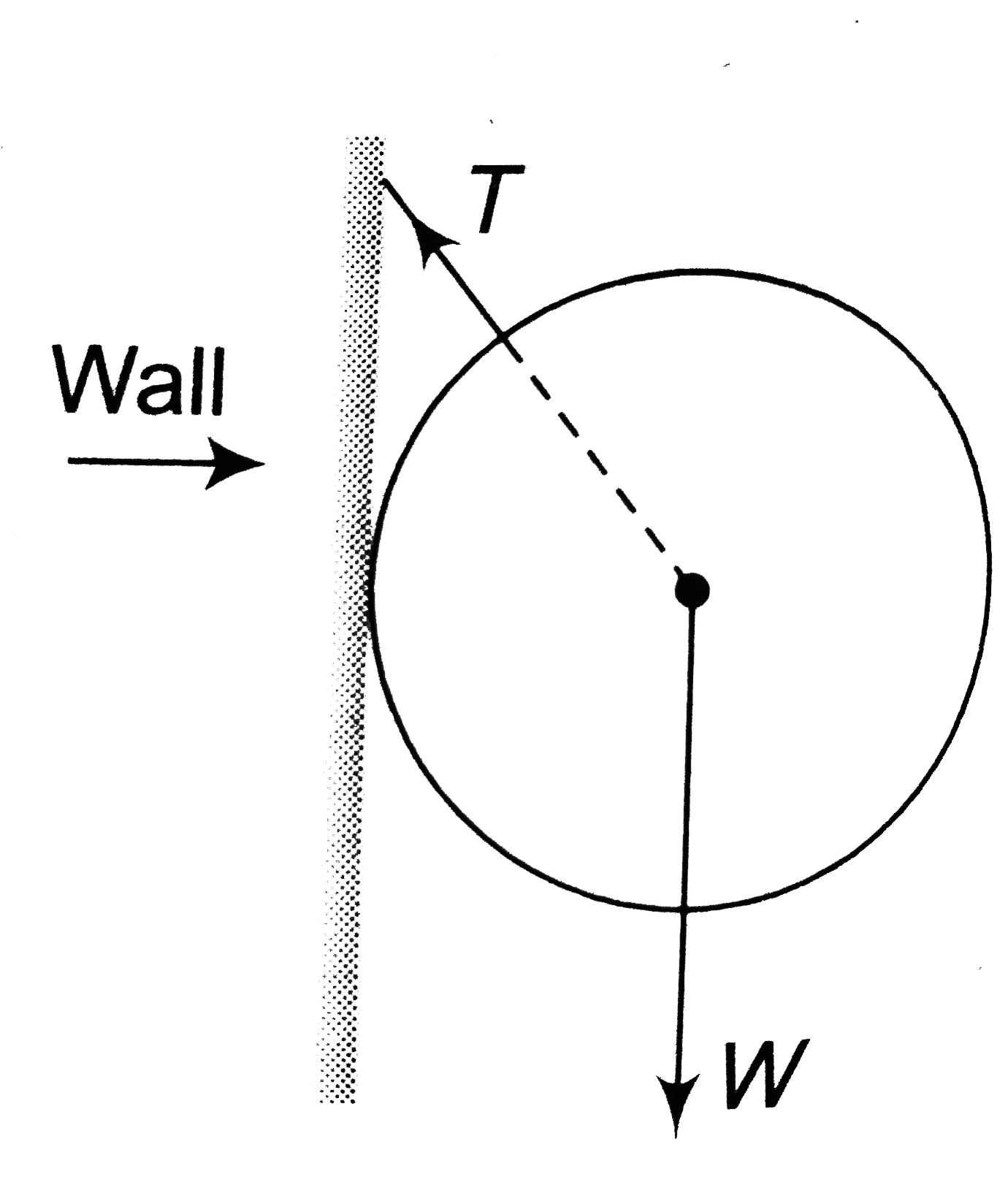
View SolutionFind the tension in the string AB loaded with weight W at the middlw, when AB is horizontal:

View Solution
