A sphere strikes a wall and rebounds with coefficient of restitution 13. If it rebounds with a velocity of 0.1m/sec at an angle of 60∘ to the normal to the wall, the loss of kinetic energy is :
50%
3313%
40%
6623%
The correct Answer is:D
To solve the problem of finding the loss of kinetic energy when a sphere strikes a wall and rebounds, we can follow these steps:
Step 1: Understand the Given Data
- Coefficient of restitution e=13
- Final velocity V=0.1m/s
- Angle of rebound θ=60∘
Step 2: Break Down the Final Velocity into Components
The final velocity can be broken down into its horizontal and vertical components:
- Horizontal component: Vx=Vcos(60∘)=0.1cos(60∘)=0.1×12=0.05m/s
- Vertical component: Vy=Vsin(60∘)=0.1sin(60∘)=0.1×√32=0.05√3m/s
Step 3: Use the Coefficient of Restitution
The coefficient of restitution relates the velocities before and after the collision:
e=VxUx
Where Ux is the initial horizontal component of the velocity before the collision. Rearranging gives:
Ux=Vxe=0.0513=0.15m/s
Step 4: Determine the Initial Vertical Component
Since the vertical component does not change during the collision:
Uy=Vy=0.05√3m/s
Step 5: Calculate the Initial Velocity
Now, we can find the magnitude of the initial velocity U:
U=√U2x+U2y=√(0.15)2+(0.05√3)2
Calculating this:
U=√0.0225+0.0075=√0.03=0.1732m/s
Step 6: Calculate the Initial and Final Kinetic Energies
The kinetic energy is given by:
KE=12mv2
- Initial kinetic energy KEi=12mU2=12m(0.1732)2
- Final kinetic energy KEf=12mV2=12m(0.1)2
Step 7: Find the Loss of Kinetic Energy
The loss of kinetic energy ΔKE is given by:
ΔKE=KEi−KEf=12m(0.17322−0.12)
Calculating the values:
ΔKE=12m(0.03−0.01)=12m(0.02)=0.01m
Conclusion
The loss of kinetic energy is 0.01m joules, where m is the mass of the sphere.
- Coefficient of restitution e=13
- Final velocity V=0.1m/s
- Angle of rebound θ=60∘
The final velocity can be broken down into its horizontal and vertical components:
- Horizontal component: Vx=Vcos(60∘)=0.1cos(60∘)=0.1×12=0.05m/s
- Vertical component: Vy=Vsin(60∘)=0.1sin(60∘)=0.1×√32=0.05√3m/s
The coefficient of restitution relates the velocities before and after the collision:
e=VxUx
Where Ux is the initial horizontal component of the velocity before the collision. Rearranging gives:
Ux=Vxe=0.0513=0.15m/s
Since the vertical component does not change during the collision:
Uy=Vy=0.05√3m/s
Now, we can find the magnitude of the initial velocity U:
U=√U2x+U2y=√(0.15)2+(0.05√3)2
Calculating this:
U=√0.0225+0.0075=√0.03=0.1732m/s
The kinetic energy is given by:
KE=12mv2
- Initial kinetic energy KEi=12mU2=12m(0.1732)2
- Final kinetic energy KEf=12mV2=12m(0.1)2
The loss of kinetic energy ΔKE is given by:
ΔKE=KEi−KEf=12m(0.17322−0.12)
Calculating the values:
ΔKE=12m(0.03−0.01)=12m(0.02)=0.01m
The loss of kinetic energy is 0.01m joules, where m is the mass of the sphere.
Topper's Solved these Questions
- BOOK - ANURAG MISHRACHAPTER - IMPULSE AND MOMENTUMEXERCISE - L-221videos
IMPULSE AND MOMENTUM
- BOOK - ANURAG MISHRACHAPTER - IMPULSE AND MOMENTUMEXERCISE - L-3,(P-1)5videos
IMPULSE AND MOMENTUM
- BOOK - ANURAG MISHRACHAPTER - IMPULSE AND MOMENTUMEXERCISE - Example50videos
IMPULSE AND MOMENTUM
- BOOK - ANURAG MISHRACHAPTER - FORCE ANALYSISEXERCISE - Matching type13videos
FORCE ANALYSIS
- BOOK - ANURAG MISHRACHAPTER - RIGID BODY MOTIONEXERCISE - MATCH THE COLUMN12videos
RIGID BODY MOTION
Similar Questions
A smooth sphere of mass m is moving on a horizontal plane with a velocity 3ˆi+ˆj when it collides with a vertical wall which is parallel to the ˆj vector. If the coefficient of restitution between the sphere and the wall is 1/2. find
a. the velocity of the sphere after impact,
b. the loss in kinetic energy caused by the impact.
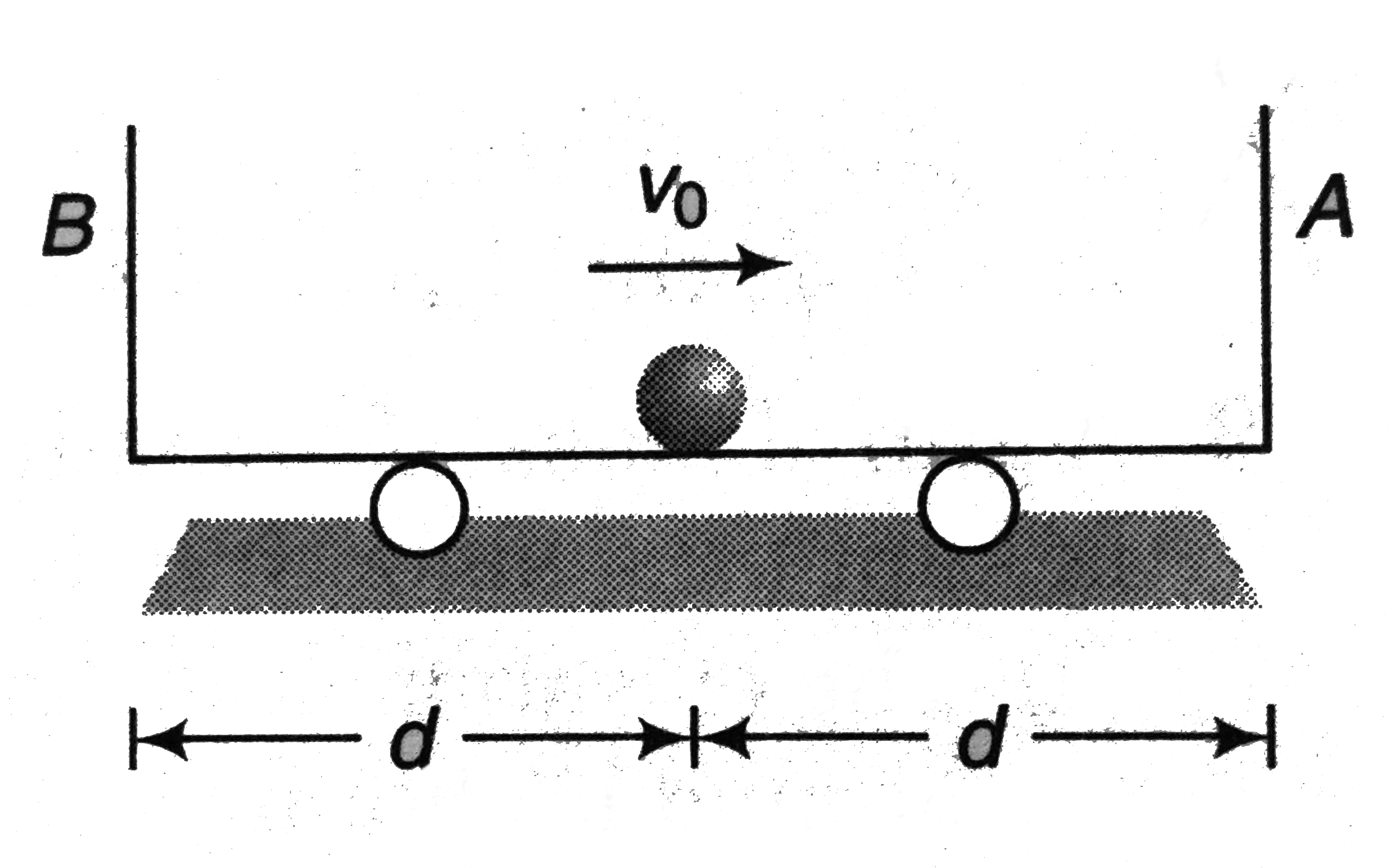
c. the impulse that acts on the sphere.View SolutionA ball of negligible size and mass m is given a velocity v0 on the centre of the cart which has a mass M and is originally at rest. If the coefficient of restitution between the ball and walls A and B is e. Determine the velocity of the ball and the cart just after the ball strikes A. Also, determine the total time needed for the ball to strike A, rebound, then strike B, and rebound and then return to the centre of the cart. Neglect friction.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A body falling with a speed of 2m/s strikes the floor and rebounds with a speed of 1 m/s. The loss of kinetic energy is
A12.5%B50%C25%D75% - Question 2 - Select One
A body falls on a surface of coefficient of restitution 0.6 from a height of 1 m . Then the body rebounds to a height of
A0.6 mB0.4 mC1 mD0.36 m - Question 3 - Select One
A ball of mass 500 gram strikes a wall with a velocity of 80 m/s and rebounds with the same velocity. If the tme of contact is 1/30 sec, then the force exerted by the ball on the wall is
A2000NB2200 NC2400ND2500N
A ball of mass 0.1 kg strikes a wall normally with a speed of 30ms−1 and rebounds with a speed of 20ms−1. The impulse of the force exerted by the wall on the ball is
View SolutionA ball strikes a wall with a velocity →u at an angle θ with the normal to the wall surface. and rebounds from it at an angle β with the surface. Then
View SolutionA particle strikes a smooth horizontal surface at an angle of 45∘ with a velocity of 100m/s and rebounds. If the coefficient of restitution between the floor and the particle is 0.57 then the angle which the velocity of the particle after it rebounds will make with the floor is
View SolutionA cricket ball of mass 0.5 kg strikes a circket bat normally with a velocity of 20 m s−1 and rebounds with a velocity of 10 m s−1. The impulse of the force exerted by the ball on the bat is
View SolutionA stone is projected from the ground with velocity 50ms at an angle of 30∘. It crosses a wall after 3 sec. How far beyond the wall the stone will strike the ground (g=10msec2?
View Solution

