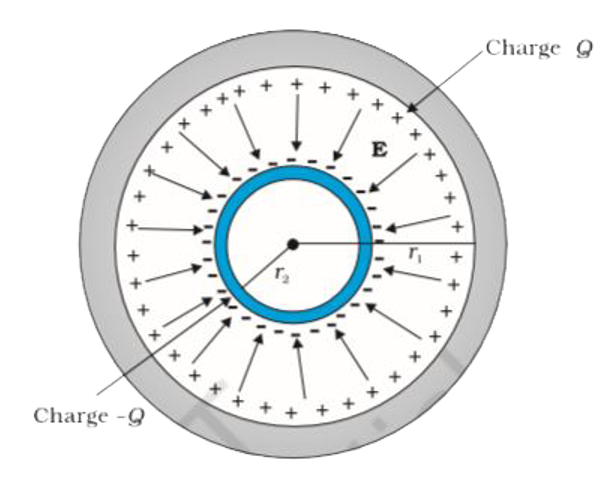
A spherical capacitor consists of two concentric spherical conductors, held in position by suitable insulating supports (Fig. 2.34). Show that the capacitance of a spherical capacitor is given by
C=4πε0r1r0r1−r2
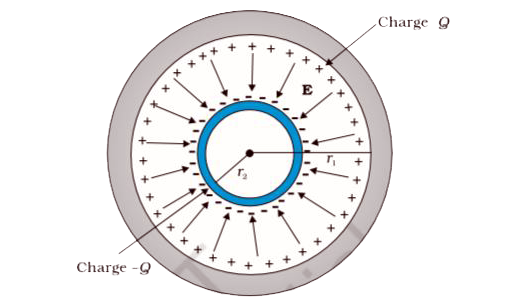
where r1 and r2 are the radii of outer and inner spheres, respectively. Solution in Gujrati
By two concentric sphedcal conductors a spherical shell is formed.
Radius of the inner shell is r1 and radius of outer shell is r2 .
The outer surface of the inner shell has induced charge - Q and the inner surface of the outer shell has charge +Q .
A spherical Gaussian surface of radius r from common point centre O .
r<r1 means inner shell E =0 and r>r2 means outside inner shell E =0 .
Due to charges on inner shell a space between two shells have radial electric field.
According to Gauss.s law, electric flux at P at a common centre from distance r,
EScos0∘=Q∈0
∴ES=Q∈0
∴E×4πr2=Q∈0 [ Area of sphere = 4πr2]
∴E=Q4π∈0r2
p.d. between two shells
V=∫r2r1→E.d→r=−∫r2r1Edr cos 180∘
=∫r2r1Edr=∫r2r1Q4π∈0r2dr
=Q4π∈0∫r2r11r2dr
=Q4π∈0[−1r]r2r1=Q4π∈0[1r1−1r2]
Capacitance of spherical capacitor
C=QV
∴CQQ4π∈0[1r1−1r2]=4π∈0r1r2r2−r1
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCEBOOK - KUMAR PRAKASHANCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCEEXERCISE - Section B ( Numerical From DARPAN. Based on Textbook )
19 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCEBOOK - KUMAR PRAKASHANCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCEEXERCISE - Section B (questions)
2 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCEBOOK - KUMAR PRAKASHANCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCEEXERCISE - Section B Numericals
16 Videos
- ELECTROMAGNETIC WAVESBOOK - KUMAR PRAKASHANCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - BOARD.S QUESTION PAPER MARCH - 2020 (PART - B) SECTION - C
5 Videos
- MAGNETISM AND MATTERBOOK - KUMAR PRAKASHANCHAPTER - MAGNETISM AND MATTEREXERCISE - SECTION D Multiple Choice Questions (MCQs) (MCQs asked in Competitive Exams) MCQs asked in Board Exam and GUJCET
7 Videos
Similar Questions
An electric field is represented by →E=Axˆi where A = 10Vm2 . Find the potential of the origin with respect to the point (10,20) m.
View SolutionA slab of material of dielectric constant K has the same area as the plates of a parallel plate capacitor but has a thickless (12)d. Where d is the separation of the plates . How is the capacitance changed when the slab is inserted between the plates
View SolutionCalculate the potential at a point P due to a charge of 4×10−7 C located 9 cm away.
View SolutionDetermine the current in each branch of the given network.

View SolutionAt a place on earth the vertical component of earth's magnetic field is √3 times its horizontal component. The angle of dip at this place is …...
View SolutionTwo spherical nuclei have mass number 216 and 64 with their radii R_(1) and R_(2) respectively. The ratio, (R_(1))/(R_(2)) is equal to
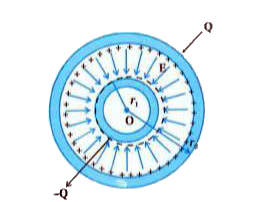
View SolutionA sperical capacitor consists of two concentric spherical conductors, held in position by suitable insulating supports. Show that the capacitance of this spherical capacitor is given by C = (4pi in_(0) r_(1) r_(2))/(r_(1) - r_(2)) , Where r_(1) and r_(2) are radill of outer and inner spheres respectively.
View SolutionConsider two concentric spherical metal shells of radii r_(1) and r_(2) (r_(2) gt r_(1)) . Find the charge on the inner shell and charge distribution.
View SolutionConsider two concentric spherical metal shells of radii r1 and r2(r2>r1). If the outer shell has a charge q and the inner one is grounded, then the charge on the inner shell is
View SolutionThe capacitance of two concentric spherical shells of radii R_(1) and R_(2) (R_(2) gt R_(1)) is
View Solutionदो संकेंद्री गोलीय चालकों जिनको उपयुक्त विद्युतरोधी आलंबों से उनकी स्थिति में रोका गया है, से मिलकर एक गोलीय संधारित्र बना है। दर्शाइए कि गोलीय संधारित्र कि धारिता C इस प्रकार व्यक्त कि जाती है :
C=4πε0r1r2r1−r2
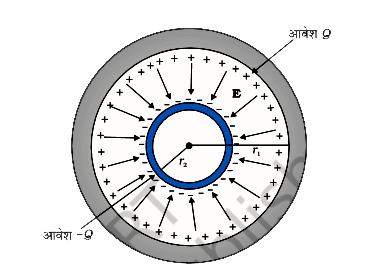
यहाँ r1 और r2 क्रमश: बाहरी तथा भीतरी गोलों कि त्रिज्याएँ हैं ।
View SolutionA spherical capacitor consists of two concentric spherical conductors, held in position by suitable insulating supports (Fig). Show that the capacitance of a spherical capacitor is given by C=4πε0r1r2r1−r2
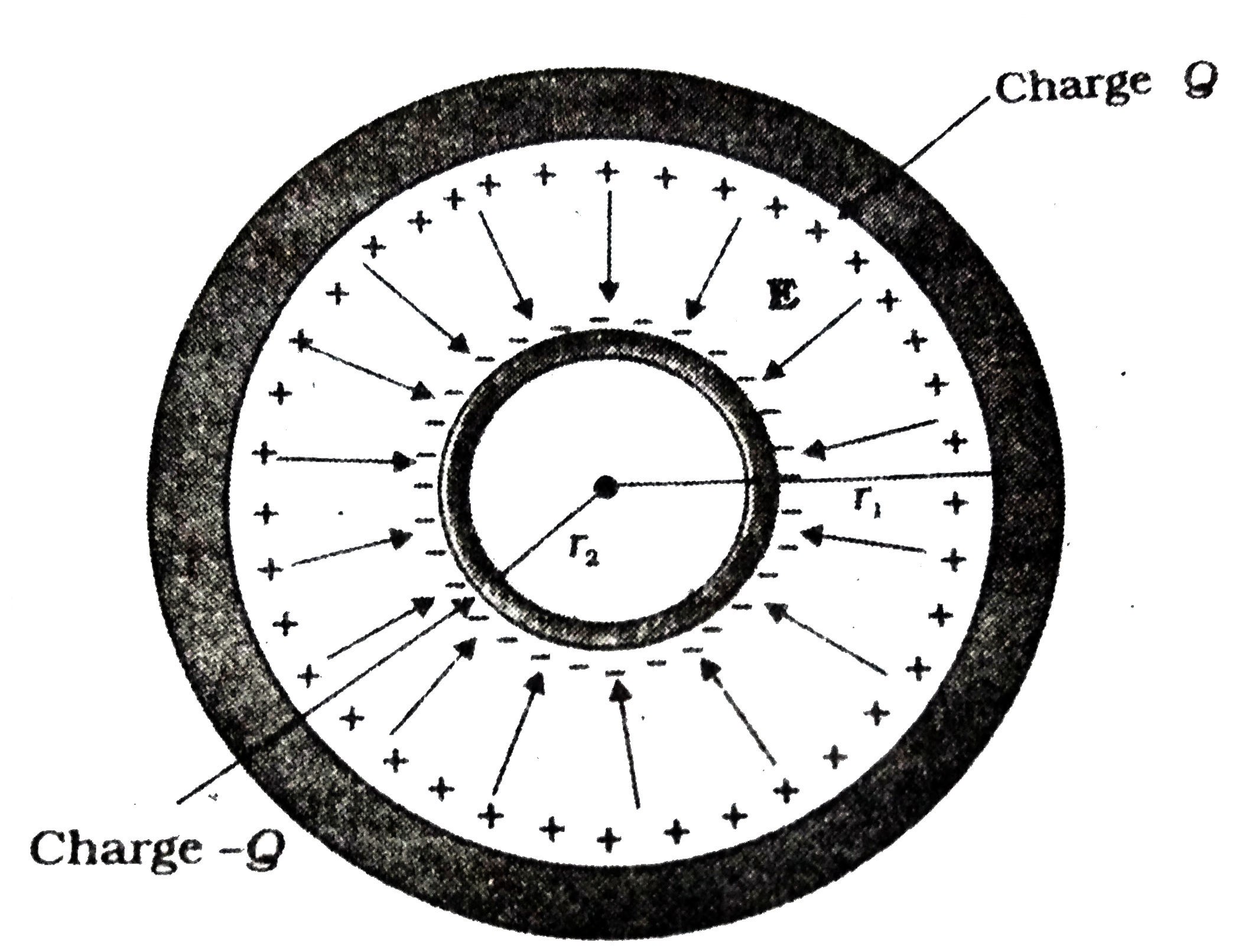
where r1andr2 are the radii of outer and inner spheres , respectively.
View Solutionदो संकेंद्री गोलीय चालकों जिनको उपयुक्त विद्युतरोधी आलंबों से उनकी स्थिति में रोका गया है, से मिलकर एक गोलीय संधारित्र बना है ( चित्र) दर्शाइए कि गोलीय संधारित्र की धारिता C इस प्रकार व्यक्त की जाती है। C=4πε0r1r2r1−r2

यहाँ r1और r2, क्रमशः बाहरी तथा भीतरी गोलों की त्रिज्याएँ
View SolutionTwo charged spherical conductors of radii R_(1) and R_(2) are connected by a wire. Then the ratio of surface charge densitites of the spheres sigma_(1)//sigma_(2) is
View SolutionA spherical conductor consists of two concentric conductive shells that are held steady with proper antitrust bases. Prove that the capacitance of the spherical capacitor C=(4piepsilon_(0)r_(1)r_(2))/(r_(1)-r_(2)) Where r_(1) And r_(2) , Are the diameters of the outer and inner spheres, respectively [Fig. 10].
View SolutionA spherical capacitor consists of two concentric spherical conductors, held in position by suitable insulating supports (Fig. 2.34). Show that the capacitance of a spherical capacitor is given by
C=4πε0r1r0r1−r2
where r1 and r2 are the radii of outer and inner spheres, respectively.
View Solution
