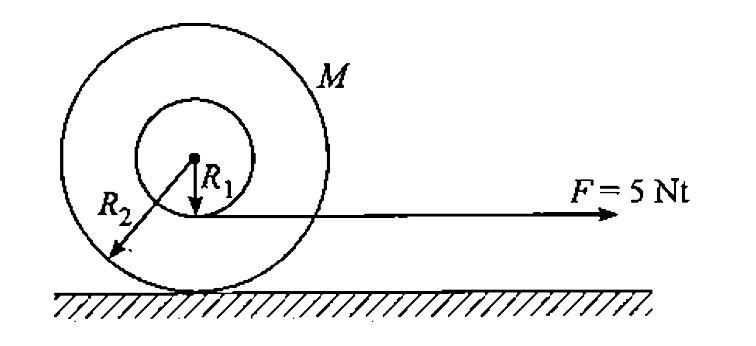
A spool has a mass of 2 kg,an inner radius =3cm,and an outer radius .A constant horizontal force of 5N is applied to the free end of a massless thread that is wrapped around the inner cylinder of the spool as shownin figure-5.150. If the spool rolls without slipping, calculate the linear acceleration along the horizontal surface. What is the minimum coefficient of static friction required to prevent slipping ?
The correct Answer is:
Topper's Solved these Questions
- RIGID BODIES AND ROTATIONAL MOTIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - RIGID BODIES AND ROTATIONAL MOTIONEXERCISE - MCQ
16 Videos
- NEWTONS LAWS OF MOTIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Unsolved Numerical Problem
81 Videos
- WORK, ENERGY AND POWERBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - WORK, ENERGY AND POWEREXERCISE - unsolved
1 Videos
Similar Questions
The radius of a sphere is 5 cm. Calculate the radius of gyration about any tangent.
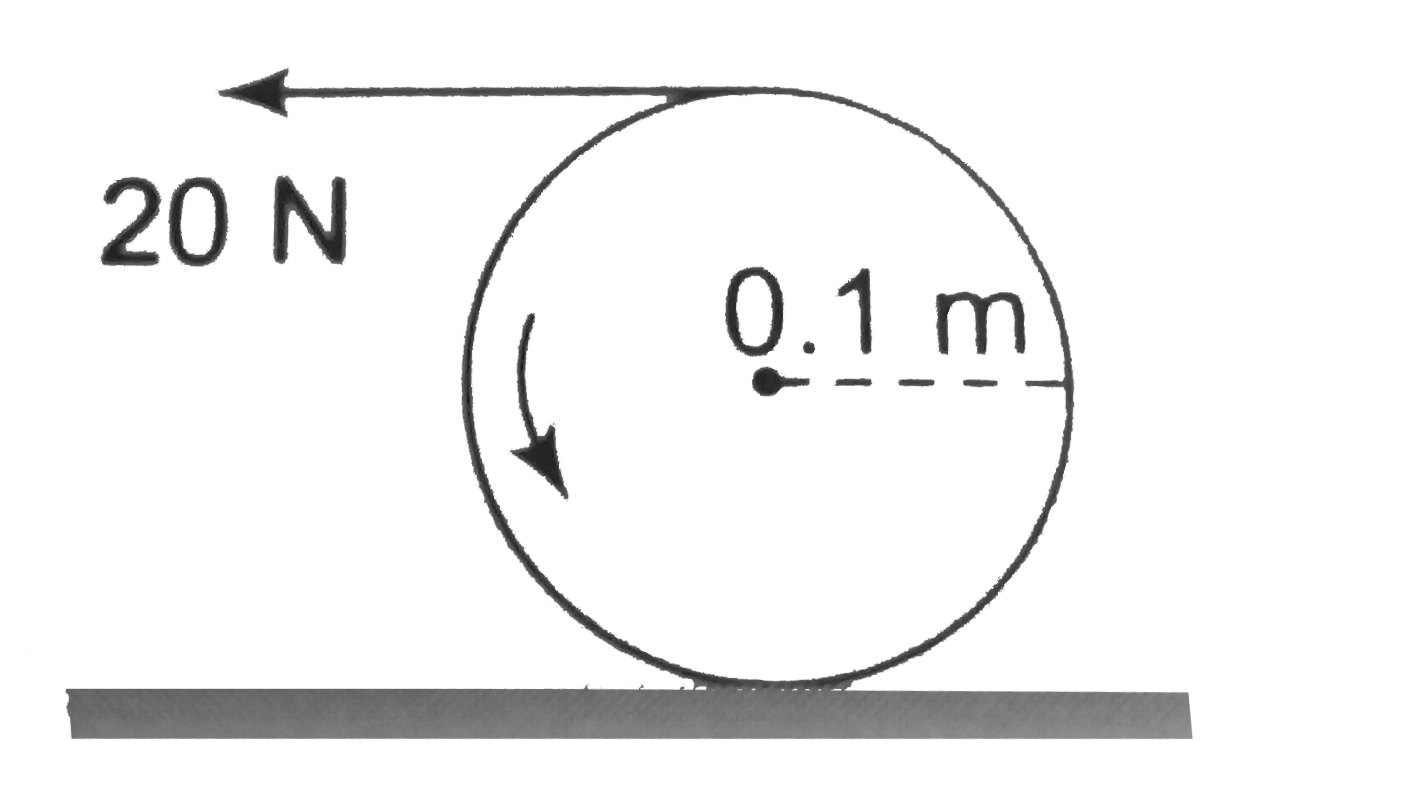
View SolutionThe rope shown in figure is wound around a cylinder of mass 4kg and moment of inertia 0.02kgm2 about the cylinder axis. If the cylinder rolls without slipping, then the linear acceleration of its centre of mass is.
 .
.
View Solution
Knowledge Check
The rope shown in figure is wound around a cylinder of mass 4kg and moment of inertia 0.02kgm2 about the cylinder axis. If the cylinder rolls without slipping, then the linear acceleration of its centre of mass is.
 .A6.7m/s2B10.0m/s2C3.3m/s2Dnone of these
.A6.7m/s2B10.0m/s2C3.3m/s2Dnone of theseThe radius of gyration of a body about an axis at a distance of 4cm from its centre of mass is 5cm. The radius of gyration about a parallel axis through centre of mass is
A2cmB5cmC4cmD3cmInner and outer radii of a spool are r and R respectively. A thread is wound over its inner surface and placed over a rough horizontal surface. Then:
 .AThread unwinds, spool rotates anticlockwise and friction acts leftwardsBThread winds, spool rotates clockwise and friction acts leftwardsCThread winds, spool moves to the right and friction act rightwardsDThread winds, spool moves to the right and friction does not come into existence
.AThread unwinds, spool rotates anticlockwise and friction acts leftwardsBThread winds, spool rotates clockwise and friction acts leftwardsCThread winds, spool moves to the right and friction act rightwardsDThread winds, spool moves to the right and friction does not come into existence
A cylinder of mass m and radius R rolls down an inclined plane of inclination θ. Calculate the linear acceleration of the axis of cylinder.
View SolutionA string is wrapped around a cylinder of radius R and pulled at the top most point horizontally by a force F The cylinder rolls without slipping on the horizontal surface. The work done by the force by the time the cylinder makes one full rotation is
View Solution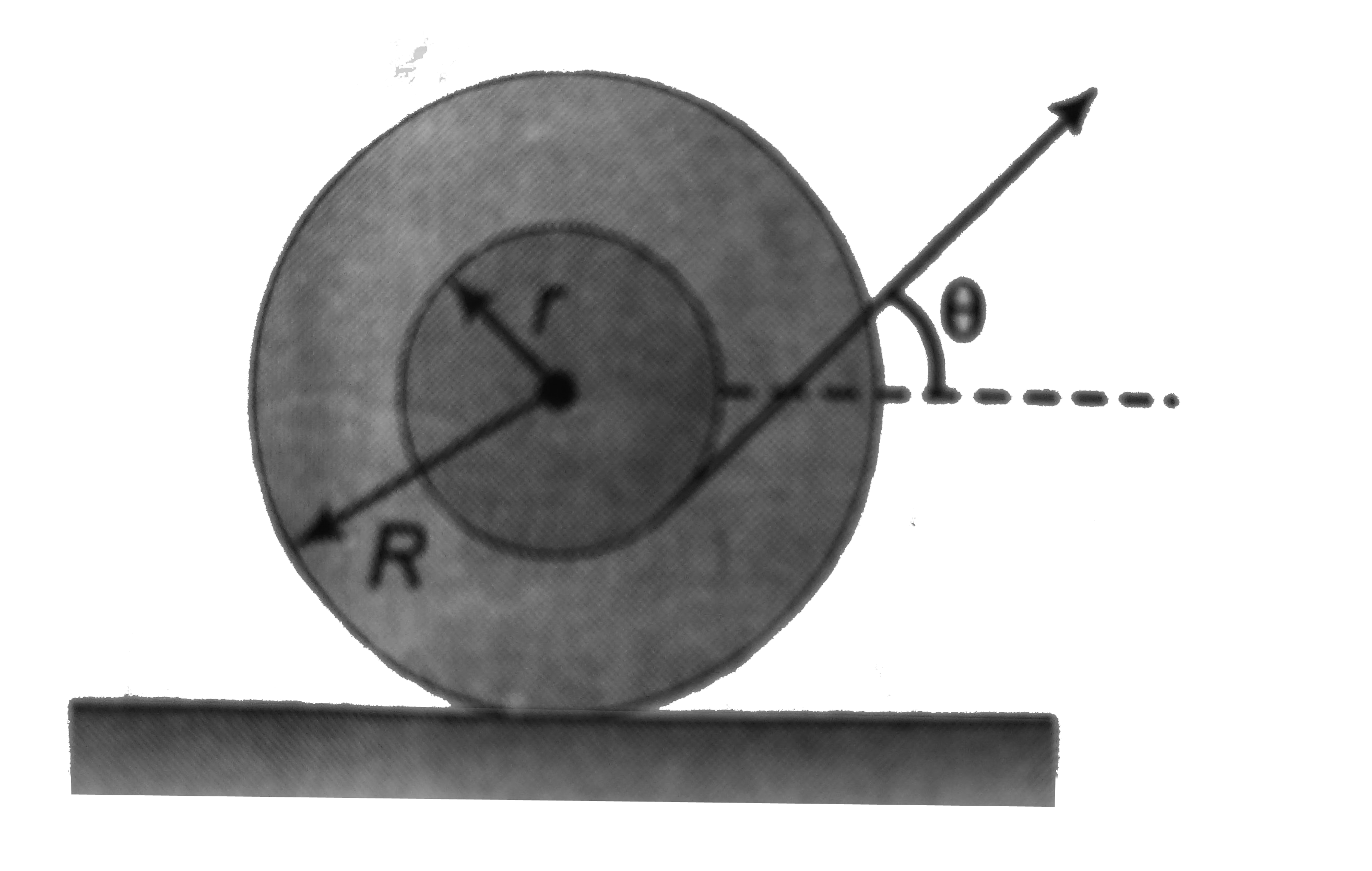
A spool is pulled at an angle θ with the horizontal on a rough horizontal surface as shown in the figure. If the spool remains at rest, the angle θ is equal to
View Solution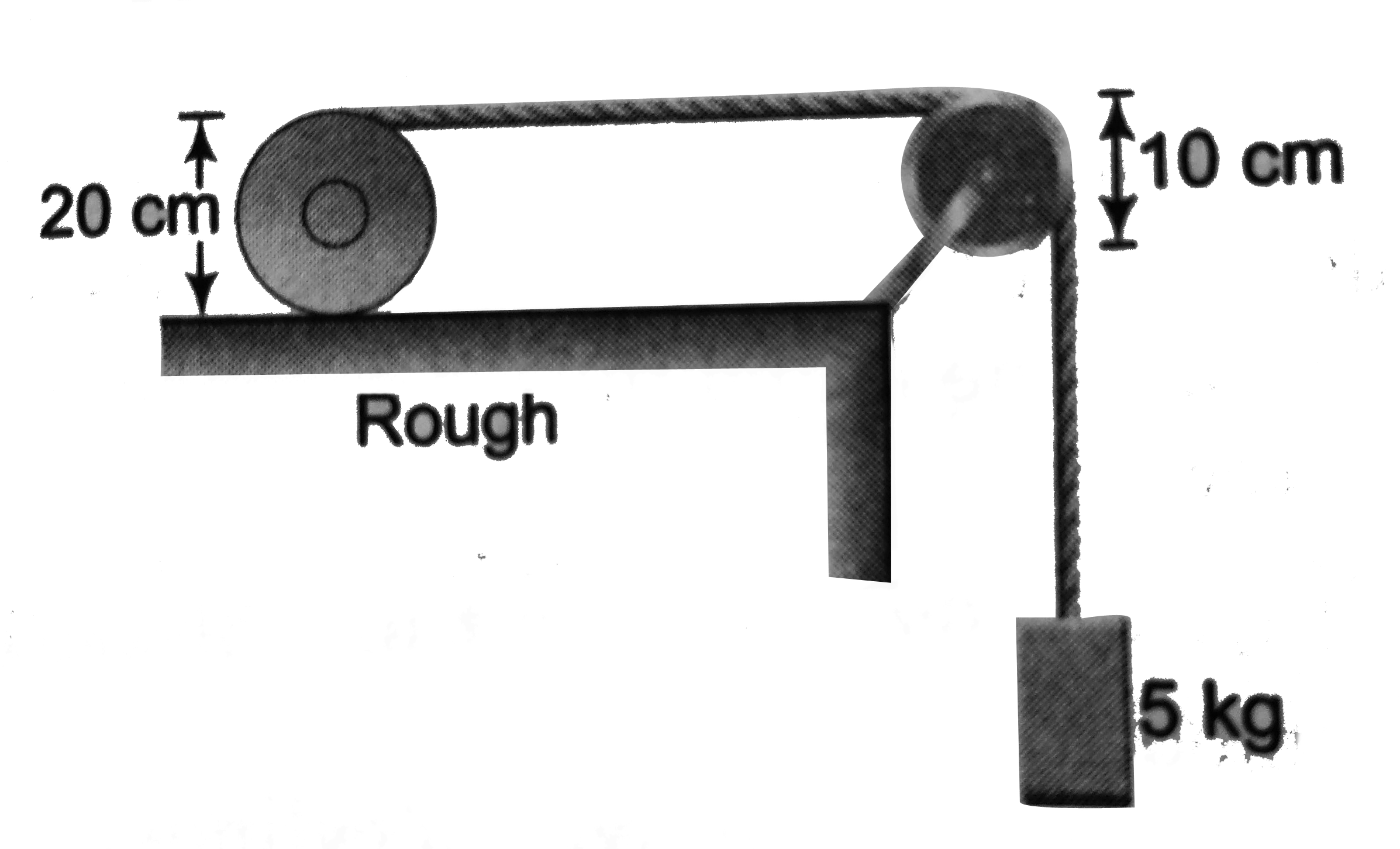
in figure the cylinder of mass 10kg and radius 10 cm has a tape wrapped round it. The pully weighs 100 N and has a radius 5 cm. When the system is released the 5 kg mass comes down and the cylinder rolls without slipping. Calculate the acceleration and velocity of the mass as a function of time.
View Solution
A spool is pulled at an angle θ with the horizontal on a rough horizontal surface as shown in the figure. If the spool remains at rest, the angle θ is equal to
View Solution
