A spring is elongated by 2 cm due to a 80 g mass attached to it. Another body of mass 600g is attached to the end of the spring and it is displaced by 8 cm from its equilibrium position. Calculate the energy of the system in this position. Considering the principle of conservation of energy, determine the velocity of the body when it is at a distance of 4 cm.
Force constant of the spring,
k=80×9802=40×980dyn⋅cm−1
Mass, m = 600 g , amplitude, A = 8 cm.
Total energy, E = maximum potential energy = potential energy at the ends of the path of motion = 12kA2=12×40×980×(8)2=1254400erg=0.12544J.
Even for x = 4 cm, the total energy remains unchanged. If v is the velocity at this position, then
12mv2+12kx2=12×40×980×64
or, 12mv2=12×40×980×64−12×40×980×42
= 12×40×980×(64−16)
= 12×40×980×48
or, v2=40×980×48m=40×980×48600=4×49×16
or, v=√4×49×16=2×7×4=56cm⋅s−1.
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - CHHAYA PUBLICATIONCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - SECTION RELATED QUESTIONS
8 Videos
- SIMPLE HARMONIC MOTIONBOOK - CHHAYA PUBLICATIONCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - HIGHER ORDER THINKING SKILL (HOTS) QUESTIONS
32 Videos
- ROTATION OF RIGID BODIES BOOK - CHHAYA PUBLICATIONCHAPTER - ROTATION OF RIGID BODIES EXERCISE - CBSE SCANNER
10 Videos
- STATICSBOOK - CHHAYA PUBLICATIONCHAPTER - STATICSEXERCISE - CBSE SCANNER
3 Videos
Similar Questions
A cart of mass 2.00kg is attached to the end of a horizontal spring with force constant k=150N/m. The cart is displaced 15.0cm from its equilibrium position and released. What are
(a) the amplitude (b) the period ( c) the mechanical energy (e) the maximum velocity of the cart ? Neglect friction.
View SolutionA cart of mass 2.00kg is attached to the end of a horizontal spring with force constant k=150N/m. The cart is displaced 15.0cm from its equilibrium position and released. What are
(a) the amplitude (b) the period ( c) the mechanical energy (e) the maximum velocity of the cart ? Neglect friction.
View Solution
Knowledge Check
For a body of mass m attached to the spring the spring factor is given by
Am/ω2Bmω2Cm2ωDm2ω2One end of an unstretched vertical spring is attached to the ceiling and an object attached to the other end is slowly lowered to its equilibrium position. If S is the gain in spring energy and G is the loss in gravitational potential energy in the process, then
A(a) S=GB(b) S=2GC(c) G=2SD(d) None of the aboveOne end of an unstretched vertical spring is attached to the ceiling and an object attached to the other end is slowly lowered to its eqilibrium position. If S be gain in spring energy and G be loss in gravitational potential energy in the process, then
AS=GBS=2GCG=2SDNone of these
One end of an unstretched vertical spring is attached to the ceiling and an object attached to the other end is slowly lowered to its equilibrium position. If S is the gain in spring energy and G is the loss in gravitational potential energy in the process, then
View SolutionTwo identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in figure. When the mass is displaced from equilibrium position by a distance x towards right, find the restoring force.
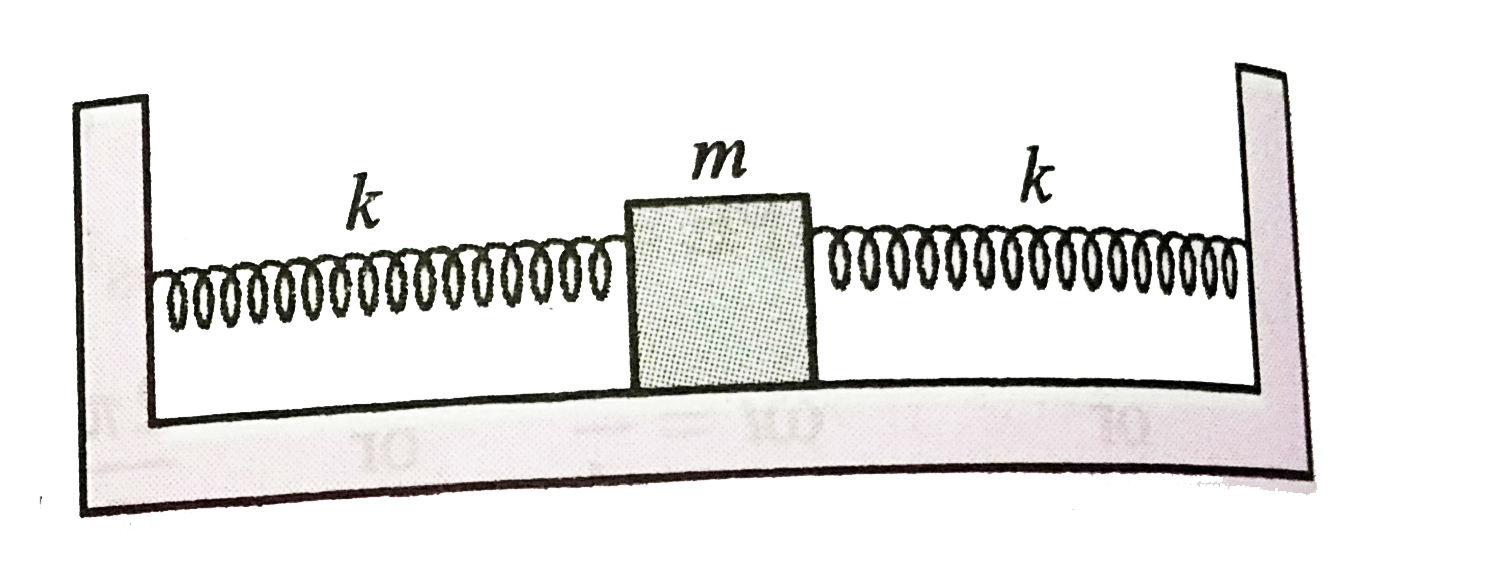
View SolutionTow identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in figure. When the mass is displaced from equilibrum position by a distance x towards right, find the restoring force.

View SolutionTow identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in figure. When the mass is displaced from equilibrum position by a distance x towards right, find the restoring force.

View SolutionA 10 kg metal block is attached to a spring constant 1000Nm−1. A block is displaced from equilibrium position by 10 cm and released. The maximum acceleration of the block is
View Solution
