A standing wave is maintained in a homogerneous string of cross - sectional area a and density p . It is formed bt yhe superpostions given of two waves travelling in opposite direations given by the equations

$\frac{3 π s p ω^{2} a^{2}}{2 k}$

$\frac{π s p ω^{2} a^{2}}{2 k}$

$\frac{5 π s p ω^{2} a^{2}}{2 k}$

$\frac{2 π s p ω^{2} a^{2}}{2 k}$

Text Solution

Verified by Experts

The correct Answer is:C

Class 11 PHYSICS TRAVELLING WAVES

Topper's Solved these Questions

WAVE MOTIONBOOK - DC PANDEYCHAPTER - WAVE MOTIONEXERCISE - More Than One Option is Correct
23 Videos
WAVE MOTIONBOOK - DC PANDEYCHAPTER - WAVE MOTIONEXERCISE - Comprehion Type Questions
19 Videos
WAVE MOTIONBOOK - DC PANDEYCHAPTER - WAVE MOTIONEXERCISE - JEE MAINS
50 Videos
VECTORSBOOK - DC PANDEYCHAPTER - VECTORSEXERCISE - Medical enrances gallery
9 Videos
WORK, ENERGY & POWERBOOK - DC PANDEYCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based
2 Videos

Knowledge Check

A standing wave is maintained in a homogeneous string of corss-sectional area 'S' and density $p h e$ . It is formed by the superposition of two waves travelling in opposite directions given by the equation $y_{1} = a sin (ω t = k x) and y_{2} = 2 a sin (ω t + k x)$ . The total mechanical energy confined between the sections corresponding to the abjacent antinodes is:
A $\frac{3 π s p ω^{2} a^{2}}{2 k}$
B $\frac{π s p ω^{2} a^{2}}{2 k}$
C $\frac{5 π s p ω^{2} a^{2}}{2 k}$
D $\frac{2 π s p ω^{2} a^{2}}{2 k}$
Assertion: Stationary waves are so called because particles are at rest in stationary waves.
Reason: They are formed by the superposition of two identical waves travelling in opposite directions.
AIf both Assertion and Reason are true and the Reason is correct explanation of the Assertion.
BIf both Assertion and Reason are true but Reason is not the correct explanation of Assertion.
CIf Assertion is true, but the Reason is false.
DIf Assertion is false but the Reason is true.
Consider a standing wave formed on a string . It results due to the superposition of two waves travelling in opposite directions . The waves are travelling along the length of the string in the $x$ - direction and displacements of elements on the string are along the $y$ - direction . Individual equations of the two waves can be expressed as
$Y_{1} = 6 (c m) sin [5 (r a d / c m) x - 4 (r a d / s) t]$
$Y_{2} = 6 (c m) sin [5 (r a d / c m) x + 4 (r a d / s) t]$ Here $x$ and $y$ are in $c m$ .
Answer the following questions.
Figure 7.104( c) shows the standing wave pattern at $t = 0$ due to superposition of waves given by $y_{1}$ and $y_{2}$ in Figs.7.104(a) and (b) . In Fig. 7.104 (c ) , $N$ is a node and $A$ and antinode . At this instant say $t = 0$ , instantaneous velocity of points on the string

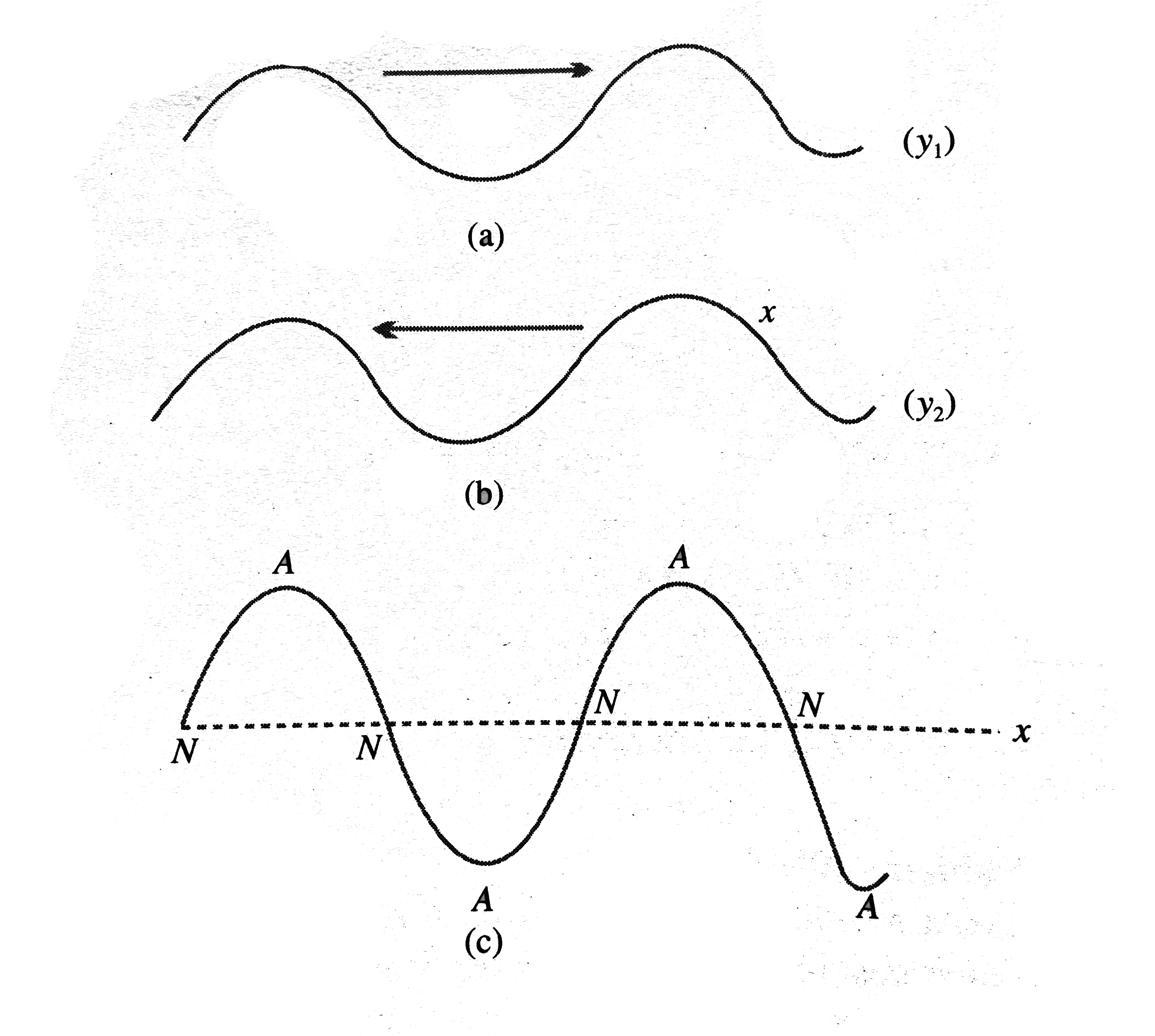Ais different for different points
Bis zero for all points
Cchanges with position of the point
Dis constant but not equal to zero for all points

DC PANDEY-WAVE MOTION-ONLY ONE OPTION IS CORRECT

Home
Ask Doubt
Profile

A standing wave is maintained in a homogerneous string of cross - sectional area a and density p . It is formed bt yhe superpostions given of two waves travelling in opposite direations given by the equations

The correct Answer is:C

Topper's Solved these Questions

Similar Questions

Knowledge Check

Assertion: Stationary waves are so called because particles are at rest in stationary waves. Reason: They are formed by the superposition of two identical waves travelling in opposite directions.

DC PANDEY-WAVE MOTION-ONLY ONE OPTION IS CORRECT

Assertion: Stationary waves are so called because particles are at rest in stationary waves.
Reason: They are formed by the superposition of two identical waves travelling in opposite directions.