A standing wave $y = 2 A sin k x cos ω t$ is set up in the wire $A B$ fixed at both ends by two vertical walls (see Fig. 3.197). The region between the walls contains a constant magnetic field $B$ . Now answer the following question:

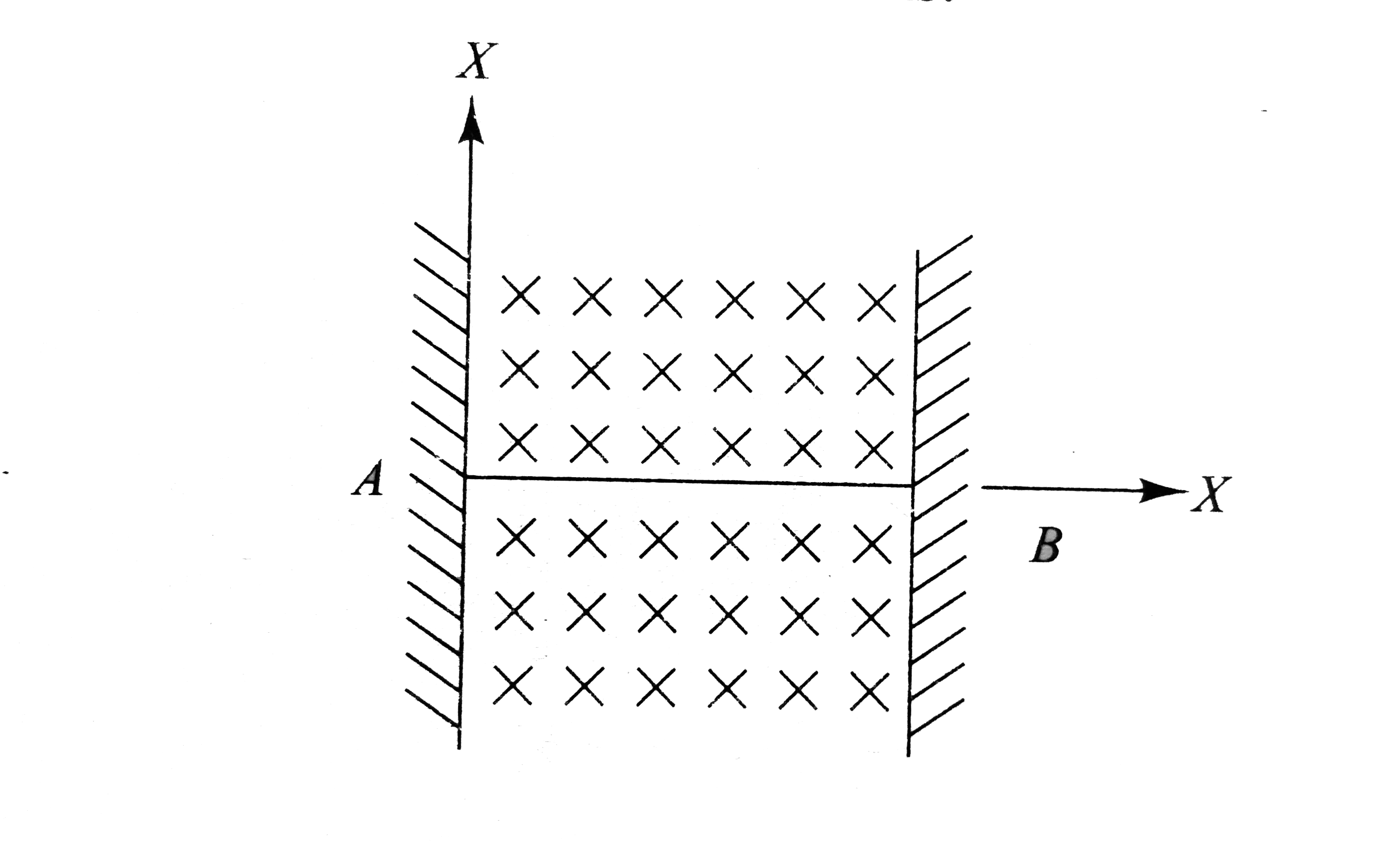
The wire is found to vibrate in the third harmonic. The maximum emf induced is

(a) $\frac{4 (A B) ω}{k}$

(b) $\frac{3 (A B) ω}{k}$

( c) $\frac{2 (A B) ω}{k}$

(d) $\frac{A B ω}{k}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

(a) $y = 2 A sin k x cos ω t$
$v = \frac{d y}{d t} = - 2 A sin k x ω sin ω t$
$v_{max} = - 2 A ω sin k x, k = \frac{3 π}{A B}$
$e = \int_{0}^{l = A B} b v_{max} d x = - 2 a ω B \int_{0}^{A B} sin k x d x = + \frac{2 ω A B}{k} [\frac{cos (3 π)}{A B} A B - cos θ] = \frac{- 4 (A B) ω}{k}$
$ω t = \frac{π}{2}$
$t = \frac{π}{2 ω}$
For second harmonic $k = \frac{2 π}{A B}$

Updated on:21/07/2023

Class 12 PHYSICS ELECTROMAGNETIC INDUCTION

Topper's Solved these Questions

Knowledge Check

Question 1 - Select One
A stsnding wave $y = 2 A sin k x cos ω t$ is set up in the wire $A B$ fixed at both ends by two vertical walls (see Fig. 3.197). The region between the walls contains a constant magnetic field $B$ . Now answer the following question:

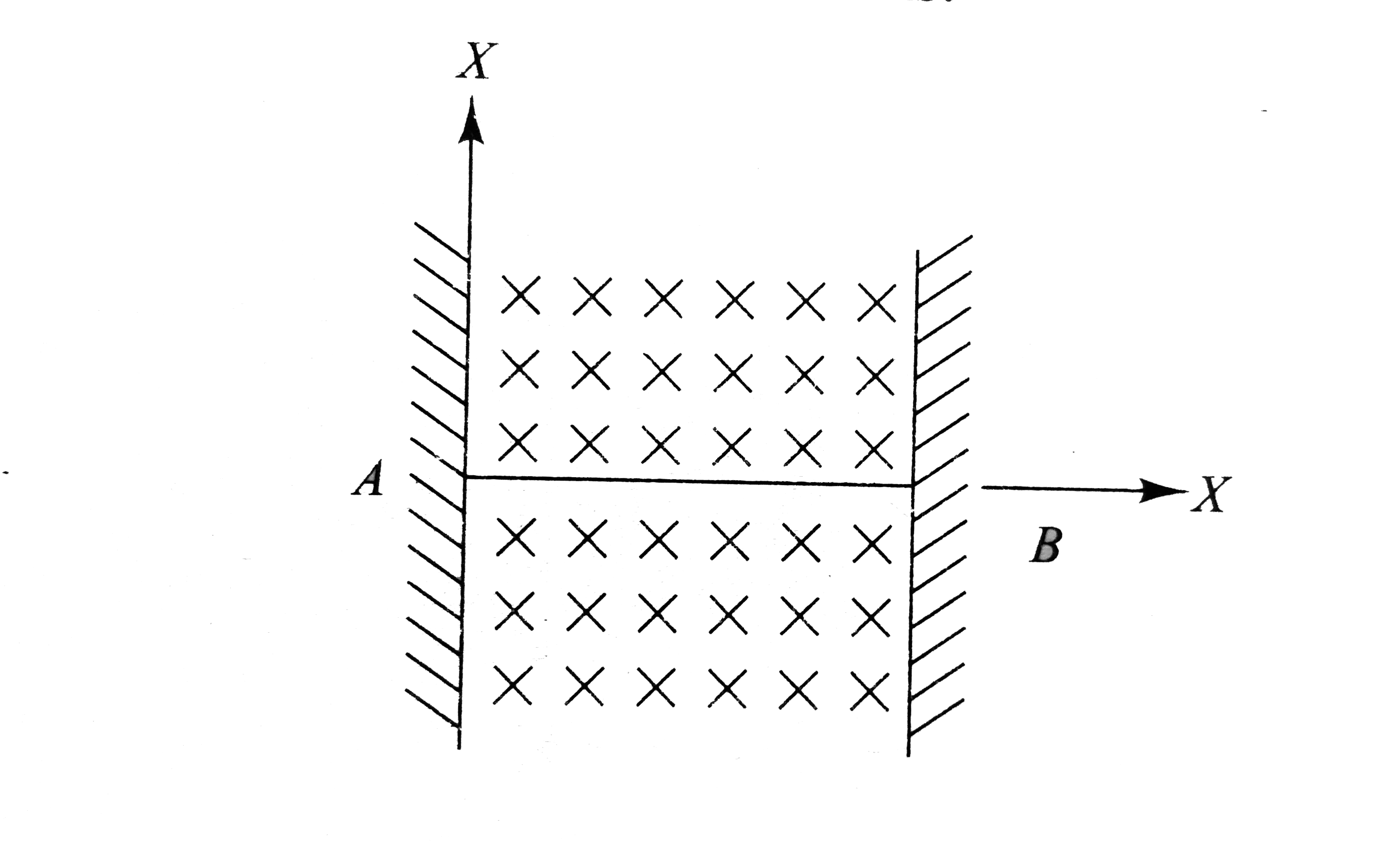
In the above qestion, the time when the emf becomes maximum for the time is
A(a) $\frac{2 π}{ω}$
B(b) $\frac{π}{ω}$
C( c) $\frac{π}{2 ω}$
D(d) $\frac{π}{4 ω}$
Question 2 - Select One
A stsnding wave $y = 2 A sin k x cos ω t$ is set up in the wire $A B$ fixed at both ends by two vertical walls (see Fig. 3.197). The region between the walls contains a constant magnetic field $B$ . Now answer the following question:

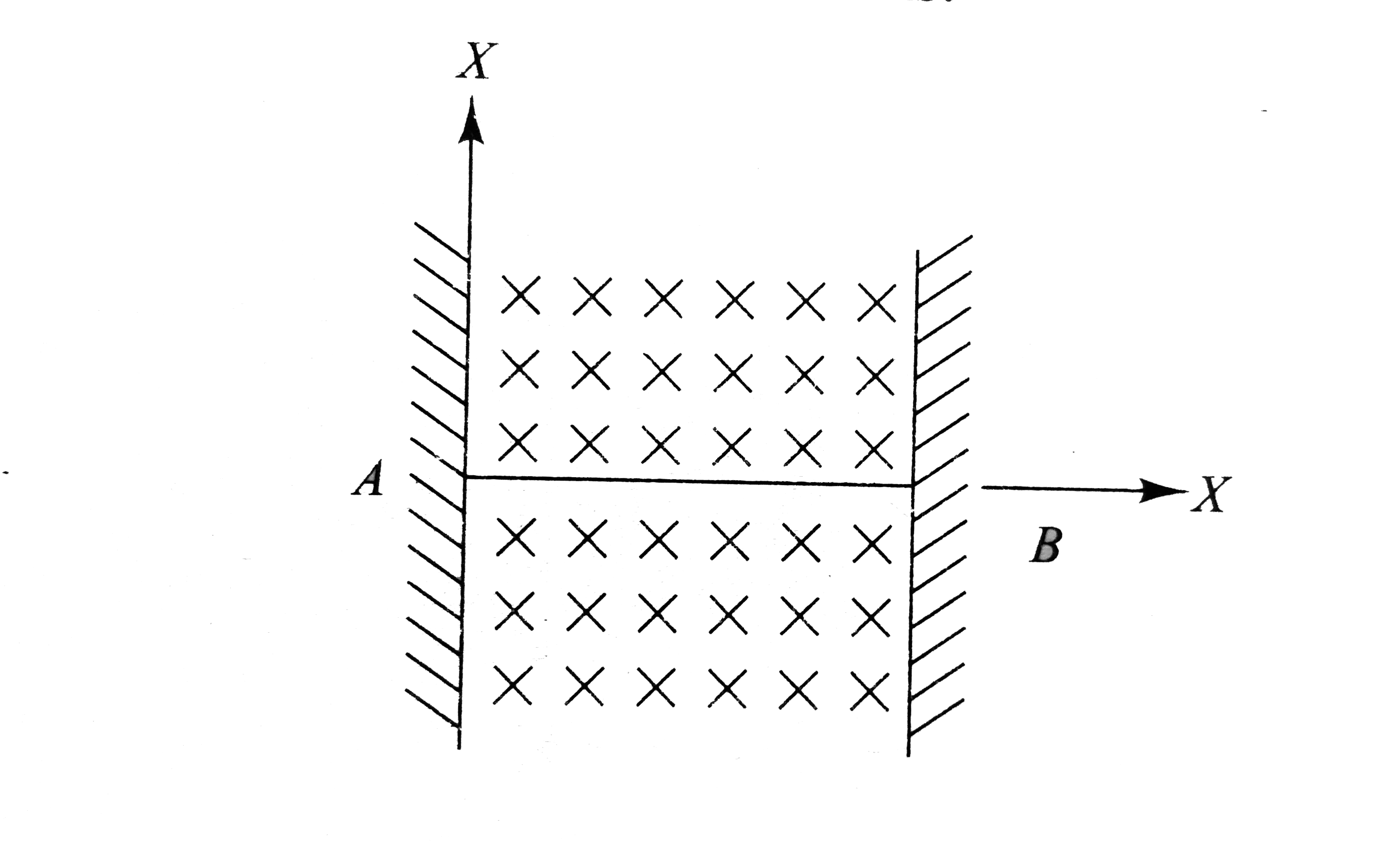
In which of the following modes the emf induced in $A B$ is always zero?
A(a) fundamental modes
B(b) second harmonic
C( c) ssecond overtone
D(d) fourth overtone
Question 3 - Select One
There are two infinite parallel current carrying wires in vertical plane. Lower wire is fixed and upper wire is having a linear mass density $λ$ . Two wires are carrying currents $I_{1}$ and $I_{2}$ . Now upper wire is placed in a magnetic field produced by lower wire. Magnetic field due to lower wire at the location of upper wire is $\frac{μ_{0} I}{2 π d}$ , where $I_{1}$ current lower wire, $d$ separation between wires.
Force on any small portion of upper wire having length $d l$ is $d F = \frac{μ_{0} I_{1} I_{2} d l}{2 π d},$ where $I_{2}$ current in the upper wire. If directions of current in the wires is appropriate then upper wire can be in equilibrium if its weight is balanced by magnetic force. Now answer the following questions.
If upper wire is slightly displaced from its mean position and released it will perform simple harmonic motion. As wire then total mechanical energy of wire
ARemains constant
BDecreases
CWe can't say anything about mechanical energy in magnetic field
DIncreases

CENGAGE PHYSICS-ELECTROMAGNETIC INDUCTION-Exercises Linked Comprehension

Home
Ask Doubt
Profile

A standing wave y=2Asinkxcosωt is set up in the wire AB fixed at both ends by two vertical walls (see Fig. 3.197). The region between the walls contains a constant magnetic field B. Now answer the following question: The wire is found to vibrate in the third harmonic. The maximum emf induced is

The correct Answer is:A

(a) y=2Asinkxcosωt v=dydt=−2Asinkxωsinωt vmax=−2Aωsinkx,k=3πAB e=∫l=AB0bvmaxdx=−2aωB∫AB0sinkxdx=+2ωABk[cos(3π)ABAB−cosθ]=−4(AB)ωk ωt=π2 t=π2ω For second harmonic k=2πAB

Topper's Solved these Questions

Similar Questions

Knowledge Check

A stsnding wave y=2Asinkxcosωt is set up in the wire AB fixed at both ends by two vertical walls (see Fig. 3.197). The region between the walls contains a constant magnetic field B. Now answer the following question: In the above qestion, the time when the emf becomes maximum for the time is

A stsnding wave y=2Asinkxcosωt is set up in the wire AB fixed at both ends by two vertical walls (see Fig. 3.197). The region between the walls contains a constant magnetic field B. Now answer the following question: In which of the following modes the emf induced in AB is always zero?

CENGAGE PHYSICS-ELECTROMAGNETIC INDUCTION-Exercises Linked Comprehension