A steel wire of mass 4.0 g and length 80 cm is fixed at the two ends. The tension in the wire is 50 N. Find the frequency and wavelength of the fourth harmonic of the fundamental.
The correct Answer is:frequency = 250 and
To solve the problem, we will follow these steps:
Step 1: Convert the mass and length into appropriate units
- Given mass of the wire = 4.0 g = 4.0 / 1000 kg = 0.004 kg
- Given length of the wire = 80 cm = 80 / 100 m = 0.8 m
Step 2: Calculate the mass per unit length (μ)
- The mass per unit length (μ) is calculated using the formula:
μ=masslength=0.004 kg0.8 m=0.005 kg/m
Step 3: Calculate the wave speed (v) in the wire
- The wave speed (v) in the wire can be calculated using the formula:
v=√Tμ
where T is the tension in the wire.
- Given tension (T) = 50 N, we can substitute the values:
v=√50 N0.005 kg/m=√10000=100 m/s
Step 4: Determine the wavelength (λ) of the fourth harmonic
- For a wire fixed at both ends, the wavelength of the nth harmonic is given by:
λn=2Ln
where L is the length of the wire and n is the harmonic number.
- For the fourth harmonic (n = 4):
λ4=2×0.8 m4=1.6 m4=0.4 m=40 cm
Step 5: Calculate the frequency (f) of the fourth harmonic
- The frequency can be calculated using the formula:
f=vλ
- Substituting the values we found:
f=100 m/s0.4 m=250 Hz
Final Answers:
- Wavelength (λ) = 40 cm
- Frequency (f) = 250 Hz
---
- Given mass of the wire = 4.0 g = 4.0 / 1000 kg = 0.004 kg
- Given length of the wire = 80 cm = 80 / 100 m = 0.8 m
- The mass per unit length (μ) is calculated using the formula:
μ=masslength=0.004 kg0.8 m=0.005 kg/m
- The wave speed (v) in the wire can be calculated using the formula:
v=√Tμ
where T is the tension in the wire.
- Given tension (T) = 50 N, we can substitute the values:
v=√50 N0.005 kg/m=√10000=100 m/s
- For a wire fixed at both ends, the wavelength of the nth harmonic is given by:
λn=2Ln
where L is the length of the wire and n is the harmonic number.
- For the fourth harmonic (n = 4):
λ4=2×0.8 m4=1.6 m4=0.4 m=40 cm
- The frequency can be calculated using the formula:
f=vλ
- Substituting the values we found:
f=100 m/s0.4 m=250 Hz
- Wavelength (λ) = 40 cm
- Frequency (f) = 250 Hz
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Question for short Answer7videos
WAVE MOTION AND WAVES ON A STRING
- BOOK - HC VERMA ENGLISHCHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Objective -29videos
WAVE MOTION AND WAVES ON A STRING
- BOOK - HC VERMA ENGLISHCHAPTER - THE FORCESEXERCISE - Questions for short Answer9videos
THE FORCES
- BOOK - HC VERMA ENGLISHCHAPTER - WORK AND ENERGYEXERCISE - Question for Short Answer16videos
WORK AND ENERGY
Similar Questions
A 2.00 m-long rope, having a mass of 80 g, is fixed at one end and is tied to a light string at the other end. The tension in the string is 256 N. (a) Find the frequencies of the fundamental and the first two overtones. (b) Find the wavelength in the fundamental and the first two overtones.
View SolutionA turning fork is sounded together with a stretched sonometer wire of length 50 cm. When the tension in the wire is 100 N, 4 beats are heard. Find the frequency of the tuning fork if the same number of beats are heard when the tention in the wire reduced to 81 N.
View SolutionA 1m long rope, having a mass of 40g, is fixed at one end and is tied to a light string at the other end. The tension in the string in 400N. Find the wavelength in second overtone (in cm).
View SolutionA sonometer wire has a total length of 1m between the fixed ends. Where should the two bridges be placed below the wire so that the three segments of the wire have their fundamental frequencies in the ratio 1 : 2 : 3 ?
View SolutionA sonometer wire has a total length of 1 m between the fixed ends. Where should the two bridges be placed below the wire so that the three segments of the wire have their fundamental frequencies in the ratio 1 : 2 : 3 ?
View SolutionA metal wire of diameter 1mm is held on two knife by a distance 50cm . The tension in the wire is 100N. The wire vibrating with its fundamental frequency and a vibrationg tuning fork together produce 5beats/s. The tension in the wire is then reduced to 81N. When the two excited , beats are heard at the same rate .
Calculate
i. the frequency of the fork and
ii. the density of material of wireView SolutionA piano wire with mass 3.00 g and length 80.0 cm is stretched with a tension of 25.0 N. A wave with frequancy 120.0 Hz and amplitude 1.6 mm travels along the wire. (a) calculate the average power carried by the wave. (b) what happenes to the avarage power if the wave amplitude is halved?
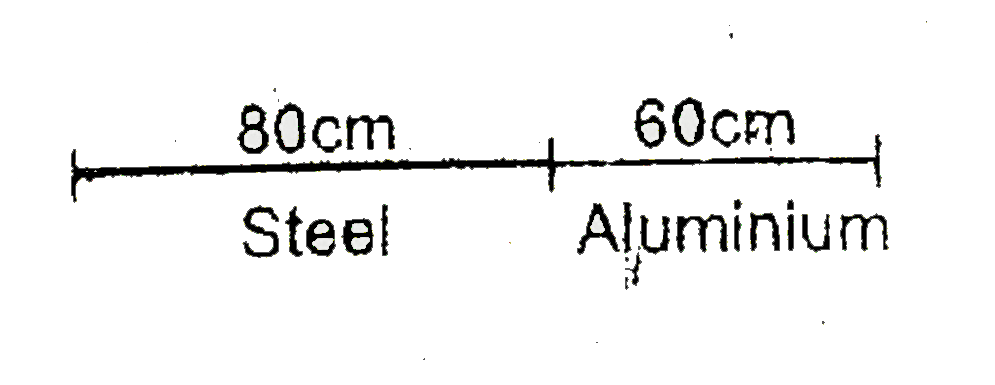
View SolutionFigure shows an aluminium wire of length 60cm joined to a steel wire of length 80cm and stretched between two fixed supports. The tension produced is 40N. The cross-sectional area of the steel wire is 1.0mm2 and that of the aluminimum wire is 3.0mm2 The minimum frequency of a tuning fork which can produce standing waves in the system with the joint as a node is 10P (in Hz) the find P. Given density of aluminimum is 2.6g/cm3 and that of steel is 7.8g/cm3.
 View Solution
View SolutionA metal wire of diameter 1mm is held on two knife edges by a distance 50cm. The tension in the wire is 100N. The wire vibrating with its fundamental frequency and a vibrating tuning fork together produce 5beats/s. The tension in the wire is then reduced to 81N. When the two are excited, beats are heard at the same rate. Calculate
(a) frequency of a fork and
(b) the density of material of wire.View SolutionThe total length of a sonometer wire fixed between two bridges is 110 cm. Now, two more bridges are placed to divide the length of the wire in the ratio 6:3:2. If the tension in the wire is 400 N and the mass per unit length of the wire is 0.01 kg m−1, then the minimum common frequency with which all the three parts can vibrate, is
View Solution

