A stiff wire is bent into a cylinder loop of diameter D. It is clamped by knife edges at two points opposite to each other . A transverse wave is sent around the loop by means resonance frequency (fundamental mode) of the loop in terms of wave speed v and diameter D is
vD
2vπD
vπD
v2πD
The correct Answer is:C
To find the resonance frequency (fundamental mode) of a stiff wire bent into a cylindrical loop of diameter D, we can follow these steps:
Step 1: Understand the Setup
The wire is bent into a circular loop and clamped at two opposite points. This creates a standing wave pattern in the loop.
Step 2: Identify the Nodes and Antinodes
In the fundamental mode of vibration, there are two nodes at the clamped points and one antinode in between. The distance between the two nodes is half the wavelength (λ/2).
Step 3: Relate the Wavelength to the Geometry
The circumference of the loop can be expressed in terms of the diameter D:
C=πD
In the fundamental mode, the length of the loop corresponds to half a wavelength:
C=λ2
Thus, we can write:
πD=λ2
From this, we can solve for the wavelength:
λ=2πD
Step 4: Use the Wave Speed Formula
The wave speed v is related to the frequency f and wavelength λ by the formula:
v=fλ
Rearranging this gives us:
f=vλ
Step 5: Substitute for Wavelength
Now substituting the expression for λ into the frequency formula:
f=v2πD
Conclusion
Thus, the fundamental frequency of the loop in terms of wave speed v and diameter D is:
f=v2πD
Final Answer
The resonance frequency (fundamental mode) of the loop is:
f=vπD
---
The wire is bent into a circular loop and clamped at two opposite points. This creates a standing wave pattern in the loop.
In the fundamental mode of vibration, there are two nodes at the clamped points and one antinode in between. The distance between the two nodes is half the wavelength (λ/2).
The circumference of the loop can be expressed in terms of the diameter D:
C=πD
In the fundamental mode, the length of the loop corresponds to half a wavelength:
C=λ2
Thus, we can write:
πD=λ2
From this, we can solve for the wavelength:
λ=2πD
The wave speed v is related to the frequency f and wavelength λ by the formula:
v=fλ
Rearranging this gives us:
f=vλ
Now substituting the expression for λ into the frequency formula:
f=v2πD
Thus, the fundamental frequency of the loop in terms of wave speed v and diameter D is:
f=v2πD
The resonance frequency (fundamental mode) of the loop is:
f=vπD
Topper's Solved these Questions
- SUPERPOSITION AND STANDING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Multiple
26 Videos
- SUPERPOSITION AND STANDING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Assertion - Reasoning
6 Videos
- SUPERPOSITION AND STANDING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Subjective
24 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer
16 Videos
- THERMODYNAMICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - 24
1 Videos
Similar Questions
The figure shows a circular loop of radius a with two long parallel wires (νmbered1and2) all in the plane of the paper . The distance of each wire from the centre of the loop is d. The loop and the wire are carrying the same current I. The current in the loop is in the counterclockwise direction if seen from above.
(q) The magnetic fields(B) at P due to the currents in the wires are in opposite directions.
(r) There is no magnetic field at P.
(s) The wires repel each other.
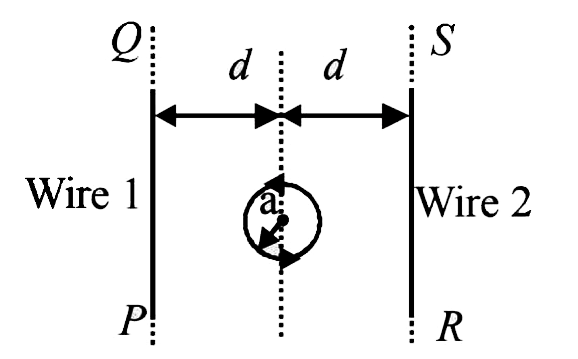
(4) When d≈a but wires are not touching the loop , it is found that the net magnetic field on the axis of the loop at a height h above the loop is zero. In that case
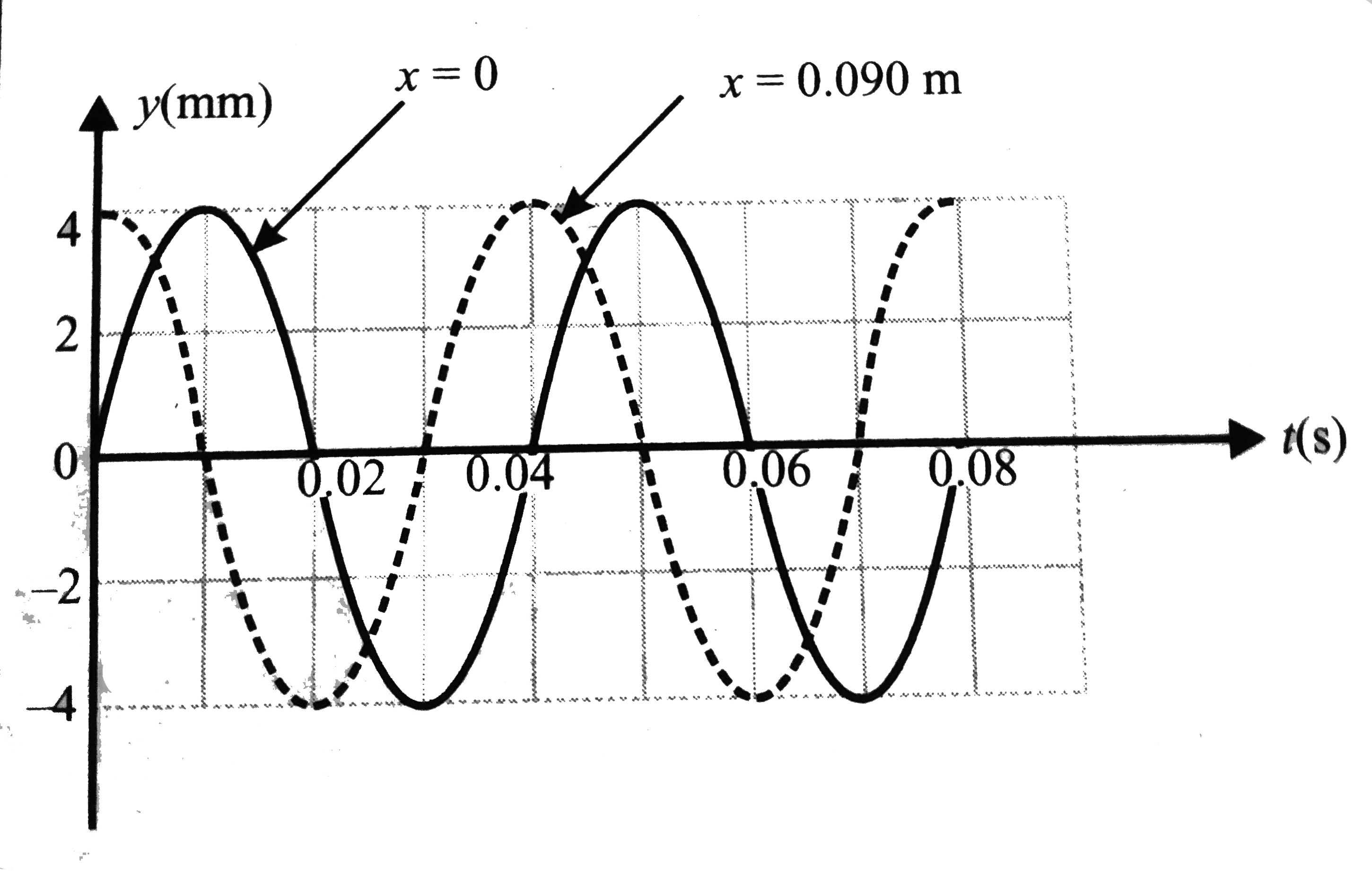
View SolutionA sinusoidal wave is propagating along a streched string that lies along the x-axis. The displacement of the string as a function of time is graphed in for particles at x-0 and at x=0.0900 m. (a) what is the amplitude of the wave? (b)what is the period of the wave? (c ) you are told that the two points x=0 and x=0.0900 m are within one wavelength of each other. if the wave is moving in the +x-direction, determine the wavelength and the wave speed. (d) if instead the wave is moving in the -x-direction, determine the wavelength and the wave speed. (e) would it be possible to derermine definitely the wavelength in parts (c ) and (d) if you were not told that the two points were within one wavelength of each other? why ot why not?
View SolutionThe figure shows a circular loop of radius a with two long parallel wires (numbered 1 and 2) all in the plane of the paper . The distance of each wire from the centre of the loop is d. The loop and the wire are carrying the same current I. The current in the loop is in the counterclockwise direction if seen from above.
(q) The magnetic fields(B) at P due to the currents in the wires are in opposite directions.
(r) There is no magnetic field at P.
(s) The wires repel each other.

(4) When d≈a but wires are not touching the loop , it is found that the net magnetic field on the axis of the loop . In that case
View SolutionThe figure shows a circular loop of radius a with two long parallel wires (νmbered1and2) all in the plane of the paper . The distance of each wire from the centre of the loop is d. The loop and the wire are carrying the same current I. The current in the loop is in the counterclockwise direction if seen from above.
(q) The magnetic fields(B) at P due to the currents in the wires are in opposite directions.
(r) There is no magnetic field at P.
(s) The wires repel each other.
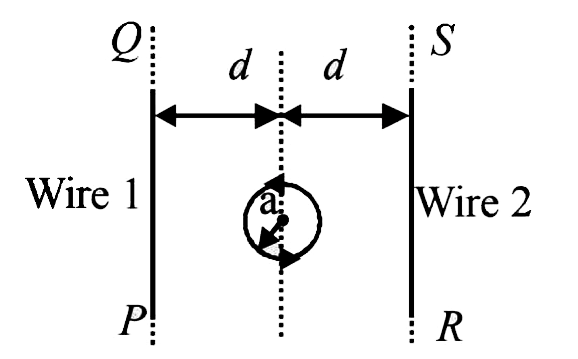
(5) Consider d>>a, and the loop is rotated about its diameter parallel to the wires by 30∘ from the position shown in the figure. If the currents in the wire are in the opposite directions, the torque on the loop at its new position will be ( assume that the net field due to the wires is constant over the loop).
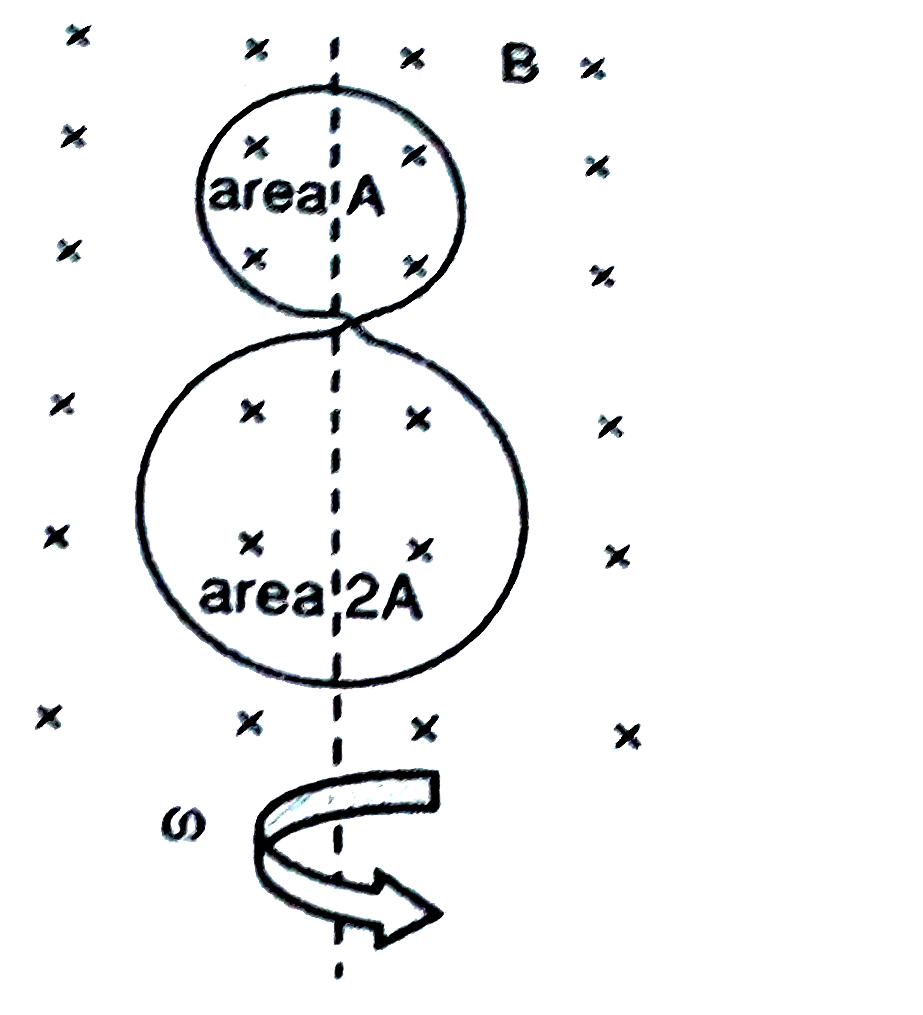
View SolutionA circular insulated copper wire loop is twisted to form two loops of are A and 2A as shown in the figure. At the point of crossing the wires remain electrically insulated from each other. The entire loop lies in the plane ( of the paper). A uniform magnetic →B point into the plane of the paper . At t=0, the loop starts rotation about the common diameter as axis with a constant angular velocity ω in the magnetic field. Which of the following options is /are correct ?
View SolutionA wire with mass 40.0 g is stretched so that its ends are tied down at points 80.0 cm apart.The wire vibrates in its fundamental mode with frequency 60.0 Hz and with an amplitude at the antinodes of 0.300 cm.
(a) What is the speed of propagation of transverse wave in the wire?
Compute the tension in the wire.
(c) Find the maximum transverse velocity and acceleration of particles in the wire.
View SolutionA wire of uniform cross-section is stretched between two points 100cm apart. The wire is fixed at one end and a weight is hung over a pulley at the other end. A weight of 9kg produces a fundamental frequency of 750Hz.
(a) What is the velocity of the wave in wire?
(b) If the weight is reduced to 4kg, what is the velocity of wave?
View SolutionA narrow tube is bent in the form of a circle of radius R, as shown in figure. Two small holes S and D are made in the tube at the position at right angle to each other. A source placed at S generates a wave of intensity I0 which two parts: one part travels along the longer path, while the other travels along the shorter path. Both the waves meet at point D where a detector is placed.

2. If a minima is formed at the detector, then the magnitude of wavelength λ of the wave produced is given by
View SolutionA narrow tube is bent in the form of a circle of radius R, as shown in figure. Two small holes S and D are made in the tube at the position at right angle to each other. A source placed at S generates a wave of intensity I0 which two parts: one part travels along the longer path, while the other travels along the shorter path. Both the waves meet at point D where a detector is placed.

1. If a maxima if formed at a detector, then the magnitude of wavelength λ of the, wave produced is given by
View SolutionA narrow tube is bent in the form of a circle of radius R, as shown in figure. Two small holes S and D are made in the tube at the position at right angle to each other. A source placed at S generates a wave of intensity I0 which two parts: one part travels along the longer path, while the other travels along the shorter path. Both the waves meet at point D where a detector is placed.

The maximum intensity produced at D is given by
View Solution
