A stone dropped from a cliff hits the ground in 4 sec. Height of the cliff is
39.2 m
29.4 m
9.8 m
78.4 m
The correct Answer is:D
To find the height of the cliff from which a stone is dropped, we can use the equations of motion. Here’s a step-by-step solution:
Step 1: Identify the known values
- The time (t) it takes for the stone to hit the ground is given as 4 seconds.
- The initial velocity (u) of the stone when it is dropped is 0 m/s (since it is dropped).
- The acceleration (a) due to gravity (g) is approximately 9.8 m/s².
Step 2: Use the kinematic equation
We will use the equation of motion:
s=ut+12at2
where:
- s is the distance (height of the cliff, h),
- u is the initial velocity,
- a is the acceleration,
- t is the time.
Step 3: Substitute the known values
Since the stone is dropped, we have:
- u=0
- a=g=9.8m/s2
- t=4s
Substituting these values into the equation:
h=0⋅t+12gt2
Step 4: Simplify the equation
Since u=0, the equation simplifies to:
h=12gt2
Step 5: Calculate the height
Now substituting the values of g and t:
h=12⋅9.8⋅(42)
h=12⋅9.8⋅16
h=4.9⋅16
h=78.4meters
Conclusion
The height of the cliff is 78.4 meters.
---
- The time (t) it takes for the stone to hit the ground is given as 4 seconds.
- The initial velocity (u) of the stone when it is dropped is 0 m/s (since it is dropped).
- The acceleration (a) due to gravity (g) is approximately 9.8 m/s².
We will use the equation of motion:
s=ut+12at2
where:
- s is the distance (height of the cliff, h),
- u is the initial velocity,
- a is the acceleration,
- t is the time.
Since the stone is dropped, we have:
- u=0
- a=g=9.8m/s2
- t=4s
h=0⋅t+12gt2
Since u=0, the equation simplifies to:
h=12gt2
Now substituting the values of g and t:
h=12⋅9.8⋅(42)
h=12⋅9.8⋅16
h=4.9⋅16
h=78.4meters
The height of the cliff is 78.4 meters.
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - problems
47 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - Numerical exercise(LEVEL-1)
22 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - EXERCISE -II
80 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - QUESTION FOR DESCRIPTIVE ANSWER
7 Videos
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH SERIESCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - EXERCISE-III
49 Videos
Similar Questions
A stone is dropped from a height h . It hits the ground with a certain momentum P . If the same stone is dropped from a height 100% more thanthe preyiious height, the momentum when it hits the ground will change by
View SolutionA stone dropped from the top of a tower reaches the ground in 3 s. The height of the tower is
View Solution
Knowledge Check
A ball of mass m is dropped from a cliff of height H. The ratio of its kinetic energy to the potential energy when it is fallen through a height 3/4 H is
A3:4B4:3C1:3D3:1A rock is dropped off a cliff and strikes the ground with an impact velocity of 30m/s. How high was the cliff ?
A15mB20mC30mD45 mA stone is dropped from the top of a tower and one second later, a second stone is thrown vertically downward with a velocity 20ms−1. The second stone will overtake the first after travelling a distance of (g=10ms−2)
Ag2⎡⎢ ⎢⎣n(u−gn2)(u−gn)⎤⎥ ⎥⎦2Bg2⎡⎢ ⎢⎣n(u2−gn)u−gn⎤⎥ ⎥⎦2Cg2⎡⎢ ⎢⎣n(u2−gn)u2−gn⎤⎥ ⎥⎦2Dg5[u−gnu2−gn]2
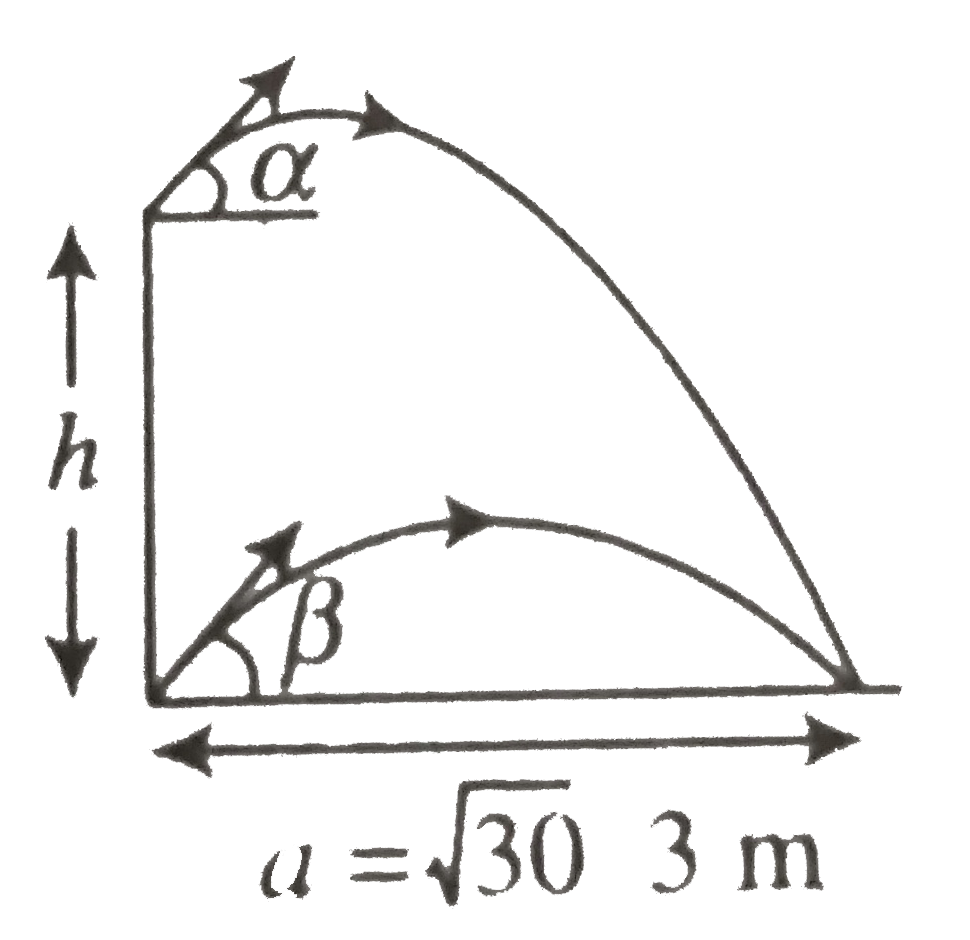
Shots are fired simultaneously from the top and bottom of a vertical cliff with the elevation α=30∘, beta =60∘, respectively. The shots strike an object simultaneously at the same point. If a=10(√3) m is the horizontal distance of the object from the cliff, then the height h of the cliff is
View SolutionA stone is dropped from top of a cliff.It is seen to hit the ground below after 4.2 s. How high is the cliff?
View SolutionA stone is dropped from the top of a tower. If it hits the ground after 10 seconds, what is the height of the tower?
View SolutionA boat is being rowed away from a cliff 150 meter high. At the top of the cliff the angle of depression of the boat changes from 60∘ to 45∘ in 2 minutes. Find the speed of the boat.
View SolutionA stone drop from height h reaches to earth surface in 1 sec. if the same stone taken to moon and drop freely then it will reaches from the surface of the moon in the time (the 'g' of moon is 1/6 times of earth)-
View Solution
