A stone is allowed to fall form the top of a tower 100m high and at the same time another stone is projected vertically upwards from the ground with a velocity of 25 m/s calculate. When and where the two stones will meet.
Let t be the point at which two stones meet and let h be their height from the ground. It is given in the equation that height of the tower is h=100m.
Now first consider the stone which falls from the top of the tower. So distance covered by this stone at time .t. can be calculated using equation of motion.
X−Xo=uot+3/2gt2
Since initial velocity u=o so we get
100−x=1/2gt2 (1)
The distance covered by the same that is thrown in upwards direction from ground is
x=25t−1/2gt2 (2)
Adding equation 1 and 2 we get t=4s Putting value to eqn (2)
X=25×4−1/2×9.8×(4)2=100−78.4
=21.6m
Topper's Solved these Questions
- BOOK - CPC CAMBRIDGE PUBLICATIONCHAPTER - GRAVITATIONEXERCISE - ADDITIONAL QUESTIONS (ANSWER THE FOLLOWING)10videos
GRAVITATION
- BOOK - CPC CAMBRIDGE PUBLICATIONCHAPTER - GRAVITATIONEXERCISE - ADDITIONAL QUESTIONS (FILL IN THE BLANKS)5videos
GRAVITATION
- BOOK - CPC CAMBRIDGE PUBLICATIONCHAPTER - GRAVITATIONEXERCISE - ADDITIONAL QUESTIONS (MATCH THE FOLLOWING)1videos
GRAVITATION
- BOOK - CPC CAMBRIDGE PUBLICATIONCHAPTER - FORCE AND LAWS OF MOTIONEXERCISE - UNIT TEST2videos
FORCE AND LAWS OF MOTION
- BOOK - CPC CAMBRIDGE PUBLICATIONCHAPTER - MOTIONEXERCISE - Unit Test6videos
MOTION
Similar Questions
A stone is allowed to fall from the top of a tower 100m high and at the same time another stone is projected vertically upwards from the ground with a velocity of 25ms.Calculate when and where the two stones will meet.
View SolutionA stone is allowed to fall from the top of a tower 100m high and at the same time another stone is projected vertically upwards from the ground with a velocity of 25m/s. Calculate when and where the two stones will meet.
View Solution
Knowledge Check
- Question 1 - Select One
A stone is dropped from the top of a 400m high tower. At the same time another stone is projected vertically upwards from the ground with a speed of 50m/s. The two stones will cross each other after a time
A2 sB4 sC6 sD8 s
A stone is allowed to fall from the top of a tower 100m high and at the same time another stone is projected vertically upwards from the ground with a velocity of 25m/s. Calculate when and where the two stone will meet.
View Solution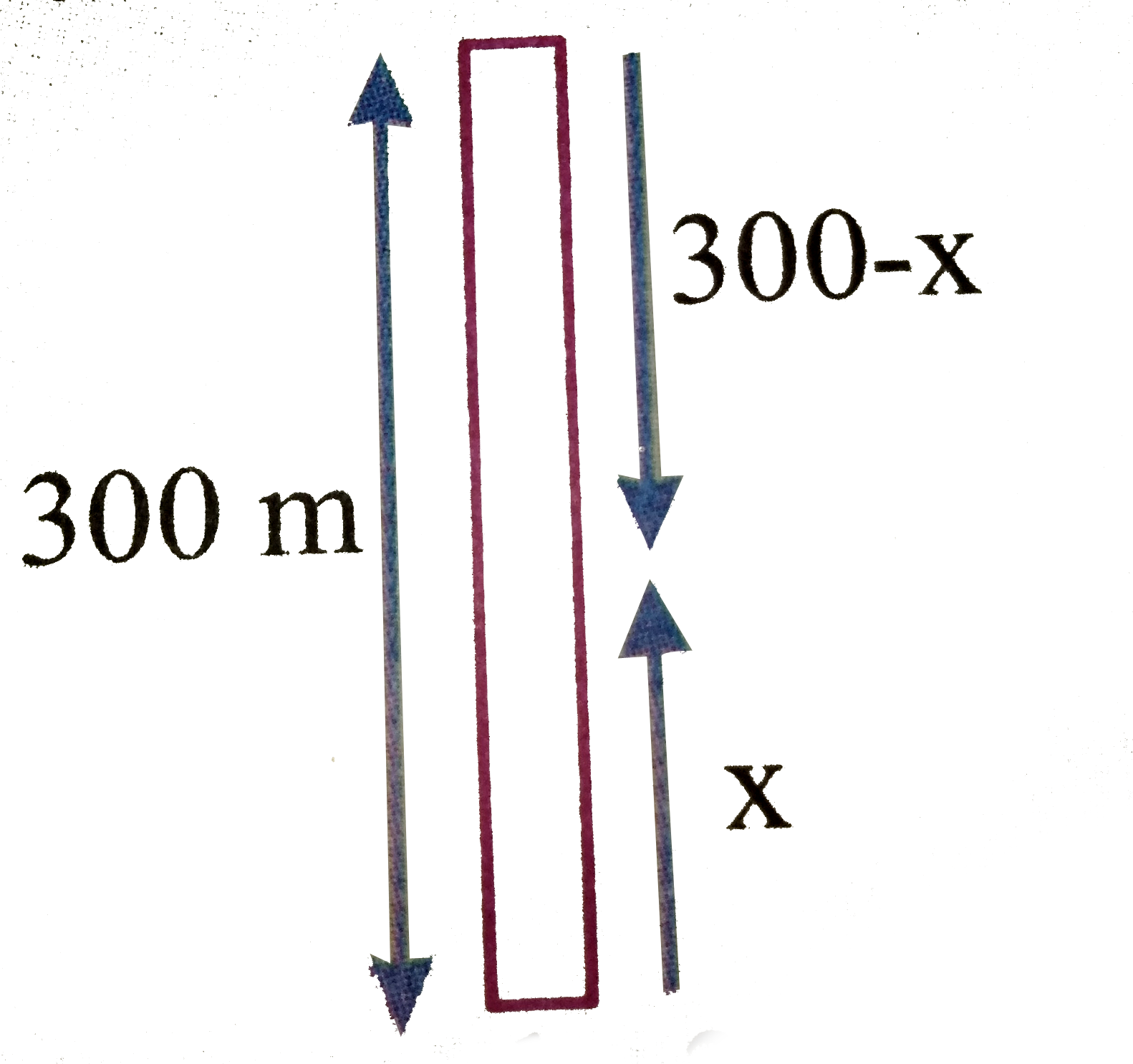
A stone is allowed to fall from the top of a tower 300 m height and at the same time another stone is projected vertically up from the ground with a velocity 100m/s. Find when and where the two stones meet?View SolutionA stone is allowed to fall from the top of a tower 300 m height and at the same time another stone is projected vertically up from the ground with a velocity 100ms−1 . Find when and where the two stones meet ?
View SolutionA stone is dropped from the top of a tower 200 m high. At the same time, another stone is projected vertically upwards from the ground with a velocity of 40m/s. Calcuate when and where the two stones will mest.
View SolutionA stone is dropped from a height 300 m and at the same time another stone is projected vertically upwards with a velocity of 100m/sec. Find when and where the two stones meet.
View SolutionForm the top of a tower 100m in height a ball is dropped and at the same time another ball is projected vertically upwards from the ground with a velcoity of 25ms−1. Find when and where the two balls will meet.
View SolutionFrom the top of a tower 100 m in height a ball is dropped and at the same time another ball is projected vertically upwards from the ground with a velocity of 25 m/s. Find when and where the two balls will meet? (g=9.8m/s)
View Solution
