A straight conductor of uniform cross-section carries a current I. Let s= specific charge of an electron. The momentum of all the free electrons per unit length of the conductor, due to their drift velocity only, is
i.s
√i/(s)
i/s
(is)2
The correct Answer is:C
Current flowing in a straight conductor
i=nAevd or vd=inAe
Total number of free electrons in the unit length of conductor, N=nA×l=nA×1 (length, l = 1m). Total linear momentum of all the free electrons per unit length =Nmvd=nAm×inAe=i0(e/m)=is
where, s is charge per unit mass (e/m).
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - CURRENT ELECTRICITYEXERCISE - MHT CET CORNER
32 Videos
- CURRENT ELECTRICITYBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE 1
75 Videos
- COMMUNICATION SYSTEMBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - COMMUNICATION SYSTEMEXERCISE - MHC CET Corner
6 Videos
- ELASTICITY BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - ELASTICITY EXERCISE - MHT CET CORNER
16 Videos
Similar Questions
A current I is passed through a silver strip of width d and area of cross-section A. The number of free electrons per unit volume is n
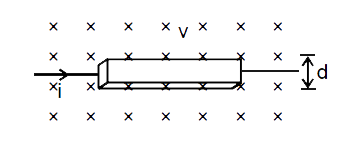
Find the drift velocity v of the electrons.
View SolutionIf A is the area of cross section of conductor, e be the charge on the electrons, n be the number of electrons per unit volume and J be the current density then drift velocity of electrons is
View Solution
Knowledge Check
In a straight conductor of uniform cross-section charege q is flowing for time t. Let s be the specific charge of an electron. The momentum of all the free electrons per unit length of the free electrons per unit length of the conductor, due to their drift velocity only is
AqtsB(qts)2C√qtsDqtsDrift velocity of free electrons when current passes through the conductor is of the order of
A10mm/sB10m/sC10km/sD106m/sA straight conductor of uniform cross section carries a time varying current, which varies at the rate dI//dt = I. If s is the specific charge that is carried by each charge carries of the conductor and l is the length of the condcutor, then the totalt force experienced by all the charge carries per unit length of the conductor due to their drift velocities only is
AF=IsBF=I2√lsCF=IsDF=2Ils
In a metal in the solid state, such as a copper wire, the atoms are strongly bound to one another and occupý fixed positions. Some electrons (called the conductor electrons) are free to move in the body of the metal while the other are strongly bound to their atoms. In good conductors, the number of free electrons is very large of the order of 1028 electrons per cubic metre in copper. The free electrons are in random motion and keep colliding with atoms. At room temperature, they move with velocities of the order of 105 m/s. These velocities are completely random and there is not net flow of charge in any directions. If a potential difference is maintained between the ends of the metal wire (by connecting it across a battery), an electric field is set up which accelerates the free electrons: These accelerated electrons frequently collide with the atoms of the conductor, as a result, they acquire a constant speed called the drift speed which is given by Ve= 1/enA where I = current in the conductor due to drifting electrons, e = charge of electron, n = number of free electrons per unit volume of the conductor and A = area of cross-section of the conductor. The drift speed of free electrons in a conductor depends upon
View SolutionWhen current flows through a conductor, then the order of drift velocity of electrons will be
View SolutionThere is a current of 40 A in a wire 10−6m2 area of cross-section. If the number of free electrons per cubic metre is 1029, then the drift velocity is :
View SolutionThe force acting per unit length on one conductor due to the other for two long parallel current - carrying wires is
View SolutionA conductor of length of l and area of cross section A has n number of electrons per unit volume of the conductor. The total charge carried by the conductor is
View Solution