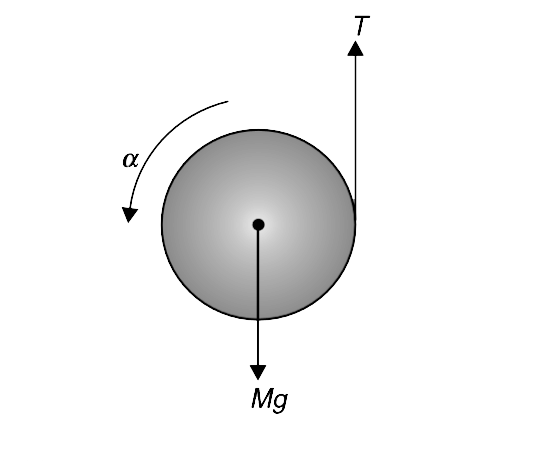
A string is wrapped around a cylinder of mass M and radius R. The string is pulled vertically upwards to prevent the centre of mass from falling as the cylinder unwinds the string. The tension in the string is
Mg6
Mg3
Mg2
2Mg3
The correct Answer is:B
Equation of motion
Mg−T=Ma ………….i
Taking torque about the axis passing through the centre of the spool and perpendicular to it
TR=Iα=12MR2(a2)
T=12Ma …………ii
From eqn i and ii
Ma=Mg−12ma
a=2g3
∴T=Mg3
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Multiple Correct26videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Linked Comprehension71videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Subjective19videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer11videos
RIGID BODY DYNAMICS 1
- BOOK - CENGAGE PHYSICSCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer16videos
SOUND WAVES AND DOPPLER EFFECT
Similar Questions
A string is wrapped around a cylinder of mass M and radius r. The string is pulled vertically upward to prevent the center of mass from falling as the cylinder unwinds the string. (a) What is the tension in the string? B) How much work has been done on the cylinder once it has reached an angular speed ω? c) what is the length of string unwound in the time its acquires angular speed ω?
View SolutionA string is wrapped around a cylinder of mass M and radius R(=2√2m).The string is pulled vertically upward to prevent the centre of mass from falling as the cylinder unwinds the string.Find the length of the string unwound when the cylinder has reached a speed ω(=5rad/s).(g=10ms2)
View Solution
Knowledge Check
- Question 1 - Select One
A string of negligible mass is wrapped on a cylindrical pulley of mass M and radius R. The other end of string is tied to a bucket of mass m. If the pulley rotates about a horizontal axis then the tension in the string is :
AmgB(M+m)gCMmg(M+2m)D2mg(M+2m) - Question 2 - Select One
A string is warapped around a cylinder of mass m and radius r. The string is also connected to a block of same mass m with the help of another pulley as shown in figure. The angular acceleration of the cylinder is ( friction is sufficient for rolling ) ( all pulleys are ideal)
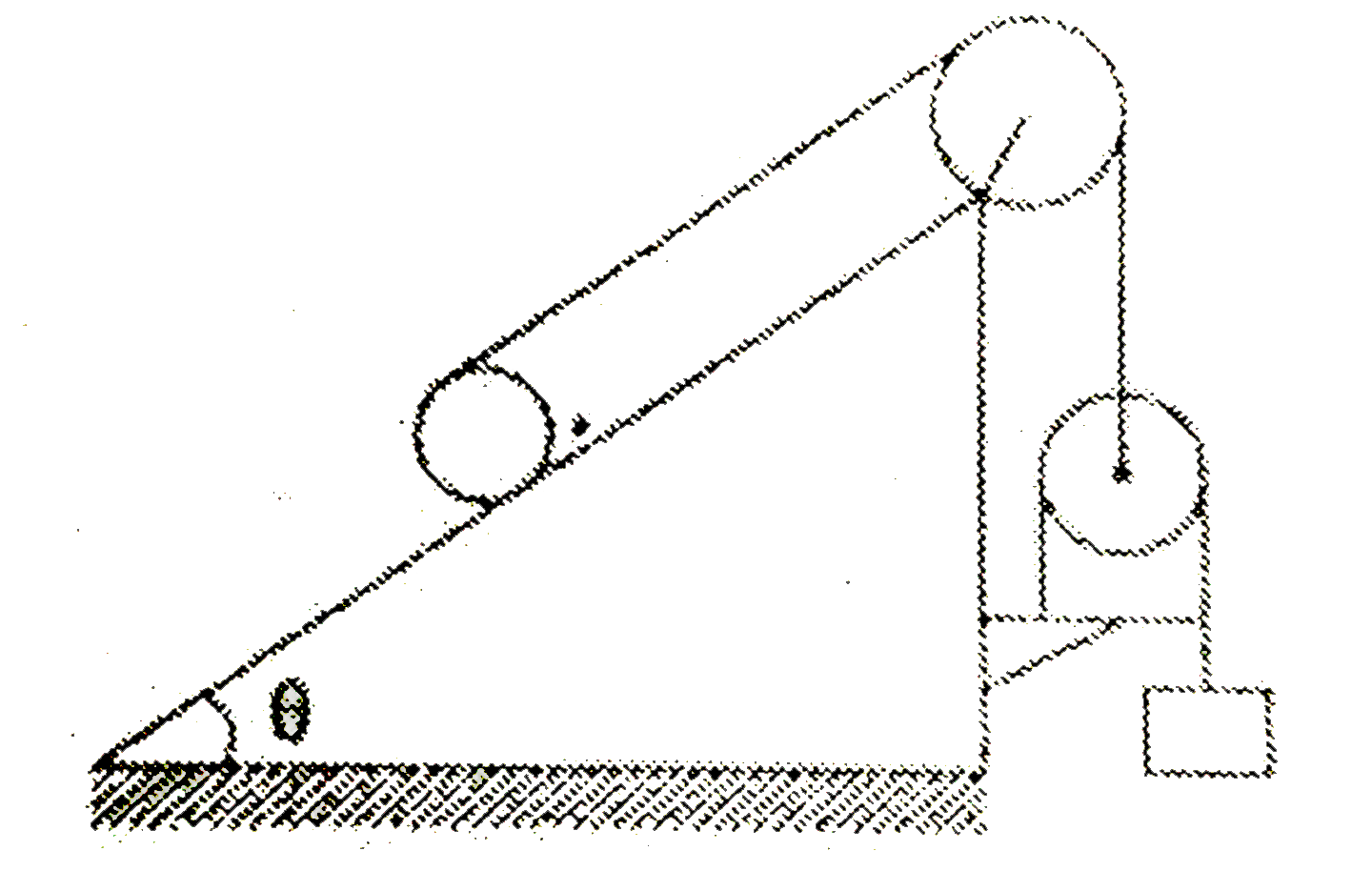 Ag(2−sinθ)16RB2g(4−sinθ)35RC7g(3−sinθ)25RDg(2−sinθ)12R
Ag(2−sinθ)16RB2g(4−sinθ)35RC7g(3−sinθ)25RDg(2−sinθ)12R - Question 3 - Select One
A string is wrapped several time on a cylinder of mass M and radius R, the cylinder is pivoted about its axis of symmetery . A block mass of m tied to the string rests on support so that the string is black. The block is lifted upto a height h and the support is removed. (shown in figure)
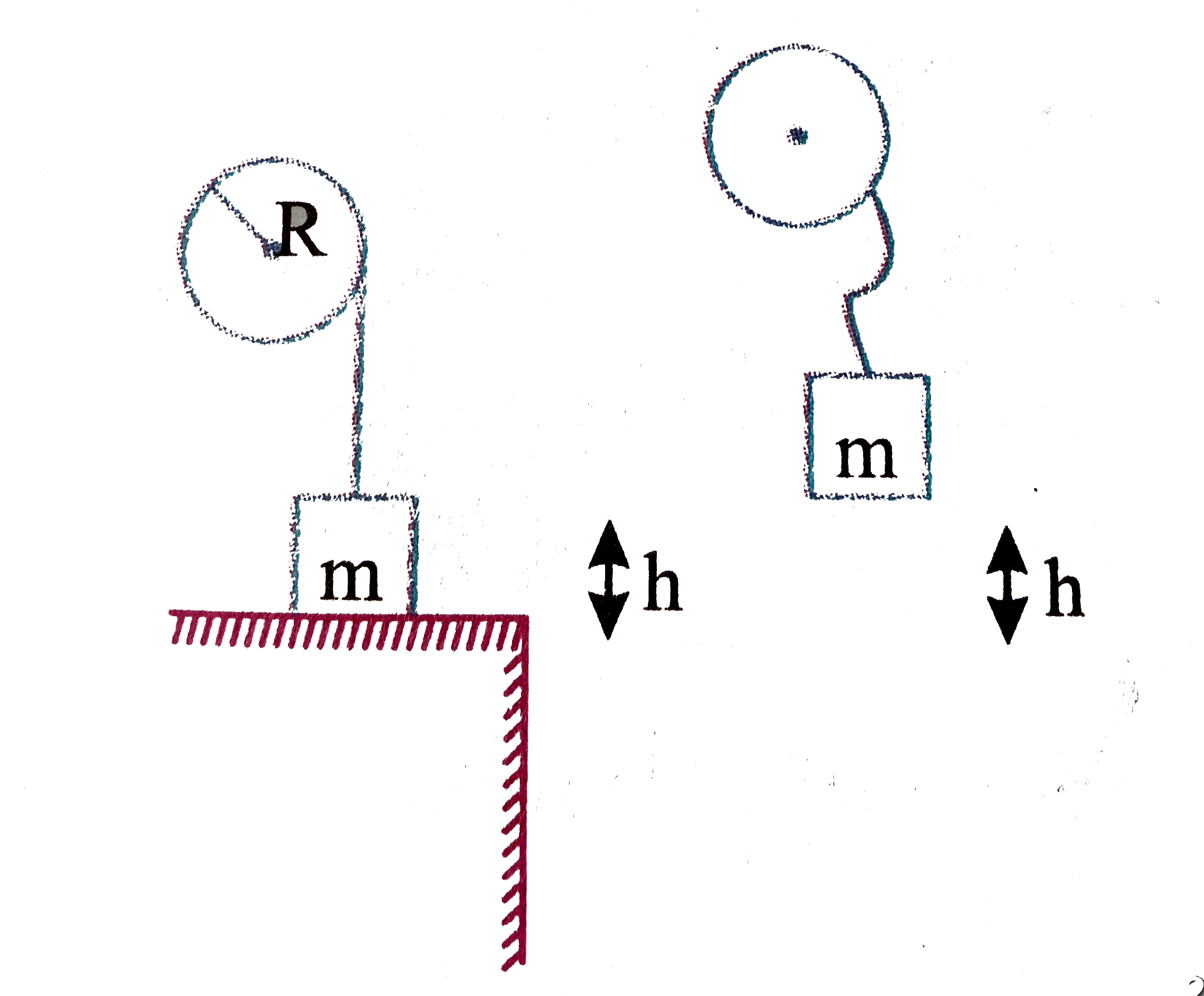
When the string experience a jerk, a large impulsive force is generated for a short duration, so that contribution of weight mg can be neglected suring this duration. then what will be speed of blocks m, just after string has becomes tautA√2gh[1+Mm]B√2gh[1+M2m]C√gh[1+Mm]D√gh[1+M2m]
A string is wrapped around a cylinder of mass M and radius R. The string is pulled vertically upward to prevent the centre of mass from falling as the string unwinds. Assume that the cylinder remains horizontal throughout and the thread does not slip. Find the length of the string unwound when the cylinder has reached an angular speed ω.
 View Solution
View SolutionA string is wound around a hollow cylinder fo mass 5 Kg and radius 0.5 m. If the strinag is now pulled with a horizontal force of 40 N, the cylinder is rolling without slipping on a horizontal surface (see figure), then the angular acceleration of the cyclinder will be (Neglect the mass and thickness of the string).
 View Solution
View SolutionA string is wrapped several time on a cylinder of mass M and radius R, the cylinder is pivoted about its axis of symmetery . A block mass of m tied to the string rests on support so that the string is black. The block is lifted upto a height h and the support is removed. (shown in figure)
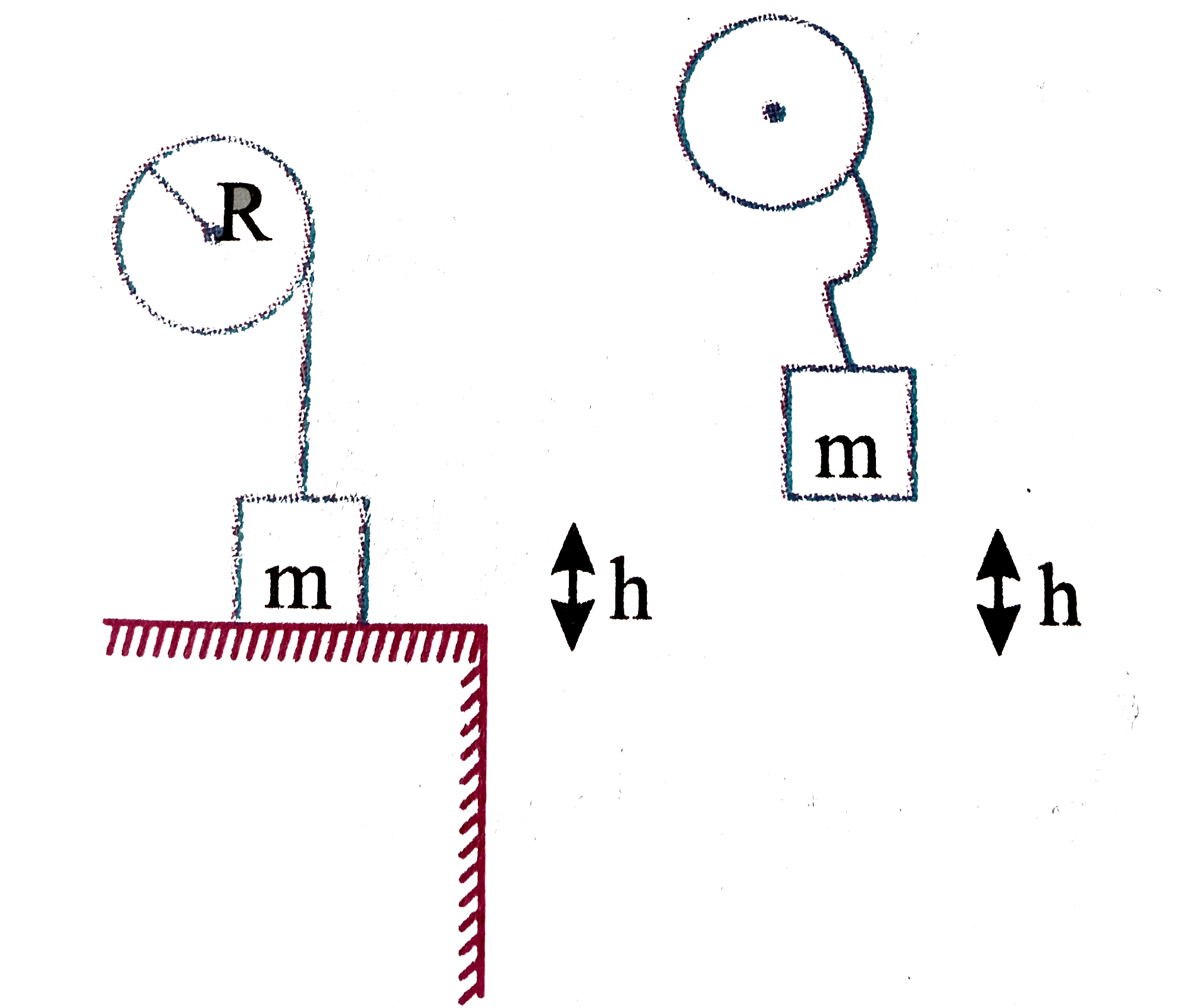
What is the angular velocity of cylinder just before the string becomes tautView SolutionA string is wrapped several time on a cylinder of mass M and radius R, the cylinder is pivoted about its axis of symmetery . A block mass of m tied to the string rests on support so that the string is black. The block is lifted upto a height h and the support is removed. (shown in figure)
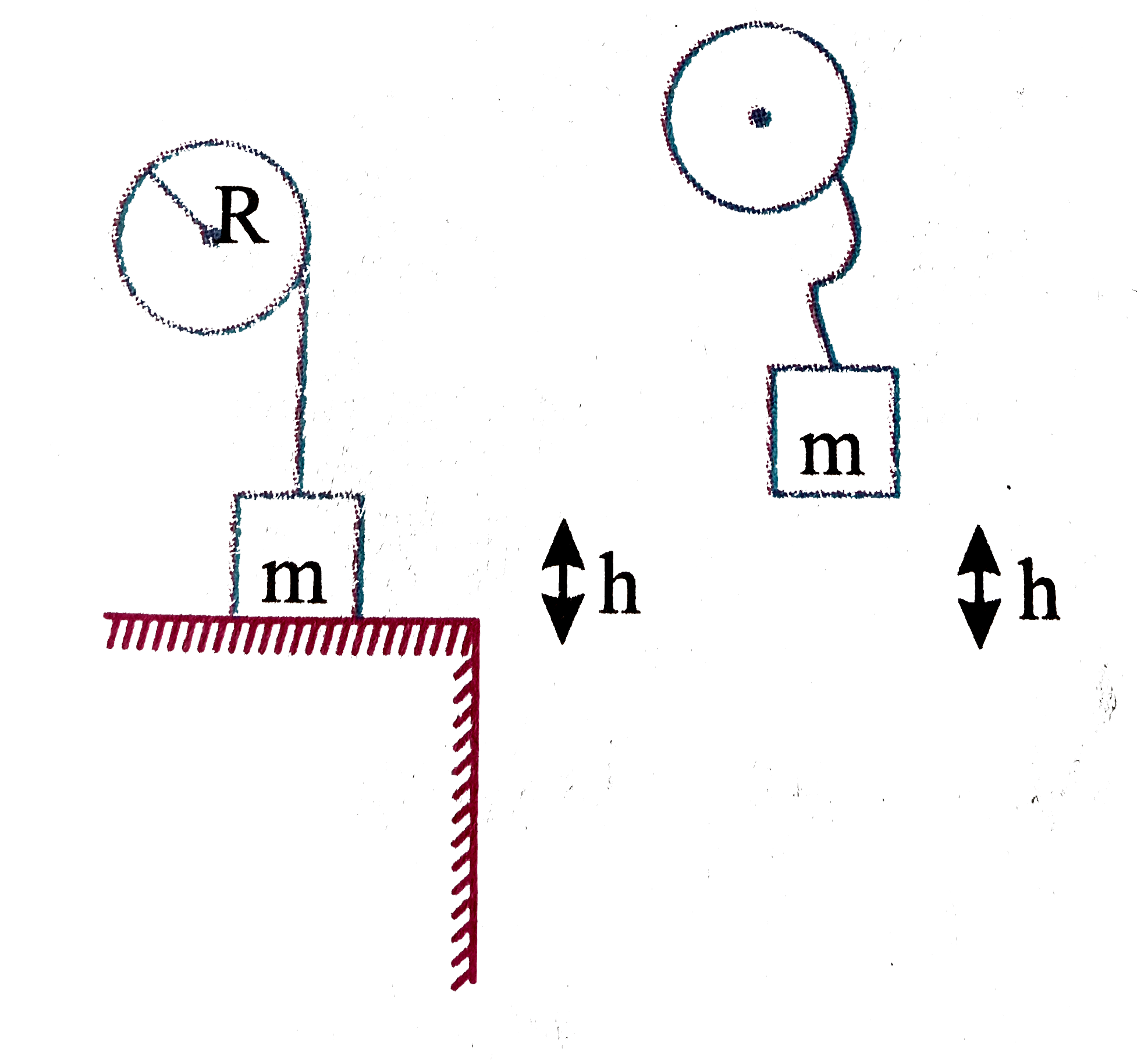
If M=m, what friction of KE is lost due to the jerk developed in the stringView SolutionThe block of mass m is at rest. Find the tension in the string A .
 View Solution
View Solution

