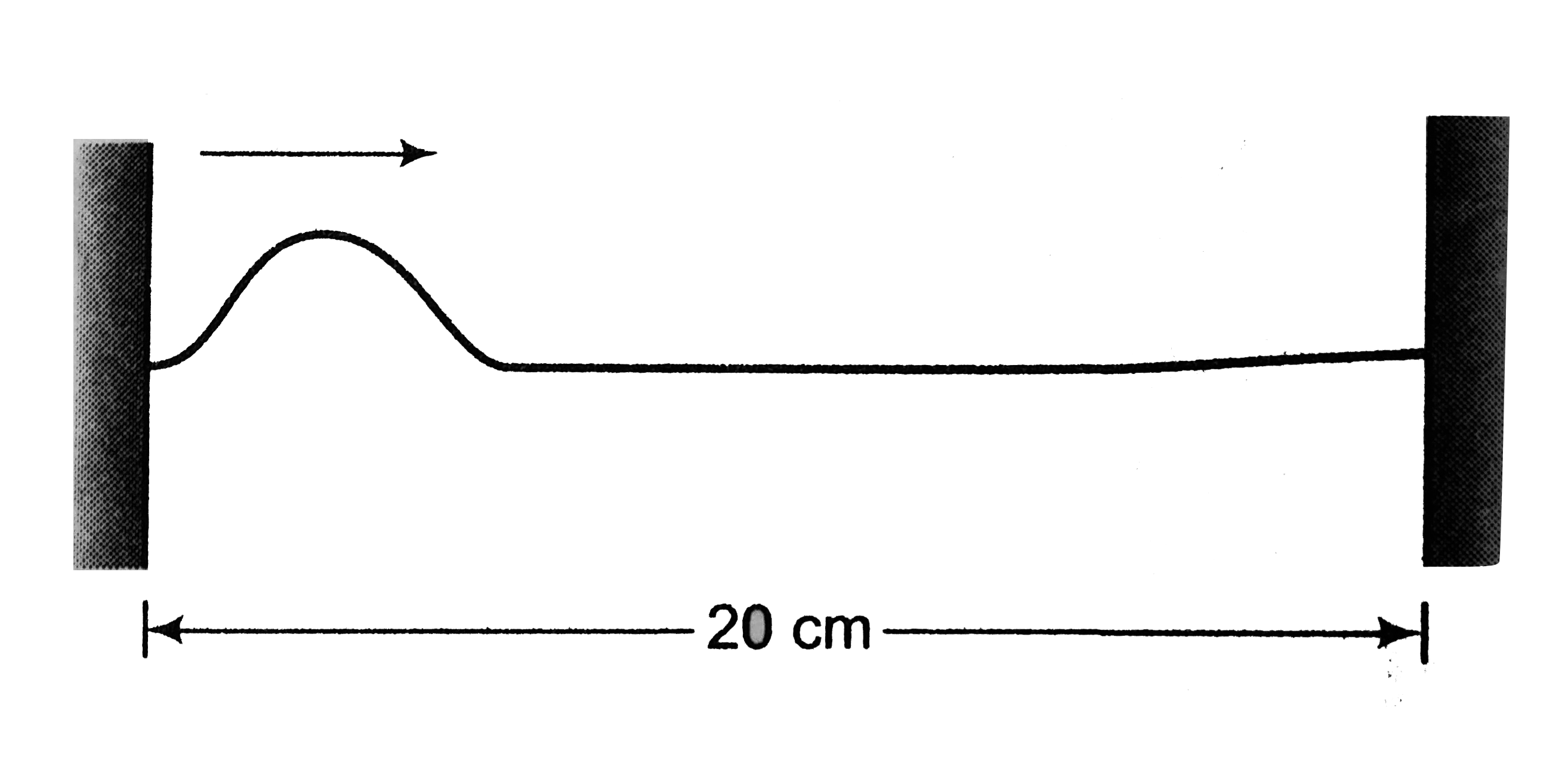
A string of length 20 cm and linear mass density 0.4g/cm is fixed at both ends and is kept under a tension of 16 N. A wave pulse is produced at t=0 near an end as shown in figure which travels towards the other end. (a) When will the string have the shape shown in the figure again? (b) Sketch the shape of the string at a time half of that found in part (a).
 Solution in Tamil
Solution in Tamil
The correct Answer is:A, B
a. Velcity of the wave
v=√(Tm)
√16x150.4
=2000cmsec
∴Timetaken→reach→theotherend<br>=20/2000=0.01sec<br>Timetaken→seetheps–eaga∈∈theorigiNAlposition<br>=0.01xx2=0.02sec
b. At t=0.01s, then will be a trough at the right end as it is reflected.
Topper's Solved these Questions
Similar Questions
A mechanical wave propagastes in a medium along the X-axis. The particles of the medium moves in what direction?
07:06View SolutionThe gravitational force acting on a particle of 1 g due to a similar particle is equal to 6.67×10−17N. Calculate the separation between the particles.
01:12View SolutionThe mass of a cyclist together with the bicycle is 90 kg. Calculate the work done by cyclist if the speed increases from 6km/h to 12 km/h.
04:31View SolutionA string of linear mass density 0.5gcm−1 and a total length 30 cm is tied to a fixed wall at one end and to a frictionless ring at the other end. The ring can move on a vertical rod. A wave pulse is produced on the string which moves towards the ring at a speed of 20cms−1. The pulse is symmetric about its maximum which is located at a distance of 20 cm from the end joined to the ring. (a) Assuming that the wave is reflected from the ends without loss of energy, find the time taken by the string to regain its shape. (b) The shape of the string changes periodically with time. Find this time period. (c) What is the tension in the string ?
 03:24View Solution
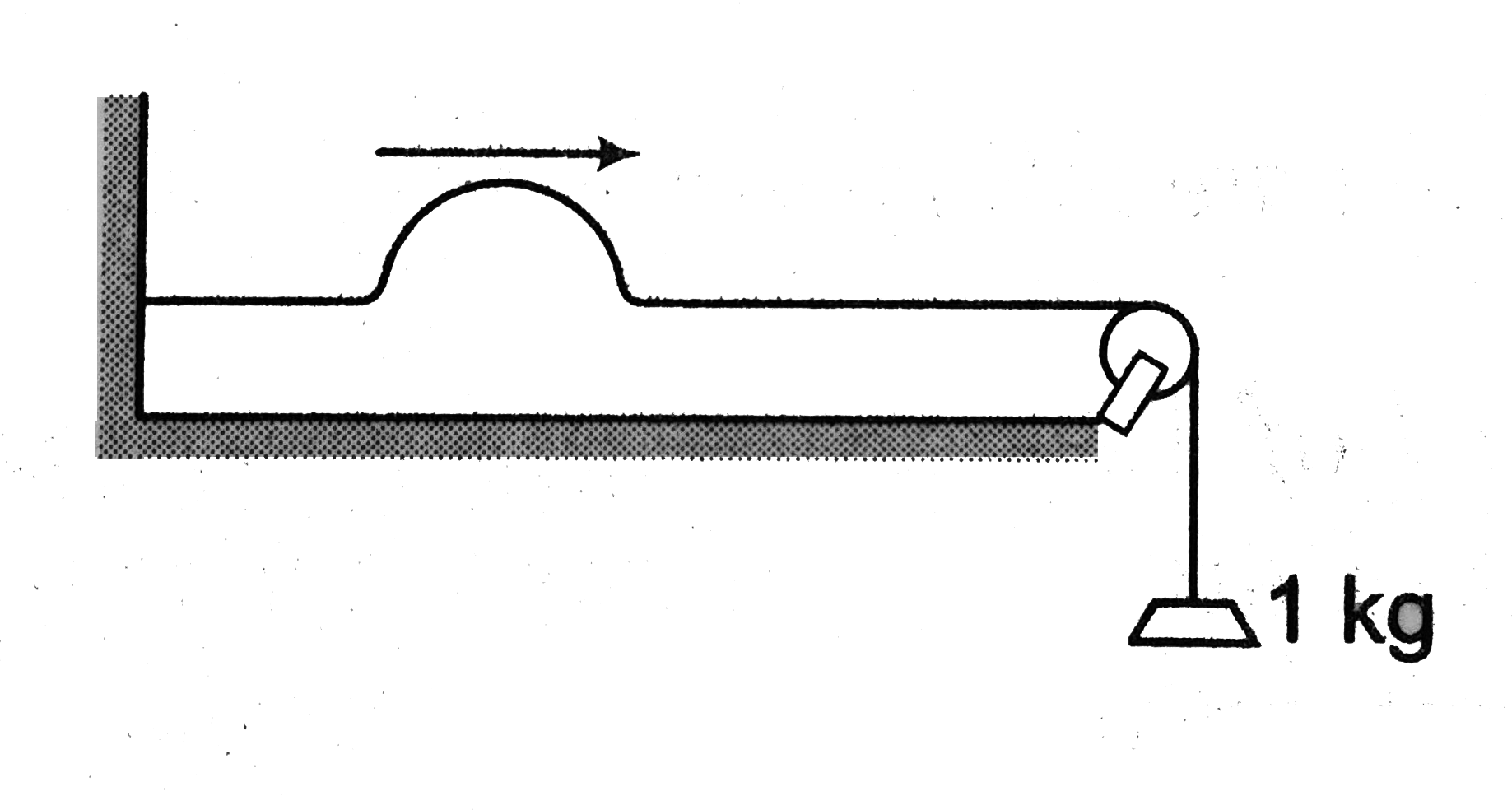
03:24View SolutionFigure shows a string of linear mass density 1.0gcm−1 on which a wave pulse is travelling. Find the time taken by pulse in travelling through a distance of 50cm on the string. Take g=10ms−2
 04:55View Solution
04:55View SolutionA string that is 10 cm long is fixed at both ends. At t=0, a pulse travelling from left to right at 1cm/sis4.0cm from the right end as shown in figure. Determine the next two times when the pulse will be at that point again. State in each case whether the pulse is upright or inverted .
 .05:11View Solution
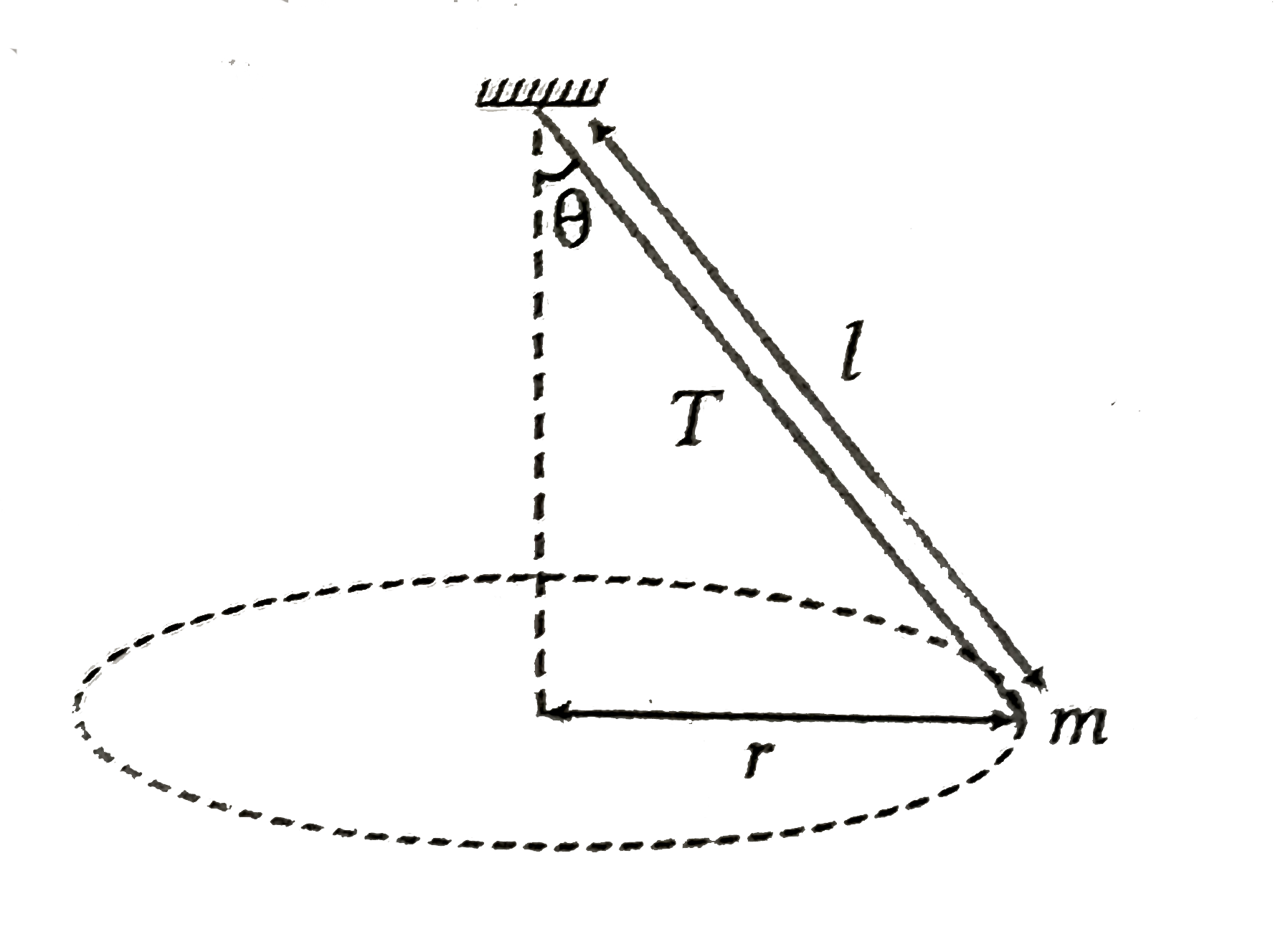
.05:11View SolutionA string of length L is fixed at one end and carries a mass M at the other end. The string makes 2/π revolution per second around the vertical axis through the fixed end as shown in the figure, then tension in the string is.
 03:05View Solution
03:05View SolutionTwo strings A and B are connected together end to end as shown in the figure. The ratio of mass per unit length (mu_(B))/(mu_(A))=4 . The tension in the string is same. A travelling wave is coming from the string A towards string B. if the fraction of the power of the incident wave that goes in sting B is (n)/(9) the value of n is:
01:17View Solution(i) The equation of wave in a string fixed at both end is y=2sinπtcosπx. Find the phase difference between oscillations of two points located at x=0.4m and x=0.6m.
(ii) A string having length L is under tension with both the ends free to move. Standing wave is set in the string and the shape of the string at time t=0 is as shown in the figure. Both ends are at extreme. The string is back in the same shape after regular intervals of time equal to T and the maximum displacement of the free ends at any instant is A. Write the equation of the standing wave.
 View Solution
View SolutionA wave pulse on a string on a string has the dimension shown in figure. The wave speed is v=1 cm /s . If point O is a free end. The shape of wave at time t = 3s si
 02:16View Solution
02:16View SolutionA string of length l fixed at one end carries a mass m at the other end. The strings makes 2πrevs−1 around the axis through the fixed end as shown in the figure, the tension in the string is
 03:53View Solution
03:53View SolutionA string of length 20 cm and linear mass density 0.40 g//cm is fixed at both ends and is kept under a tension of 16 N . A wave pulse is produced at t = 0 near an ends as shown in Fig. 7.17 (b) , which travels towards the other end . When will the string have the shape shown in the Fig . ( c).
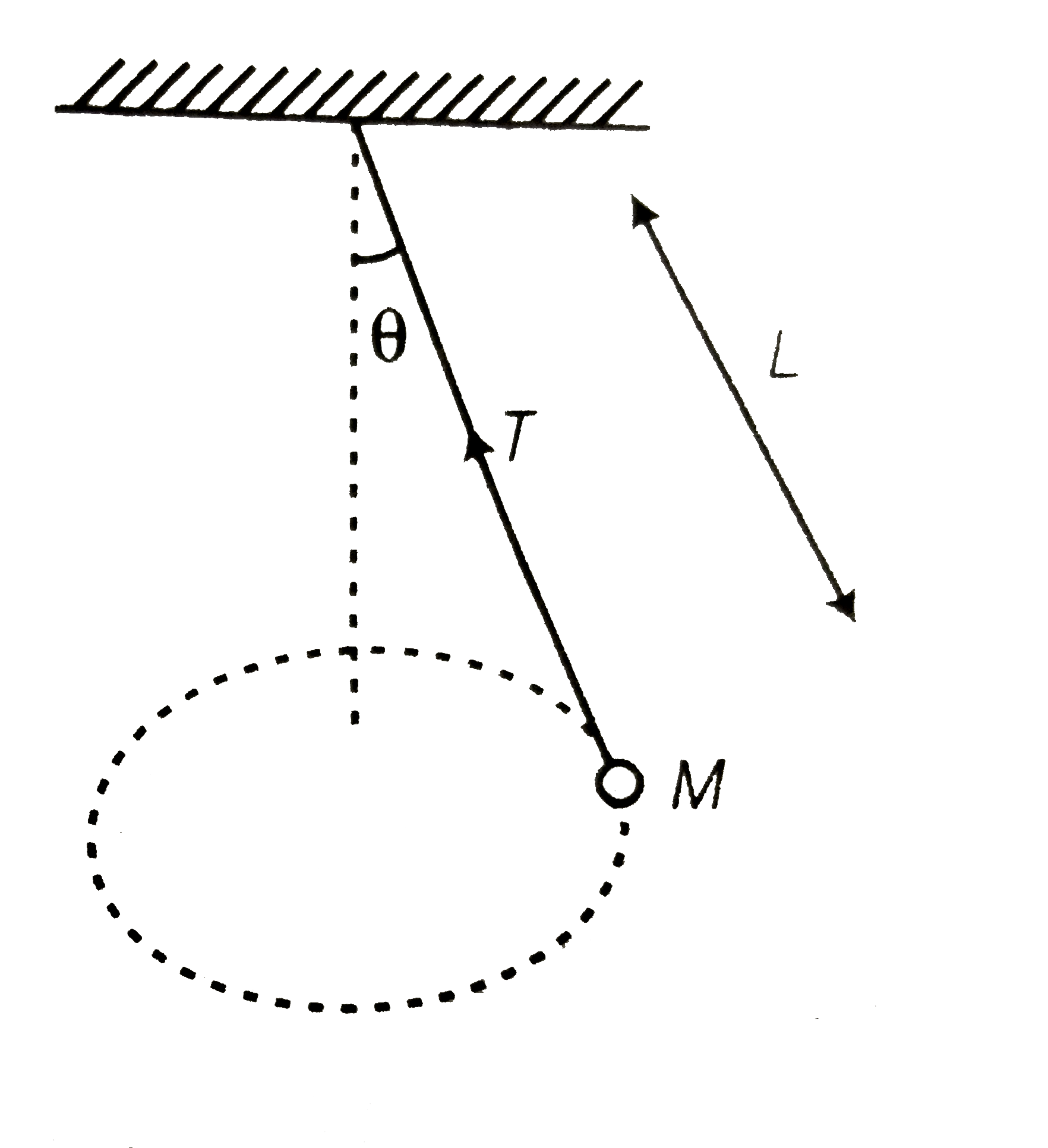
View SolutionA string of length L is fixed at one end and carries a mass M at the other end. The string makes 2π rev/s around the vertical axis through the fixed end as shown in figure, then tension in string is
 04:41View Solution
04:41View SolutionA string of linear mass density 0.5gcm−1 and a total length 30 cm is tied to a fixed wall at one end and to a frictionless ring at the other end. The ring can move on a vertical rod. A wave pulse is produced on the string which moves towards the ring at a speed of 20cms−1. The pulse is symmetric about its maximum which is located at a distance of 20 cm from the end joined to the ring. (a) Assuming that the wave is reflected from the ends without loss of energy, find the time taken by the string to regain its shape. (b) The shape of the string changes periodically with time. Find this time period. (c) What is the tension in the string ?
 03:23View Solution
03:23View SolutionA string that is 10 cm long is fixed at both ends. At t = 0, a pulse travelling from left to right at 1 cm/s is 4.0 cm from the right end as shown in figure. Determine the next two times when the pulse will be at that point again. State in each case whether the pulse is upright or inverted.
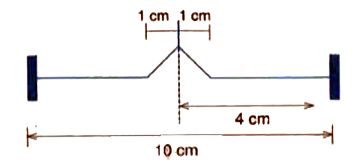 03:00View Solution
03:00View SolutionA string of length L is fixed at one end and carries a mass M at the other end . The string makes 2/π revlutions per second around the vertical axis through the fixed end as shown in the figure, then tension in the string is
02:13View Solution

