A tank of square cross section (2m×2m) is filled with water up to a height of 2.5m. Find the thrust experienced by the vertical and botom of the tank (g=10m/s2).
To solve the problem of finding the thrust experienced by the vertical and bottom surfaces of a tank filled with water, we can follow these steps:
Step 1: Calculate the thrust on the bottom of the tank
1. Identify the dimensions and parameters:
- Cross-section of the tank: 2m×2m
- Height of water: h=2.5m
- Density of water: ρ=1000kg/m3
- Acceleration due to gravity: g=10m/s2
2. Calculate the pressure at the bottom of the tank:
The pressure at the bottom due to the water column is given by:
P=ρgh
Substituting the values:
P=1000kg/m3×10m/s2×2.5m=25000Pa
3. Calculate the area of the bottom of the tank:
The area A of the bottom surface is:
A=2m×2m=4m2
4. Calculate the thrust on the bottom:
The thrust F on the bottom of the tank is given by:
F=P×A
Substituting the values:
F=25000Pa×4m2=100000N
Step 2: Calculate the thrust on the vertical walls of the tank
1. Consider a small section of the vertical wall:
- Let dx be a small height at a depth x from the top of the water.
- The pressure at this depth is:
P(x)=ρgx
2. Calculate the differential force on the small section:
The differential area dA of the vertical wall at depth x is:
dA=Ldx
where L is the width of the wall (which is 2m).
The differential force dF on this area is:
dF=P(x)dA=ρgx⋅Ldx
3. Integrate to find the total thrust on the vertical wall:
The total thrust Fv on the vertical wall from x=0 to x=2.5 m is:
Fv=∫2.50ρgxLdx
Substituting the values:
Fv=∫2.501000⋅10⋅x⋅2dx=20000∫2.50xdx
The integral of x is:
∫xdx=x22
Evaluating from 0 to 2.5:
Fv=20000⋅((2.5)22−0)=20000⋅6.252=20000⋅3.125=62500N
Final Results:
- Thrust on the bottom of the tank: 100000N
- Thrust on the vertical walls of the tank: 62500N
---
- Cross-section of the tank: 2m×2m
- Height of water: h=2.5m
- Density of water: ρ=1000kg/m3
- Acceleration due to gravity: g=10m/s2
The pressure at the bottom due to the water column is given by:
P=ρgh
Substituting the values:
P=1000kg/m3×10m/s2×2.5m=25000Pa
The area A of the bottom surface is:
A=2m×2m=4m2
The thrust F on the bottom of the tank is given by:
F=P×A
Substituting the values:
F=25000Pa×4m2=100000N
- Let dx be a small height at a depth x from the top of the water.
- The pressure at this depth is:
P(x)=ρgx
The differential area dA of the vertical wall at depth x is:
dA=Ldx
where L is the width of the wall (which is 2m).
The differential force dF on this area is:
dF=P(x)dA=ρgx⋅Ldx
The total thrust Fv on the vertical wall from x=0 to x=2.5 m is:
Fv=∫2.50ρgxLdx
Substituting the values:
Fv=∫2.501000⋅10⋅x⋅2dx=20000∫2.50xdx
The integral of x is:
∫xdx=x22
Evaluating from 0 to 2.5:
Fv=20000⋅((2.5)22−0)=20000⋅6.252=20000⋅3.125=62500N
- Thrust on the bottom of the tank: 100000N
- Thrust on the vertical walls of the tank: 62500N
Topper's Solved these Questions
- FLUID MECHANICSBOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - Solved Examples
7 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - Exercise 4.1
11 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICSCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
A tank of square cross-section of each side is filled with a liquid of height h . Find the thrust experienced by the vertical surfaces and bottom surface of the tank.
View SolutionA wooden plank of length 1m and uniform cross-section is hinged at one end of the bottom of tank as shown in figure. The tank is filled with water upto a height of 0.5m. Find the angle θ that the plank makes with the vertical in the equilibrium position. [Exclude the case thea=0]
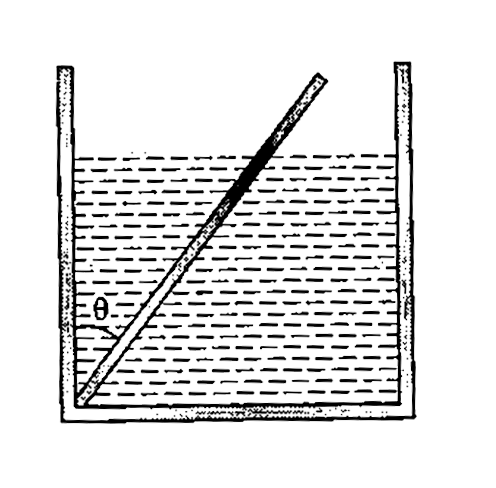
View Solution
Knowledge Check
A tank of large base area is filled with water up to a height of 5m. A hole of 2cm2 cross section in the bottom allows the water to drain out in continuous streams. For this situation, mark out the correct statement(s) (take ρwater=1000kg/m3,g=10ms−12)
 AThe cross-sectional area of the emerging stream of water decreases as it falls down.BThe cross-sectional area of the emerging stream of water increases as it falls down.CAt a distance of 5m below the bottom of the tank, the cross-sectional area of the stream is 1.414cm2.DAt a distance of 5m below the bottom of the tank, the cross-sectional area of the stream is 2.86cm2.
AThe cross-sectional area of the emerging stream of water decreases as it falls down.BThe cross-sectional area of the emerging stream of water increases as it falls down.CAt a distance of 5m below the bottom of the tank, the cross-sectional area of the stream is 1.414cm2.DAt a distance of 5m below the bottom of the tank, the cross-sectional area of the stream is 2.86cm2.A cylindrical tank having cross-sectional area A is filled with water to a height of 2.0 m. A circular hole of corss-section area is opened at a height of 75 cm from the bottom. If a/A=√0.2, then velocity with which waer emerges from the hole is
(g=9.8ms−2)A4.9ms−1B4.95ms−1C5.0ms−1D5.5ms−1A ball is projected vertically up with speed 20 m/s. Take g=10m/s2
AIt covers a distance of 5 m in 2nd second of its motionBThe displacement in 2nd and 3rd second are equalCThe distance covered in 2nd and 3rd second are equalDThe average speed for first 4 seconds is zero
The wooden plank of length 1 m and uniform cross section is hinged at one end to the bottom of a tank as shown in figure. The tank is filled with water up to a height of 0.5 m. The specific gravity of the plank is 0.5. Find the angle θ that the plank makes with the vertical in the equilibrium position. (Exclude the case θ=0)
 ,
,
View SolutionA ball is projected vertically up wards with a velocity of 100 m/s. Find the speed of the ball at half the maximum height. (g=10m/s2)
View SolutionA long cylindrical tank of cross-section area 0.5m2 is filled with water. It has an opening at a height 50 cm from the bottom, having area of cross-section 1×10−4m2. A movable piston of cross-section area almost equal to 0.5m2 is fitted on the top of the tank such that it can slide in the tank freely. A load of 20 kg is applied on the top of the water by piston, as shown in the figure. Find the speed of the water jet with which it hits the surface when piston is 1m above the bottom. (Ignore the mass of the piston). If this speed is (18x) m/s, find the value of x.
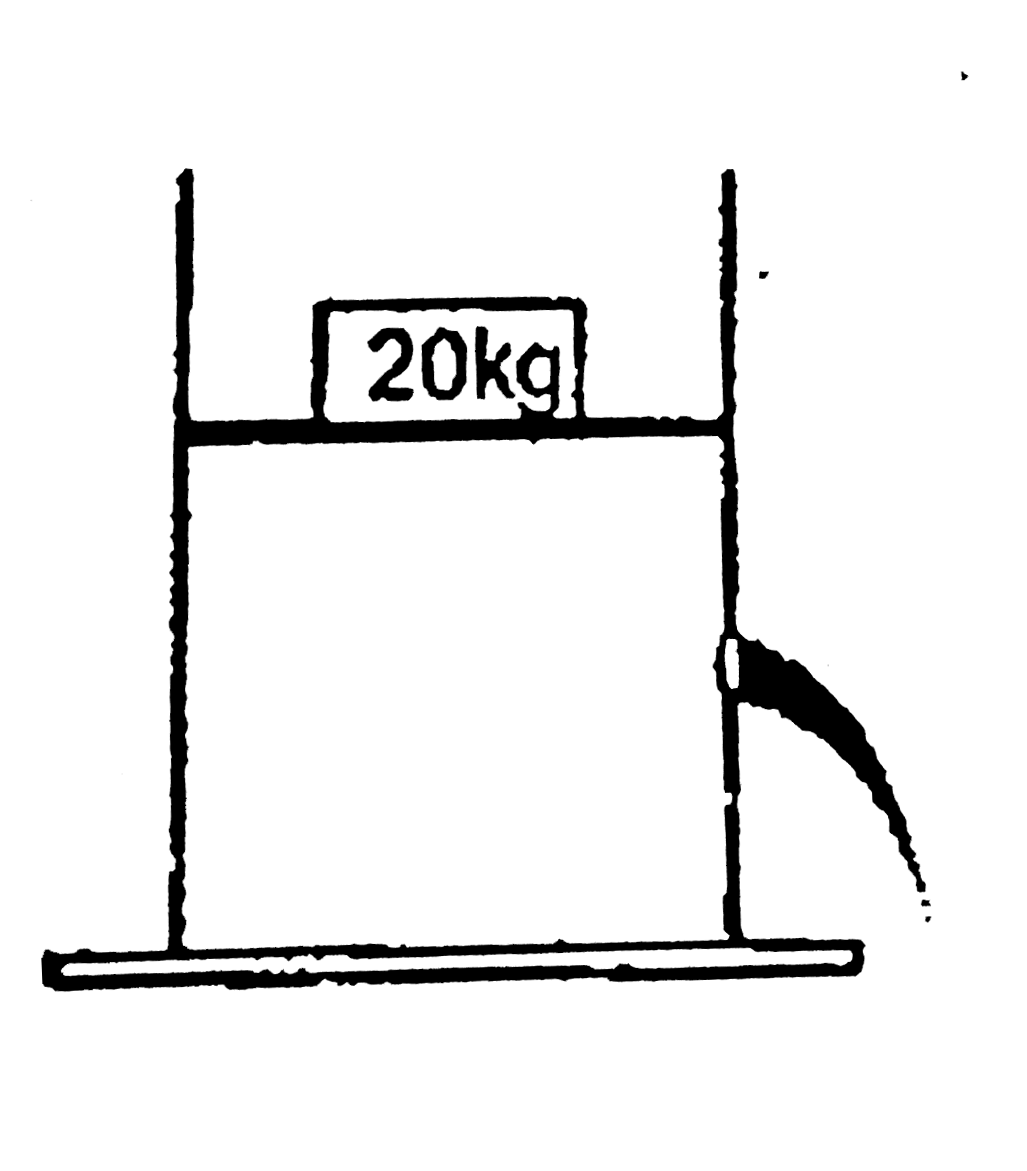
View Solution_S01_053_Q01.png)
A large cylinderical tank of cross-sectional area 1m2 is filled with water . It has a small hole at a height of 1 m from the bottom. A movable piston of mass 5 kg is fitted on the top of the tank such that it can slide in the tank freely. A load of 45 kg is applied on the top of water by piston, as shown in figure. The value of v when piston is 7 m above the bottom is (g=10m/s2)
(a). √120m/s
(b). 10m/s
(c). 1m/s
(d). 11m/s
View SolutionA tank of base area 4m2 is initially filled with water up to height 2m. An object of uniform cross-section 2m2 and height 1m is now suspended by wire into the tank, keeping distance between base of tank and that of object 1m. Density of the object is 2000kg/m3. Take atmospheric pressure 1×105N/m2,g=10m/s2.
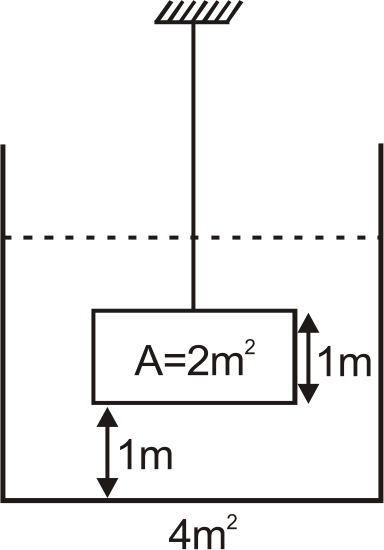
The downwards force exerted by the water on the top surface of the object is
View Solution
