(A): The electric field induced due to changing magnetic field is non-conservative.
(R): The line integral of the electric field along a closed loop is always zero.
Both A and R are true and R is the correct explanation
Both A and R are true but R is not the correct explanation of A
A is true but R is false
Both A and R are false
The correct Answer is:C
To solve the question, we need to analyze the statements given:
Statement (A): The electric field induced due to changing magnetic field is non-conservative.
Statement (R): The line integral of the electric field along a closed loop is always zero.
Step 1: Understanding the Nature of Induced Electric Fields
The electric field induced by a changing magnetic field is indeed non-conservative. This is a fundamental concept in electromagnetism, specifically described by Faraday's law of electromagnetic induction. A non-conservative electric field means that the work done in moving a charge around a closed path in this field is not zero.
Hint: Recall Faraday's law of electromagnetic induction, which states that a changing magnetic field induces an electric field.
Step 2: Evaluating the Line Integral of the Induced Electric Field
For a non-conservative electric field, the line integral of the electric field around a closed loop is not zero. According to Faraday's law, the induced electromotive force (emf) in a closed loop is equal to the negative rate of change of magnetic flux through the loop. This implies that the line integral of the electric field around a closed path is equal to the induced emf, which is generally not zero if there is a changing magnetic field.
Hint: Consider the implications of Faraday's law and how it relates to the work done in a closed loop.
Step 3: Conclusion
From our analysis:
- Statement (A) is true: The electric field induced due to a changing magnetic field is non-conservative.
- Statement (R) is false: The line integral of the electric field along a closed loop is not always zero; it is non-zero in the presence of a changing magnetic field.
Thus, the correct answer is that (A) is true and (R) is false.
Final Answer: (A) is true, (R) is false.
Statement (R): The line integral of the electric field along a closed loop is always zero.
The electric field induced by a changing magnetic field is indeed non-conservative. This is a fundamental concept in electromagnetism, specifically described by Faraday's law of electromagnetic induction. A non-conservative electric field means that the work done in moving a charge around a closed path in this field is not zero.
For a non-conservative electric field, the line integral of the electric field around a closed loop is not zero. According to Faraday's law, the induced electromotive force (emf) in a closed loop is equal to the negative rate of change of magnetic flux through the loop. This implies that the line integral of the electric field around a closed path is equal to the induced emf, which is generally not zero if there is a changing magnetic field.
From our analysis:
- Statement (A) is true: The electric field induced due to a changing magnetic field is non-conservative.
- Statement (R) is false: The line integral of the electric field along a closed loop is not always zero; it is non-zero in the presence of a changing magnetic field.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE-II
32 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE-III
29 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE-IA
58 Videos
- ELECTRIC FIELD AND POTENTIALBOOK - AAKASH SERIESCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - PROBLEMS (LEVEL-II)
26 Videos
- ELECTROMAGNETIC WAVESBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - EXERCISE -II
22 Videos
Similar Questions
Electric field induced by changing magnetic fields are
View SolutionA: The electric field induced due to changing magnetic field is non-conservative,
R: The line integral of the electric field induced due to changing magnetic field along a closed loop is always zero.
View Solution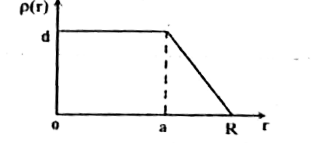
The electric field at r= R is
View SolutionIf the flux of the electric field through a closed surface is zero,
View SolutionStatement 1: Electric field produced by changing magnetic field is nonconservative.
Statement 2: For the electric field →E induced by a changing magnetic field which has closed lines of force, ∮→E.→dl=0
View SolutionElectric Field due to Dipole
View SolutionFor the following electric field lines, the the electric field is
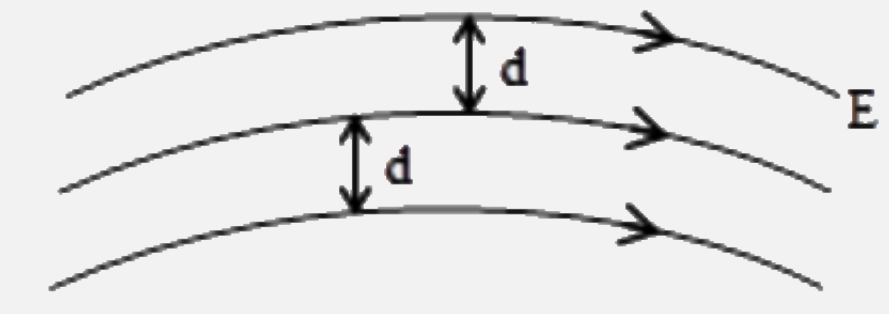
View SolutionThe unit of physical quantity obtained by the line integral of electric field is
View SolutionThe electric and magnetic field of an electromagnetic wave is
View SolutionAssertion : An emf →E is induced in a closed loop where magnetic flux is varied. The induced →E is not a conservative field.
Reason : The line intergral →E.→dl around the closed loop is non-zero.
View Solution
