A metal sphere with its centre at A and radius R has a charge 2q on it. The field at a point B outside the sphere is E. If another metal sphere of radius 3R and having a charge -3q is placed with its centre at point B, find out the resultant electric field at a point mid way between A and B.
The correct Answer is:10E
To find the resultant electric field at a point midway between two metal spheres with given charges, we can follow these steps:
Step 1: Understand the setup
We have two metal spheres:
- Sphere 1 (centered at point A) has a charge of 2q and radius R.
- Sphere 2 (centered at point B) has a charge of −3q and radius 3R.
We need to find the electric field at point C, which is midway between points A and B.
Step 2: Determine the distance from A to B
Let the distance between the centers A and B be d. Since point C is midway, the distance from A to C is d/2 and the distance from B to C is also d/2.
Step 3: Calculate the electric field due to Sphere 1 at point C
The electric field EA due to Sphere 1 at point C can be calculated using the formula for the electric field due to a point charge:
EA=k⋅Qr2
where Q=2q and r=d/2. Thus,
EA=k⋅2q(d/2)2=k⋅2qd2/4=8kqd2
Step 4: Calculate the electric field due to Sphere 2 at point C
The electric field EB due to Sphere 2 at point C is given by:
EB=k⋅Qr2
where Q=−3q and r=d/2. Thus,
EB=k⋅(−3q)(d/2)2=k⋅(−3q)d2/4=−12kqd2
Step 5: Determine the resultant electric field at point C
The resultant electric field EC at point C is the vector sum of EA and EB:
EC=EA+EB=8kqd2+−12kqd2=−4kqd2
Step 6: Conclusion
The resultant electric field at point C is:
EC=−4kqd2
This indicates that the electric field at point C is directed towards Sphere 2, due to the negative charge on it.
---
We have two metal spheres:
- Sphere 1 (centered at point A) has a charge of 2q and radius R.
- Sphere 2 (centered at point B) has a charge of −3q and radius 3R.
Let the distance between the centers A and B be d. Since point C is midway, the distance from A to C is d/2 and the distance from B to C is also d/2.
The electric field EA due to Sphere 1 at point C can be calculated using the formula for the electric field due to a point charge:
EA=k⋅Qr2
where Q=2q and r=d/2. Thus,
EA=k⋅2q(d/2)2=k⋅2qd2/4=8kqd2
The electric field EB due to Sphere 2 at point C is given by:
EB=k⋅Qr2
where Q=−3q and r=d/2. Thus,
EB=k⋅(−3q)(d/2)2=k⋅(−3q)d2/4=−12kqd2
The resultant electric field EC at point C is the vector sum of EA and EB:
EC=EA+EB=8kqd2+−12kqd2=−4kqd2
The resultant electric field at point C is:
EC=−4kqd2
This indicates that the electric field at point C is directed towards Sphere 2, due to the negative charge on it.
Topper's Solved these Questions
- ELECTRIC FIELD AND POTENTIALBOOK - AAKASH SERIESCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - PROBLEMS (LEVEL-I)
13 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - AAKASH SERIESCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Practice Exercise
57 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Additional Exercise
11 Videos
Similar Questions
Knowledge Check
Metallic sphere of radius R is charged to potential V. Then charge q is proportional to
AVBRCboth V and RDnone of these
A hallow metal sphere of radius R is uniformly charged. The electric field due to the sphere at a distance r from the centre:
View SolutionA conducting sphere of radius R is given a charge Q. The electric potential and the electric field at the centre of the sphere respectively are
View SolutionA conducting sphere of radius R is given a charge Q. The electric potential and the electric field at the centre of the sphere respectively are
View SolutionFlux passing through the shaded surface of a sphere when a point charge q is placed at the center is (radius of the sphere is R)
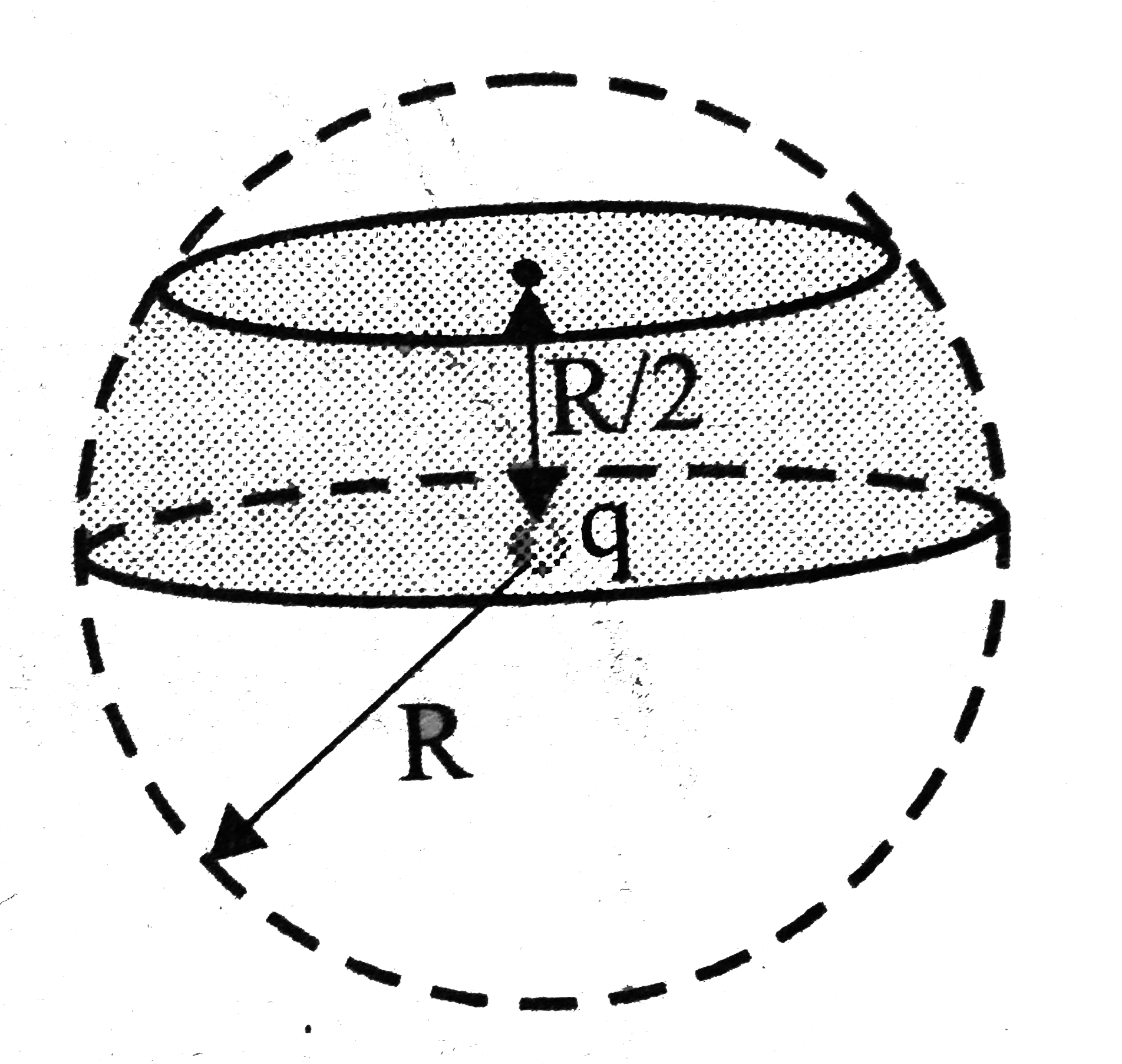
View SolutionA hollow metal sphere of radius 5cm is charged so that the potential on its surface is 10V. The potential at the centre of the sphere is
View SolutionFigure shows a uniformly charged thin non-conducting sphere of total charge Q and radius R. If point charge q is situated at point 'A' which is at a distance r<R from centre of the sphere, then find out following :
(i) Force acting on charge q.
(ii) Electric field at centre of sphere.
(iii) Electric field at point B.

View SolutionA conducting sphere of radius R is charged to a potential of V volts. Then the electric field at a distance r(>R) from the centre of the sphere would be
View Solution
