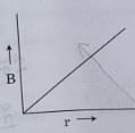
A thick current carrying cable of radius 'R' carries current 'I' uniformly distributed across its crosssection. The variation of magnetic field B(r) due to cable with distance 'r' from the axis of the cable is represented by.
To solve the problem of the variation of the magnetic field B(r) due to a thick current-carrying cable of radius R that carries a current I uniformly distributed across its cross-section, we can use Ampère's Law. Here’s a step-by-step solution:
Step 1: Understand the Configuration
We have a thick cylindrical cable of radius R carrying a current I uniformly distributed across its cross-section. We need to analyze the magnetic field B(r) at a distance r from the axis of the cable.
Step 2: Apply Ampère's Law
Ampère's Law states that:
∮→B⋅d→l=μ0Ienc
where Ienc is the current enclosed by the Amperian loop.
Step 3: Case 1 - Inside the Cable (r<R)
For points inside the cable (where r<R):
- The current density J is given by:
J=IπR2
- The enclosed current Ienc for a radius r is:
Ienc=J⋅A=J⋅πr2=IπR2⋅πr2=Ir2R2
- Now, applying Ampère's Law:
B(2πr)=μ0Ienc⟹B(2πr)=μ0(Ir2R2)
- Therefore, the magnetic field inside the cable is:
B(r)=μ0Ir2πR2
Step 4: Case 2 - Outside the Cable (r≥R)
For points outside the cable (where r≥R):
- The entire current I is enclosed:
Ienc=I
- Applying Ampère's Law:
B(2πr)=μ0I⟹B(2πr)=μ0I
- Therefore, the magnetic field outside the cable is:
B(r)=μ0I2πr
Step 5: Summary of Results
- For r<R:
B(r)=μ0Ir2πR2
- For r≥R:
B(r)=μ0I2πr
Step 6: Graphical Representation
- The magnetic field B(r) increases linearly with r for r<R and decreases hyperbolically for r≥R.
Conclusion
The variation of the magnetic field B(r) with distance r from the axis of the cable can be represented as:
- A linear increase for r<R
- A hyperbolic decrease for r≥R
We have a thick cylindrical cable of radius R carrying a current I uniformly distributed across its cross-section. We need to analyze the magnetic field B(r) at a distance r from the axis of the cable.
Ampère's Law states that:
∮→B⋅d→l=μ0Ienc
where Ienc is the current enclosed by the Amperian loop.
For points inside the cable (where r<R):
- The current density J is given by:
J=IπR2
- The enclosed current Ienc for a radius r is:
Ienc=J⋅A=J⋅πr2=IπR2⋅πr2=Ir2R2
- Now, applying Ampère's Law:
B(2πr)=μ0Ienc⟹B(2πr)=μ0(Ir2R2)
- Therefore, the magnetic field inside the cable is:
B(r)=μ0Ir2πR2
For points outside the cable (where r≥R):
- The entire current I is enclosed:
Ienc=I
- Applying Ampère's Law:
B(2πr)=μ0I⟹B(2πr)=μ0I
- Therefore, the magnetic field outside the cable is:
B(r)=μ0I2πr
- For r<R:
B(r)=μ0Ir2πR2
- For r≥R:
B(r)=μ0I2πr
- The magnetic field B(r) increases linearly with r for r<R and decreases hyperbolically for r≥R.
The variation of the magnetic field B(r) with distance r from the axis of the cable can be represented as:
- A linear increase for r<R
- A hyperbolic decrease for r≥R
Topper's Solved these Questions
Similar Questions
Knowledge Check
A long straight wire of radius 10mm carries a current uniformly distributed over its cross section. The ration of magnetic fields due to the wire at distance of 5mm and 15 mm, respectively from the axis of the wire is
A34B23C13D2A cylindrical long wire of radius R carries a current I uniformly distributed over the cross sectional area of the wire. The magnetic field at a distance x from the surface inside the wire is
Aμ0I2π(R−x)Bμ0I2πxCμ0I2π(R+x)Dnone of theseA long straight wire of radius a carries a steady current i. The current is uniformly distributed across its cross section. The ratio of the magnetis field at (a)/(2)and(2a) is
A(1/2)B(1/4)C4D1
A long, cylindrical wire of radius b carries a current i distributed uniformly over its cross section. Find the magnitude of the magnetic field at a point inside the wire at a distance a from the axis.
View SolutionA long straight solid metal wire of radius R carries a current I, uniformly distributed over its circular cross-section. Find the magnetic field at a distance r from axis of wire (i) inside and (ii) outside the wire.
View SolutionA long staright wire of radius a carries a steady current I. The curent is unifromly distributed over its cross-section. The ratio of the magnetic fields B and B' , at radial distances a2 and 2a respectively from the axis of the wire is:
View SolutionA long straight wire of radius a carries a steady current I. The current is uniformly distributed over its cross-section. The ratio of the inagnetic fields B and B', at radial distances a2 and 2a respectively, from the axis of the wire is
View SolutionA long, thick straight conductor of radius R carries current I uniformly distributed in its cross section area.The ratio of energy density of the magnetic field at distance R/2 from surface inside the conductor and outside the conductor is:
View Solution
