A thin uniform disc (see figure) of mass M has outer radius 4R and inner radius 3R. The work required to take a unit mass for point P on its axis to infinity is
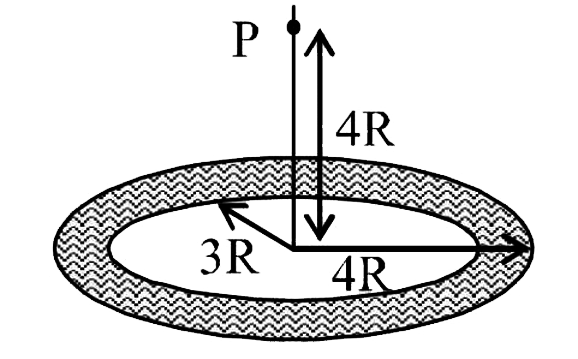
2GM7R(4√2−5)
−2GM7R(4√2−5)
GM4R
2GM5R(√2−1)
The correct Answer is:A
Consider an element of the disc of radius x and thichness dx. Mass of the element is
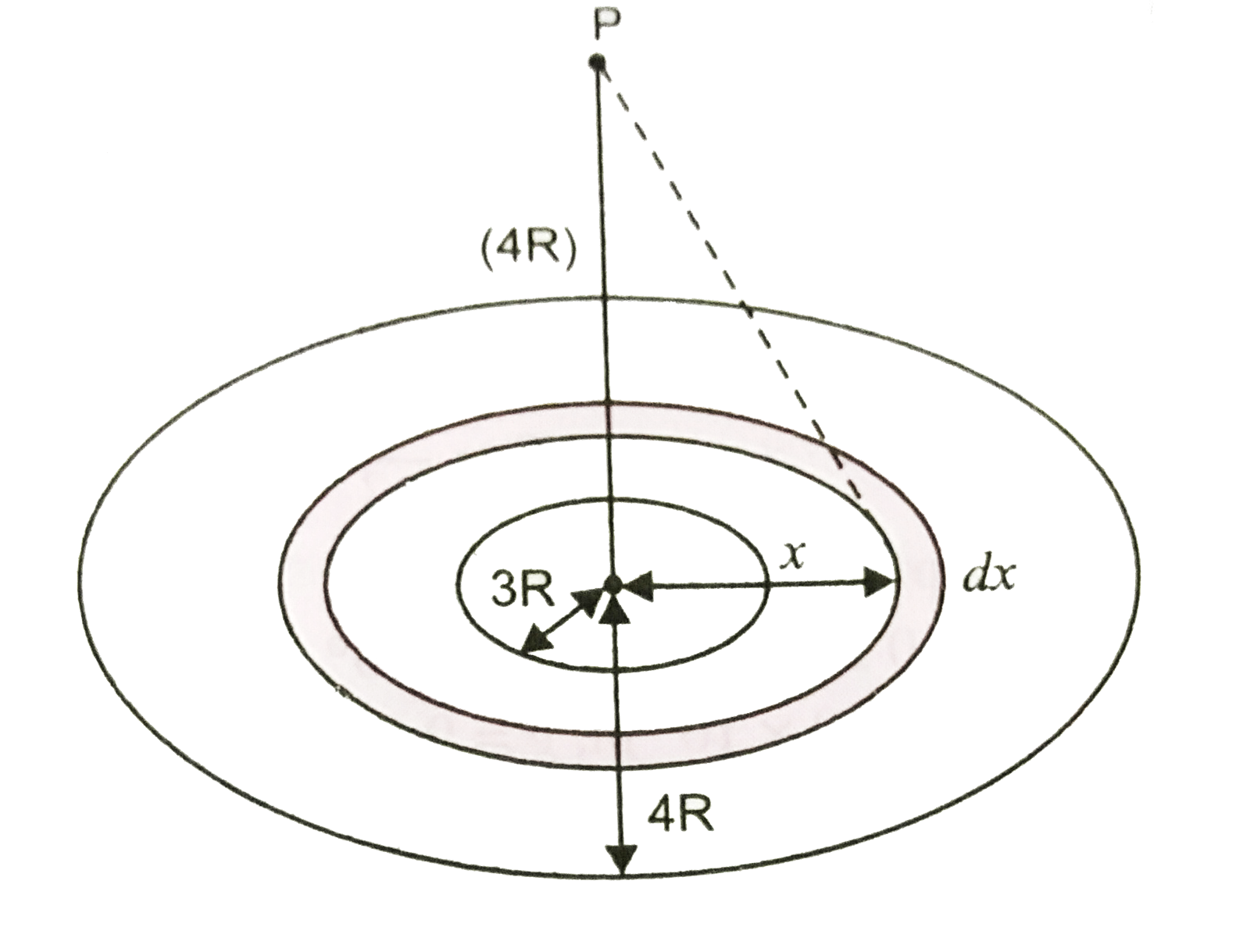
dM=M×2πxdx[π(4R)2−π(3R)2]=2M7R2xdx
Gravitational potential at P due to element of the
disc dV=−G(2M7R2xdx)×1√x2+(4R)2
Workdone in taking a unit mass from unit P to infinity is
W=−V=+∫4R3RG2M7R2xdx1√x2+(4R)2
=2GM7R2∫4R3Rxdx[x2+(4R)2]1/2
Let √x2+(4R)2=z
Differenting it, we have
12[x2+(4R)2]−1/2×2xdx=dz
or xdx[x2+(4R)2]2=dz
When, x=3R
z=√(3R)2+(4R)2=5R
When, x=4R
z=√(4R)2+(4R)2=4√2R
∴W=2GM7R2∫4√2R5Rdz=2GM7R2[z]4√2R5R
=2GM7R2[4√2R−5R]=2GM7R[4√2−5]
Similar Questions

A uniform disc of mass M and radius R is pivoted about the horizontal axis through its centre C A point mass m is glued to the disc at its rim, as shown in figure. If the system is released from rest, find the angular velocity of the disc when m reaches the bottom point B.
View SolutionA thin uniform circular disc of mass M and radius R is rotating in a horizontal plane about an axis passing through its centre and perpendicular to its plane with an angular velocity ω . Another disc of same dimensions but of mass 14 M is placed gently on the first disc co-axially. The angular velocity of the system is
View Solution
Knowledge Check
The energy required to move a body of massm from an orbit of radius 3R to 4R is
AGMm2RBGMm6RCGMm12RDGMm24RFor a uniform ring of mass M and radius R at its centre
Afield and potential both are zeroBfield is zero but potential is GMRCfield is zero but potential is −GM/RDmagnitude of field is GMR2 and potential −GMRA thin annular disc of outer radius R and R2 inner radius has charge Q uniformly distributed over its surface. The disc rotates about its axis with a constant angular velocity ω. Choose the correct option(s):
AThe magnetic field intensity at the centre of the disc is μ0Qω3πRBThe magnetic field intensity at the centre of the disc is μ0Qω4RCThe magnetic moment of the disc is 5QωR216DThe magnetic moment of the disc is 3QωR24
A thin uniform circular disc of mass M and radius R is rotating in a horizontal plane about an axis passing through its centre and perpendicular to its plane with an angular velocity ω. Another disc of same dimensions but of mass M/4 is placed gently on the first disc coaxially. The angular velocity of the system now is
View SolutionThe moment of inertia of an annular disc of mass M, outer and inner radii R and r, about its diameter is
View SolutionThe moment of inertia of an annular disc of mass M, outer and inner radii R and r, about its diameter is
View SolutionConsider a thin spherical shell of uniform density of mass M and radius R:
View SolutionA thin uniform circular disc of mass M and radius R is rotating in a horizontal plane about an axis perpendicular to the plane at an angular velocity ω . Another disc of mass M / 3 but same radius is placed gently on the first disc coaxially . the angular velocity of the system now is
View Solution
