A tuning fork of frequency 256hZ is moving towards a well with a velocity of 5m/s . If the speed of sound is 330m/s , then the number of beats heard per second by a stationary observer lying between tuning fork and the well is
2
4
zero
8
The correct Answer is:C
To solve the problem step by step, we will use the concept of the Doppler effect to find the frequency heard by the observer and then determine the number of beats.
Step 1: Identify the given values
- Frequency of the tuning fork (source), f=256Hz
- Velocity of the tuning fork (source), vs=5m/s (moving towards the wall)
- Speed of sound in air, v=330m/s
Step 2: Calculate the frequency heard by the observer (Doppler effect)
Since the tuning fork is moving towards the wall and the observer is stationary, we can use the Doppler effect formula for a moving source and a stationary observer:
f′=f(vv−vs)
Substituting the values:
f′=256(330330−5)
Calculating the denominator:
330−5=325
Now substituting back:
f′=256(330325)
Calculating 330325:
330325≈1.0154
Now calculating f′:
f′≈256×1.0154≈260Hz
Step 3: Frequency of the sound reflected back from the wall
The wall acts as a stationary observer reflecting the sound back. The frequency of the sound reflected back to the observer is the same as the frequency heard by the wall:
f′′=f′=260Hz
Step 4: Calculate the beats heard by the observer
The number of beats heard by the observer is the difference between the frequency of the sound heard by the observer and the frequency of the sound reflected back from the wall. Since both frequencies are equal:
Beats=|f′−f′′|=|260−260|=0Hz
Final Answer
The number of beats heard per second by the stationary observer is 0Hz.
---
- Frequency of the tuning fork (source), f=256Hz
- Velocity of the tuning fork (source), vs=5m/s (moving towards the wall)
- Speed of sound in air, v=330m/s
Since the tuning fork is moving towards the wall and the observer is stationary, we can use the Doppler effect formula for a moving source and a stationary observer:
The wall acts as a stationary observer reflecting the sound back. The frequency of the sound reflected back to the observer is the same as the frequency heard by the wall:
The number of beats heard by the observer is the difference between the frequency of the sound heard by the observer and the frequency of the sound reflected back from the wall. Since both frequencies are equal:
The number of beats heard per second by the stationary observer is 0Hz.
Topper's Solved these Questions
- SOUND WAVESBOOK - DC PANDEY ENGLISHCHAPTER - SOUND WAVESEXERCISE - Level 1 Subjective
45 Videos
- SOUND WAVESBOOK - DC PANDEY ENGLISHCHAPTER - SOUND WAVESEXERCISE - Subjective Questions
1 Videos
- SOUND WAVESBOOK - DC PANDEY ENGLISHCHAPTER - SOUND WAVESEXERCISE - Level 1 Assertion And Reason
10 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)
38 Videos
- SUPERPOSITION OF WAVESBOOK - DC PANDEY ENGLISHCHAPTER - SUPERPOSITION OF WAVESEXERCISE - Level 2 Subjective
8 Videos
Similar Questions
A source of sound of frequency 500 Hz is moving towards an observer with velocity 30 m/s . The speed of sound is 330 m/s . the frequency heard by the observer will be
View SolutionA person with vibrating tuning fork of frequency 338 Hz is moving towards a vertical wall with speed of 2ms−1 Velocity of sound in air is 340ms−1 The number of beats heard per second is
View Solution
As shown if Fig. a vibrating tuning fork of frequency 512 Hz is moving towards the wall with a speed 2ms. Take speed of sound as v=340ms and answer the following questions.
Q. Suppose that a listener is located at rest between the tuning fork and the wall. Number of beats heard by the listener per second will be
View Solution
As shown if Fig. a vibrating tuning fork of frequency 512 Hz is moving towards the wall with a speed 2ms. Take speed of sound as v=340ms and answer the following questions.
Q. Suppose that a listener is located at rest between the tuning fork and the wall. Number of beats heard by the listener per second will be
View Solution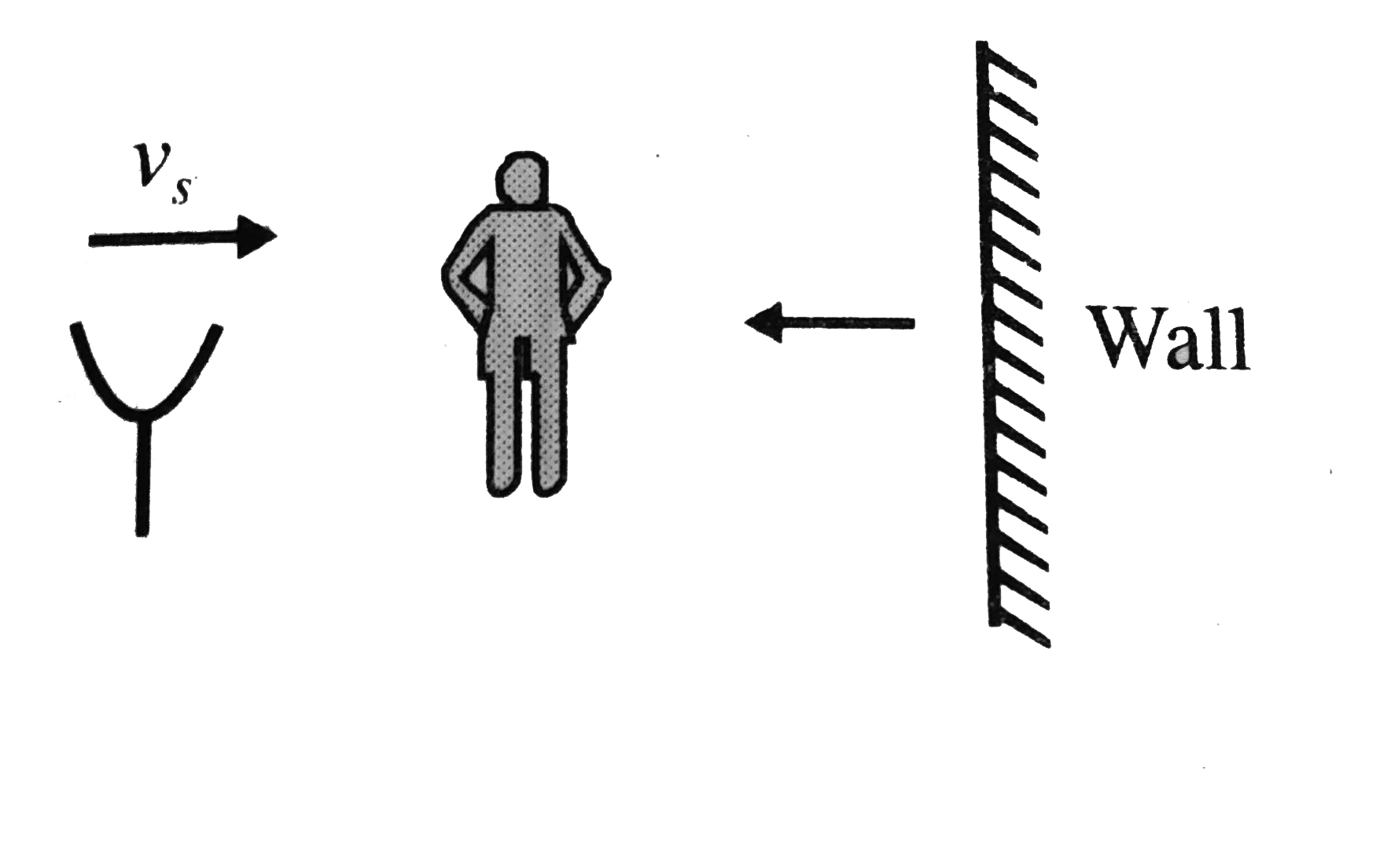
A tuning fork of frequency 380 Hz is moving towards a wall with a velocity of 4ms Then the number of beats heard by a stationary listener between direct and reflected sound will be (velocity of sound in air is 340ms)
View Solutiona bus is moving with a velocity 4 m/s towards a wall, the driver sounds a horn of frequency 173 Hz . if the speed of sound in air is 350 m/s. the number of beat heard per second by a passanger on the bus will be
View SolutionA source of sound of frequency 256Hz is moving rapidly towards wall with a velocity of 5m/sec. How many beats per second will be heard if sound travels at a speed of 330m/sec.
View Solution
As shown if Fig. a vibrating tuning fork of frequency 512 Hz is moving towards the wall with a speed 2ms. Take speed of sound as v=340ms and answer the following questions.
Q. If the listener is at rest and located such that the tuning fork is moving between the listener and the wall, number of beats heard by the listerner per second will be nearly
View Solution
As shown if Fig. a vibrating tuning fork of frequency 512 Hz is moving towards the wall with a speed 2ms. Take speed of sound as v=340ms and answer the following questions.
Q. If the listener, along with the source, is moving towards the wall with the same speed i.e., 2ms, such that the source remains between the listerner and the wall, number of beats heard by the listerner per second will be
View Solution
As shown if Fig. a vibrating tuning fork of frequency 512 Hz is moving towards the wall with a speed 2ms. Take speed of sound as v=340ms and answer the following questions.
Q. If the listener, along with the source, is moving towards the wall with the same speed i.e., 2ms, such that the source remains between the listerner and the wall, number of beats heard by the listerner per second will be
View Solution
