A tunnel is dug in the earth across one of its diameter. Two masses and are dropped from the two ends of the tunel. The masses collide and stick each other. They perform , the ampulitude of which is radius of earth)
The correct Answer is:C
To solve the problem step by step, we will analyze the situation where two masses, m and 2m, are dropped from the ends of a tunnel dug through the Earth. They collide and stick together, performing simple harmonic motion (SHM). The amplitude of this motion is given as R, where R is the radius of the Earth.
Step 1: Understand the System
- We have two masses: m and 2m.
- They are dropped from opposite ends of a tunnel that goes through the diameter of the Earth.
- When they collide, they stick together and perform SHM.
Hint: Visualize the Earth and the tunnel. Consider how gravitational force acts on the masses as they fall.
Step 2: Conservation of Energy
- For mass m:
- Initial potential energy, Ei1=−GmMR (where M is the mass of the Earth).
- Final potential energy at the center (where kinetic energy is maximum), Ef1=0.
- For mass 2m:
- Initial potential energy, Ei2=−G(2m)MR.
- Final potential energy at the center, Ef2=0.
Hint: Use the gravitational potential energy formula and remember that potential energy is negative when considering gravitational attraction.
Step 3: Calculate Velocities at the Center
- Using conservation of energy for mass m:
Ei1=Ef1⟹−GmMR=0+12mv21
Rearranging gives:
12mv21=GmMR⟹v21=2GMR
- For mass 2m:
Ei2=Ef2⟹−G(2m)MR=0+12(2m)v22
Rearranging gives:
12(2m)v22=G(2m)MR⟹v22=2GMR
Hint: Both masses will have the same speed when they meet at the center due to symmetry.
Step 4: Conservation of Momentum
- Before the collision, the momentum is:
pinitial=mv1−2mv2
- After the collision, the combined mass moves with velocity v′:
pfinal=(m+2m)v′=3mv′
- Setting initial momentum equal to final momentum:
mv1−2mv2=3mv′
Since v1=v2:
mv1−2mv1=3mv′⟹−mv1=3mv′⟹v′=−v13
Hint: Remember that momentum is conserved in collisions.
Step 5: Energy Conservation After Collision
- The total energy after the collision is the sum of kinetic and potential energy:
Einitial=−3GMR+12(3m)v′2
Substitute v′:
Efinal=−3GMR+12(3m)(−v13)2
Hint: Use the kinetic energy formula and substitute the expression for v′.
Step 6: Determine Amplitude of SHM
- The amplitude of the SHM is determined from the maximum displacement from the equilibrium position, which is R/3.
Final Answer: The amplitude of the SHM performed by the two masses after colliding is R3.
- We have two masses: m and 2m.
- They are dropped from opposite ends of a tunnel that goes through the diameter of the Earth.
- When they collide, they stick together and perform SHM.
- For mass m:
- Initial potential energy, Ei1=−GmMR (where M is the mass of the Earth).
- Final potential energy at the center (where kinetic energy is maximum), Ef1=0.
- For mass 2m:
- Initial potential energy, Ei2=−G(2m)MR.
- Final potential energy at the center, Ef2=0.
- Using conservation of energy for mass m:
Ei1=Ef1⟹−GmMR=0+12mv21
Rearranging gives:
12mv21=GmMR⟹v21=2GMR
Ei2=Ef2⟹−G(2m)MR=0+12(2m)v22
Rearranging gives:
12(2m)v22=G(2m)MR⟹v22=2GMR
- Before the collision, the momentum is:
pinitial=mv1−2mv2
- After the collision, the combined mass moves with velocity v′:
pfinal=(m+2m)v′=3mv′
- Setting initial momentum equal to final momentum:
mv1−2mv2=3mv′
Since v1=v2:
mv1−2mv1=3mv′⟹−mv1=3mv′⟹v′=−v13
- The total energy after the collision is the sum of kinetic and potential energy:
Einitial=−3GMR+12(3m)v′2
Substitute v′:
Efinal=−3GMR+12(3m)(−v13)2
- The amplitude of the SHM is determined from the maximum displacement from the equilibrium position, which is R/3.
Topper's Solved these Questions
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - Level 2 More Than One Correct
10 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - Level 2 Subjective
14 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - Level 1 Subjective
19 Videos
- GENERAL PHYSICSBOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE
10 Videos
Similar Questions
A straight tuning is due into the earth as shows in figure at a distance b from its centre. A ball of mass m is dropped from one of its ends. The time it takes to each the other end is approximately

View SolutionA tunnel is dug across the diameter of earth. A ball is released from the surface of Earth into the tunnel. The velocity of ball when it is at a distance R2 from centre of earth is (where R = radius of Earth and M = mass of Earth)
View SolutionA tunnel is dug inside the earth across one of its diameters. Radius of earth is R and its mass is M. A particle is projected inside the tunnel with velocity √2GMR from one of its ends then maximum velocit attained by the particle in the subsequent motion is √nGMR (assuming tunnel to be frictionless). Find n
View SolutionA tunnel is dug inside the earth across one of its diameters. Radius of earth is R and its mass is M. A particle is projected inside the tunnel with velocity √2GMR from one of its ends then maximum velocity attained by the particle in the subsequent motion is (assuming tunnel to be frictionless)
View SolutionA tuning is dug along the diameter of the earth. There is particle of mass m at the centre of the tunel. Find the minimum velocity given to the particle so that is just reaches to the surface of the earth. (R= radius of earth)
View SolutionIf body of mass mhas to be taken from the surface to the earth to a height h=4R, then the amount of energy required is (R = radius of the earth)
View SolutionA tunnel is dug along a diameter of the earth. Find the force in on a particle of mass m placed in the tunnel at a distance x from the centre.
View SolutionA ball of mass m is dropped from a height h equal to the radius of the earth above the tunnel dug through the earth as shows in the figure. Choose the correct options. (Mass of earth =M)
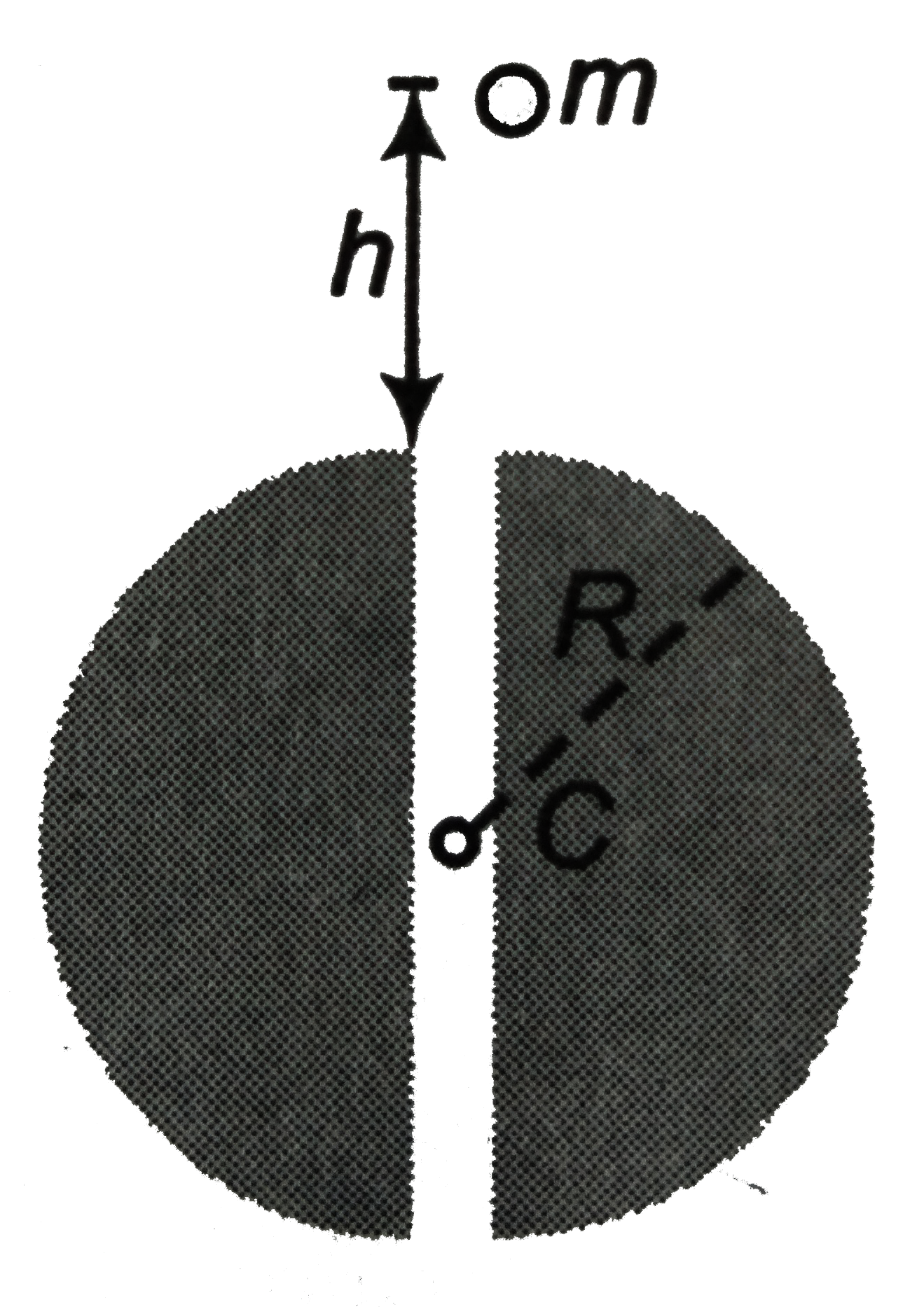
View SolutionTwo smooth tunnels are dug from one side of earth's surface to the other side, one along a diameter are dropped from one end of each of the tunnels. Both particles oscillate simple harmonically along the tunnels. Let T1 and T2 be the time period of particles along the diameter and along the chord respectively. Then:
View Solutiona narrow tunnel is dug along the diameter of the earth, and a particle of mass m0 is placed at R2 distance from the centre. Find the escape speed of the particle from that place.

View Solution
