a uniform beam, of length L and mass m = 1.8 kg, is at rest on two scales. A uniform block, with mass M = 2.7 kg, is at rest on the beam, with its center a distance L/4 from the beam's left end. What do the scales read?
To solve the problem of finding the readings on the two scales when a uniform beam and a uniform block are at rest, we can follow these steps:
Step 1: Identify the Forces Acting on the System
- The beam has a mass m=1.8kg, so its weight Wb=mg=1.8×9.8N.
- The block has a mass M=2.7kg, so its weight Wb=Mg=2.7×9.8N.
- The beam is supported by two scales, which we will denote as R1 (left scale) and R2 (right scale).
Step 2: Calculate the Total Weight
- The total weight acting downwards on the system is:
Wtotal=Wb+WM=(1.8×9.8)+(2.7×9.8)=15.435N+26.46N=41.895N
Step 3: Set Up the Equilibrium Conditions
Since the beam is at rest, we can apply the conditions for static equilibrium:
1. The sum of vertical forces must equal zero:
R1+R2=Wtotal
R1+R2=41.895N(1)
2. The sum of torques about any point must equal zero. We will take torques about the left end of the beam (where scale 1 is located):
- The torque due to R2 (acting at distance L) is counterclockwise and is given by R2⋅L.
- The torque due to the weight of the beam (acting at L/2) is clockwise and is given by Wb⋅(L/2).
- The torque due to the weight of the block (acting at L/4) is also clockwise and is given by WM⋅(L/4).
Setting the sum of torques to zero:
R2⋅L−Wb⋅L2−WM⋅L4=0
Dividing through by L (since L≠0):
R2−Wb2−WM4=0
Substituting the weights:
R2=(1.8×9.8)2+(2.7×9.8)4(2)
Step 4: Calculate R2
Calculating the individual components:
- Wb=1.8×9.8=17.64N
- WM=2.7×9.8=26.46N
Now substituting into equation (2):
R2=17.642+26.464=8.82+6.615=15.435N
Step 5: Calculate R1
Now, substitute R2 back into equation (1):
R1+15.435=41.895
R1=41.895−15.435=26.46N
Final Answer
The readings on the scales are:
- Scale 1 (left scale): R1≈26.46N
- Scale 2 (right scale): R2≈15.435N
- The beam has a mass m=1.8kg, so its weight Wb=mg=1.8×9.8N.
- The block has a mass M=2.7kg, so its weight Wb=Mg=2.7×9.8N.
- The beam is supported by two scales, which we will denote as R1 (left scale) and R2 (right scale).
- The total weight acting downwards on the system is:
Wtotal=Wb+WM=(1.8×9.8)+(2.7×9.8)=15.435N+26.46N=41.895N
Since the beam is at rest, we can apply the conditions for static equilibrium:
1. The sum of vertical forces must equal zero:
R1+R2=Wtotal
R1+R2=41.895N(1)
- The torque due to R2 (acting at distance L) is counterclockwise and is given by R2⋅L.
- The torque due to the weight of the beam (acting at L/2) is clockwise and is given by Wb⋅(L/2).
- The torque due to the weight of the block (acting at L/4) is also clockwise and is given by WM⋅(L/4).
R2⋅L−Wb⋅L2−WM⋅L4=0
Dividing through by L (since L≠0):
R2−Wb2−WM4=0
Substituting the weights:
R2=(1.8×9.8)2+(2.7×9.8)4(2)
Calculating the individual components:
- Wb=1.8×9.8=17.64N
- WM=2.7×9.8=26.46N
R2=17.642+26.464=8.82+6.615=15.435N
Now, substitute R2 back into equation (1):
R1+15.435=41.895
R1=41.895−15.435=26.46N
The readings on the scales are:
- Scale 1 (left scale): R1≈26.46N
- Scale 2 (right scale): R2≈15.435N
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - RIGID BODY DYNAMICS-IIEXERCISE - EXAMPLE1videos
RIGID BODY DYNAMICS-II
- BOOK - RESNICK AND HALLIDAYCHAPTER - RIGID BODY DYNAMICS-IIEXERCISE - CHECKPOINT4videos
RIGID BODY DYNAMICS-II
- BOOK - RESNICK AND HALLIDAYCHAPTER - RIGID BODY DYNAMICS - IEXERCISE - PRACTICE QUESTIONS (INTEGER TYPE))3videos
RIGID BODY DYNAMICS - I
- BOOK - RESNICK AND HALLIDAYCHAPTER - TEMPERATURE, ZEROTH LAW OF THERMODYNAMICS AND THERMAL EXPANSION EXERCISE - PRACTICE QUETIONS (Integer Type)4videos
TEMPERATURE, ZEROTH LAW OF THERMODYNAMICS AND THERMAL EXPANSION
Similar Questions
The radius of gyration of a uniform rod of mass m and length l about an axis perpendicular to its length and at distance l4 from its one end will be
View SolutionOne end of a thin uniform rod of length L and mass M1 is rivetted to the center of a uniform circular disc of radius r and mass M2 so that both are coplanar. The center of mass of the combination from the center of the disc is (Assume that the point of attachment is at the origin)
1) L(M1+M2)/2M1
2) LM1/2(M1+M2)
3) 2(M1+M2)/LM1
4) 2LM1/(M1+M2)View Solution
Knowledge Check
- Question 1 - Select One
A man of mass m stands at the left end of a uniform plank of length L and mass M, which lies on a frictionaless surface of ice. If the man walks to the other end of the plank, then by what distance does the plank slide on the ice?
ALBMLm+MCmLMDmLm+M - Question 2 - Select One
An elevator accelrates upward at a constant rate. A uniform string of length L and mass m supports a small block of mass M that hangs from the celling of the elevator. The tension at distance l from the celling is T. the accelration of the elegvator is
ATM+m−ml/L−gBT2M+m−ml/L+gCTM+ml/L−gDT2M−m+ml/L−g - Question 3 - Select One
A uniform rope of length 20m and mass 5kg is hanging vertically from a rigid support. A block of mass 4kg is attached to the free end. The wave length of the transverse wave pulse at the lower end of the rope is 0.04 m. The wavelength of the same pulse as it reaches the top is
A0.06mB0.12mC1.5mD2.2m
Two uniform solid of masses m1 and m2 and radii r1 and r2 respectively, are connected at the ends of a uniform rod of length l and mass m. Find the moment of inertia of the system about an axis perpendicular to the rod and passing through a point at a distance of a from the centre of mass of the rod as shown in figure.
 View Solution
View SolutionA thin uniform bar of length L and mass 8m lies on a smooth horizontal table .Two point masses m and 2 m are moving in the same horizontal plane from opposite sides of the bar with speeds 2v and v respectively .The masses stick to the bar after collision at a distances L3andL6 respectively from the center of the bar if the bar starts rotating about its center of mass as result of collision the angular speed of the bar will be
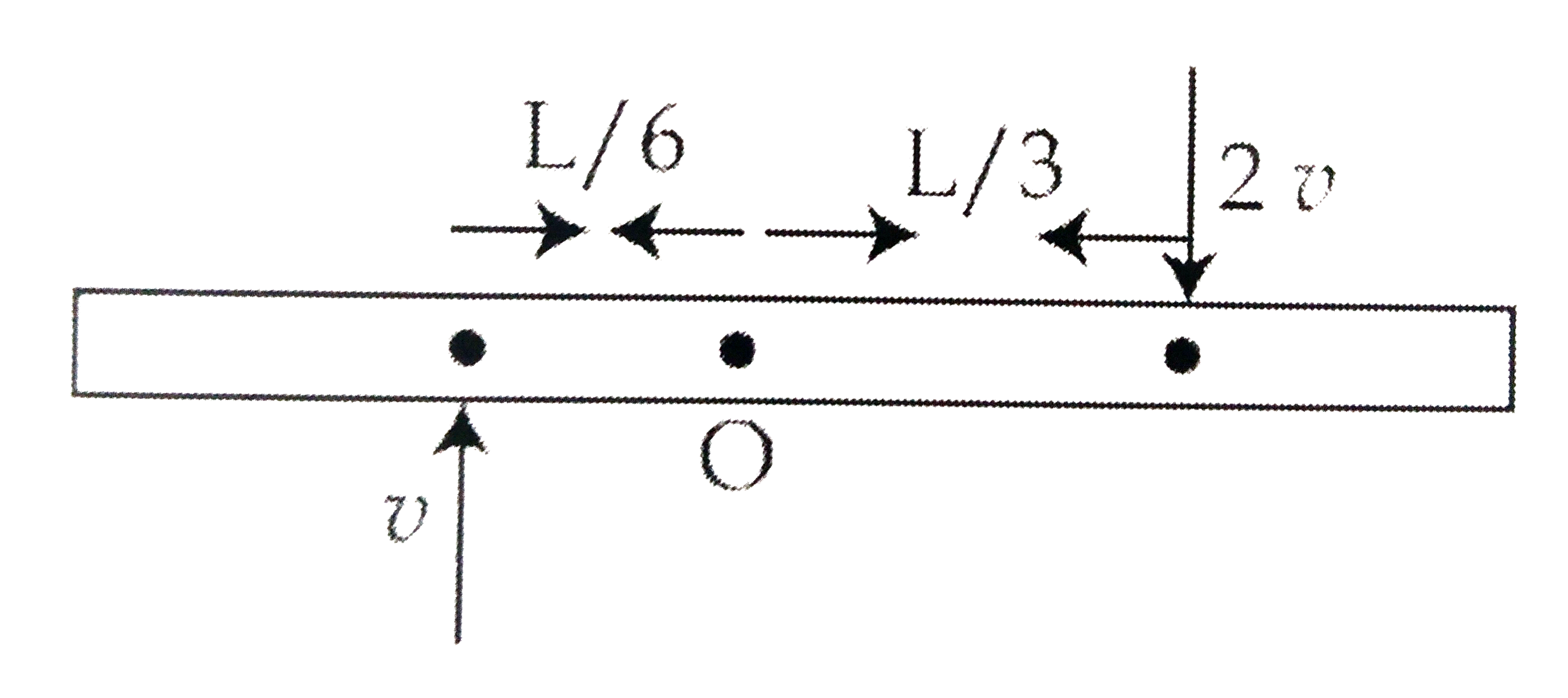 View Solution
View SolutionA uniform board of mass M and length L rests on two spring scales. A person of mass m stands at a distance l from one end as shown in Fig. (a) The board and person are at rest. What is the reading of the two scales?
View SolutionA uniform rod of mass M and length L rests on two supports. A ball of mass m=M/3 is placed at distance L/8 from the center as shown. Find reactions at supports.
 View Solution
View SolutionA uniform rod of length l and mass 2m rests on a smooth horizontal table. A point of mass m moving horizontally at right angle to the rod with velocity v collides with one end of the rod and sticks to it, then:
View Solution

