A uniform cube of mass M and side length a is placed at rest at the edge of a table. With half of the cube overhanging from the table, the cube begins to roll off the edge. There is sufficient friction at the edge so that the cube does not slip at the edge of the table. Find -
(a) the angle $θ_{0}$ through which the cube rotates before it leaves contact with the table.
(b) the speed of the centre of the cube at the instant it breaks off the table.
(c) the rotational kinetic energy of the cube at the instant its face AB becomes horizontal.

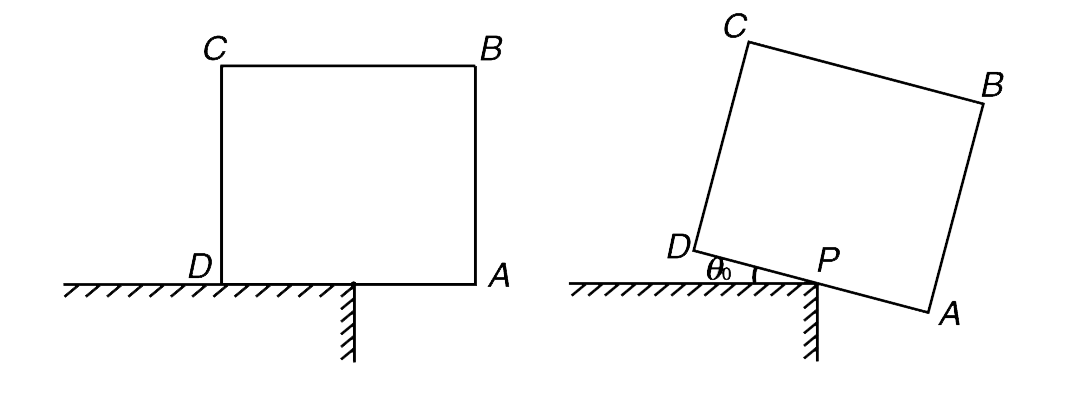
Text Solution

Verified by Experts

The correct Answer is:(a) ${cos}^{- 1} (\frac{6}{11})$
(b) $\sqrt{\frac{3 g a}{11}}$
(c) $\frac{m g a}{11}$

Updated on:21/07/2023

If the edge of a cube be 7 cm, then the volume of the cube is:
AA)321 ${cm}^{3}$
BB)343 ${cm}^{3}$
CC)433 ${cm}^{3}$
DD)243 ${cm}^{3}$
If the edge of a cube is doubled,then the volume of the cube is ___ that of the initial cube.
A4 times
B8 times
C12 times
D6 times
The edge of a cube is doubled then the percentage increase in the volume of cube is
A $100 %$
B $500 %$
C $300 %$
D $700 %$