A disc of mass m and radius R is moving on a smooth horizontal surface with the flat circular face on the surface. It is spinning about its centre with angular peed ω and has a velocity V (see figure). It just manages to hit a stick AB at its end A. The stick was lying free on the surface and stick to the disc. [The combined object becomes like a badminton racket]. Mass and length of the stick are m and 2R respectively.
(a) Calculate the angular speed of the combined object assuming V=Rω
(b) Calculate loss in kinetic energy. Why is energy lost ?
(c) If V=η(Rω), loss in kinetic energy is minimum. Find η. (Assume ω is given]
![A disc of mass m and radius R is moving on a smooth horizontal surface with the flat circular face on the surface. It is spinning about its centre with angular peed omega and has a velocity V (see figure). It just manages to hit a stick AB at its end A. The stick was lying free on the surface and stick to the disc. [The combined object becomes like a badminton racket]. Mass and length of the stick are m and 2R respectively. (a) Calculate the angular speed of the combined object assuming V = R omega (b) Calculate loss in kinetic energy. Why is energy lost ? (c) If V = eta (R omega), loss in kinetic energy is minimum. Find eta. (Assume omega is given]](https://d10lpgp6xz60nq.cloudfront.net/physics_images/IJA_PHY_V01_C06_E01_137_Q01.png)
The correct Answer is:(a) ω0=917ω
(b) ΔE=568mV2+734mR2ω2−317mVRω
(c) η=65
Topper's Solved these Questions
Similar Questions
A disc of mass m and radius R is kept on a smooth horizontal surface with its plane parallel to the surface. A particle of same mass m travelling with speed v0 collides with the stationery disc and gets embedded into it as shown in the figure. Then
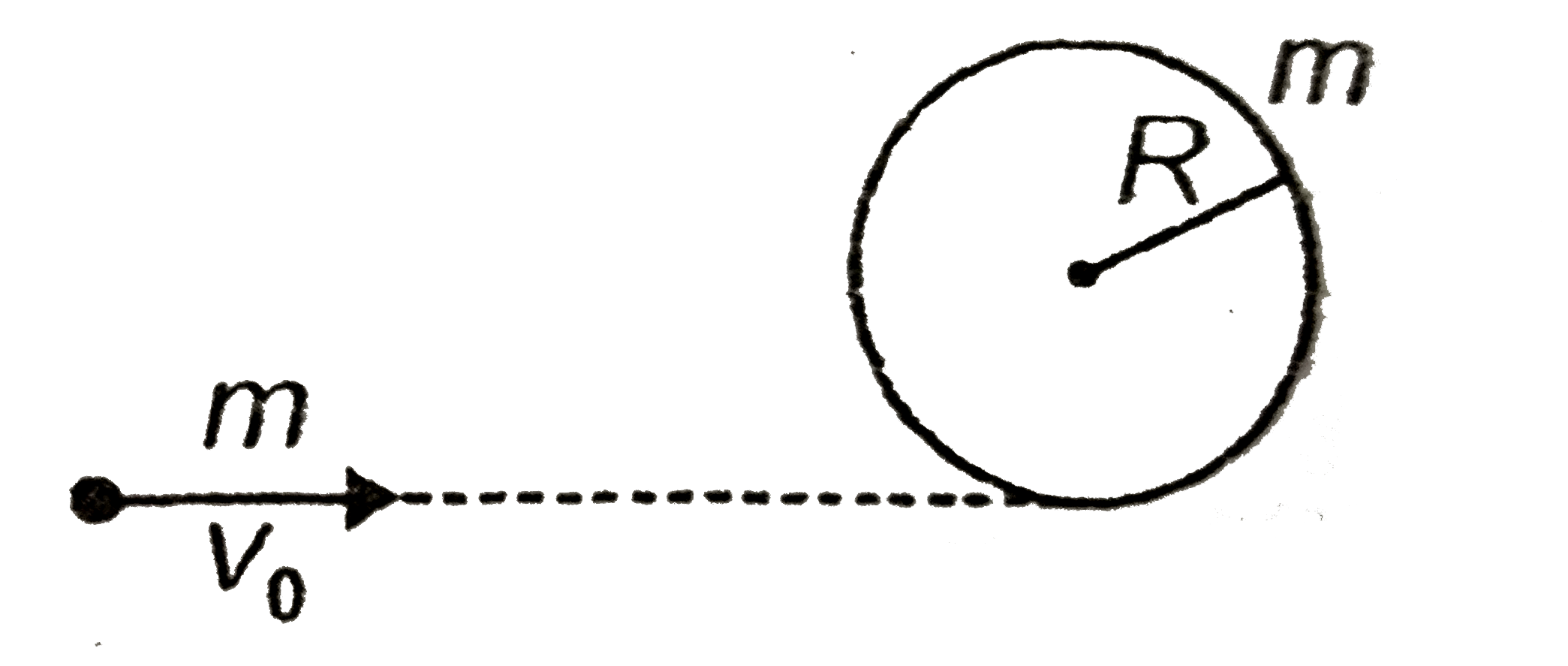
View SolutionA disc of mass m and radius R is kept on a smooth horizontal surface with its plane parallel to the surface. A particle of same mass m travelling with speed v0 collides with the stationery disc and gets embedded into it as shown in the figure. Then

View Solution
Knowledge Check
A disc of mass m and radius r placed on a routh horizontal surface. A cue of mass m hits the disc at a height h from the axis passing through centre and parallel to the surface. The disc will start pure rolling for.
Ah<r3Bh=r2Ch>r2Dh≥r2A uniform disc of mass m and radius R rotates about a fixed vertical axis passing through its centre with angular velocity ω. A particle of same angular velocity mass m and moving horizontally with velocity 2ωR towards centre of the disc collides and sticks to its rim. The impulse on the particle due to the disc is
A√173mωRB√353mωRC√373mωRD√293mωRA point mass m collides with a disc of mass m and radius R resting on a rough horizontal surface as shown . Its collision is perfectly elastic. Find angular velocity of the disc after pure rolling starts
 A(2u3R)B(3u3R)C(5u3R)D(2u5R)
A(2u3R)B(3u3R)C(5u3R)D(2u5R)
A disc of radius R is moving on a rough horizontal surface with velocity v and angular speed ω at an instant as shown in figure. Choose the correct statement.

View SolutionA disc of radius R is moving on a rough horizontal surface with velocity v and angular speed ω at an instant as shown in figure. Choose the correct statement.

View SolutionA uniform circular disc of radius R is rolling on a horizontal surface. Determine the tangential velocity : the centre of mass
View SolutionA point mass m collides with a disc of mass m and radius R resting on a rough horizontal surface as shown . Its collision is perfectly elastic. Find angular velocity of the disc after pure rolling starts

View SolutionA plastic circular disc of radius R is placed on a thin oil film spread over a flat horizontal surface the torque required to spin the disc about its central vertical axis with a constant angular velocity is proportional to
View Solution
