A uniform disc of mass M and radius R is mounted on an axle supported in frictionless bearings. A light cord is wrapped around the rim of the disc and a steady downward pull T is exerted on the cord. The angular acceleration of the disc is
TMR
MRT
2TMR
MR2T
The correct Answer is:C
To find the angular acceleration of a uniform disc of mass M and radius R when a steady downward pull T is exerted on a cord wrapped around its rim, we can follow these steps:
Step 1: Identify the Forces Acting on the Disc
The forces acting on the disc are:
- The tension T acting downward at the rim of the disc.
- The weight of the disc Mg acting downward at its center.
Step 2: Determine the Torque Due to the Tension
The torque τ caused by the tension T about the axis of rotation (center of the disc) can be calculated using the formula:
τ=T⋅R
where R is the radius of the disc.
Step 3: Apply Newton's Second Law for Rotation
According to Newton's second law for rotation, the net torque is equal to the moment of inertia I times the angular acceleration α:
τ=I⋅α
Step 4: Calculate the Moment of Inertia of the Disc
The moment of inertia I of a uniform disc about its center is given by:
I=12MR2
Step 5: Set Up the Equation
Substituting the expressions for torque and moment of inertia into the equation from Step 3:
T⋅R=(12MR2)⋅α
Step 6: Solve for Angular Acceleration α
Rearranging the equation to solve for α:
α=2TMR
Final Result
Thus, the angular acceleration α of the disc is:
α=2TMR
---
The forces acting on the disc are:
- The tension T acting downward at the rim of the disc.
- The weight of the disc Mg acting downward at its center.
The torque τ caused by the tension T about the axis of rotation (center of the disc) can be calculated using the formula:
τ=T⋅R
where R is the radius of the disc.
According to Newton's second law for rotation, the net torque is equal to the moment of inertia I times the angular acceleration α:
τ=I⋅α
The moment of inertia I of a uniform disc about its center is given by:
I=12MR2
Substituting the expressions for torque and moment of inertia into the equation from Step 3:
T⋅R=(12MR2)⋅α
Rearranging the equation to solve for α:
α=2TMR
Thus, the angular acceleration α of the disc is:
α=2TMR
Topper's Solved these Questions
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Multiple Correct
9 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Linked Comprehension
28 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Subjective
13 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICSCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE
2 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Interger
2 Videos
Similar Questions
A uniform disc of radius R and mass M is mounted on an axis supported in fixed friction less bearing. A light cord is wrapped around the rim of the wheel and suppose we hang a body of mass m from the cord.
(a) Find the angular acceleration of the disc and tangential acceleration ofpoint on the rim.
(b) At a moment t= 0, the system is set in motion, find the time dependence of the angular velocity of the system and the kinetic energy ofthe whole system.
View SolutionFigure shows a uniform disk, with mass M = 2.5 kg and radius R = 20 cm, mounted on a fixed horizontal axle. A block with mass m = 1.2 kg hangs from a massless cord that is wrapped around the rim of the disk. Find the acceleration of the falling block, the angular acceleration of the disk, and the tension in the cord. The cord does not slip, and there is no friction at the axle.
View Solution
Knowledge Check
A uniform disc of mass 2 kg and radius 1m is mounted on an axle supported on fixed frictionless bearings. A light chord is wrapped around the rim of the disc and mass of 1kg is tied to the free end. If it is released from rest-
Athe tension in the chord is 5NBin first four seconds the angular displacement of the disc is 40 radCthe work done by the torque on the disc in first four seconds is 200JDthe increase in the kinetic energy of the disc in the first four seconds in 200 JA uniform disc of mass M =2.50 kg and radius R=0.20 m is mounted on an axle supported on fixed frictionless bearings. A light cord wrapped around the rim is pulled with a force 5 N. On the same system of pulley and string, instead of pulling it down, a body of weight 5 N is suspended. If the first processis termed A and the second B, the tangential acceleration of point P will be:
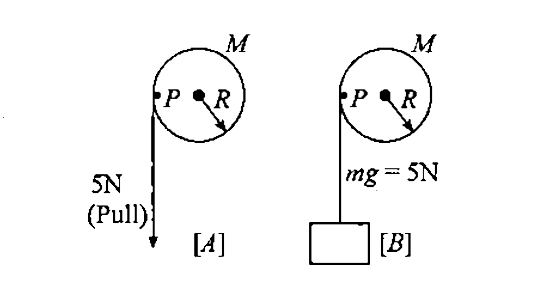 AEqual in the processes A and B.BGreaterin process A than in B.CGreater in process Bthan in 4.DIndependent of the two processes.
AEqual in the processes A and B.BGreaterin process A than in B.CGreater in process Bthan in 4.DIndependent of the two processes.A uniform disc of mass M and radius R is mounted on a fixed horizontal axis. A block of mass m hangs from a massless string that is wrapped around the rim of the disc. The magnitude of the acceleration of the falling block (m) is
A2MM+2mgB2mM+2mgCM+2m2MgD2M+m2Mg
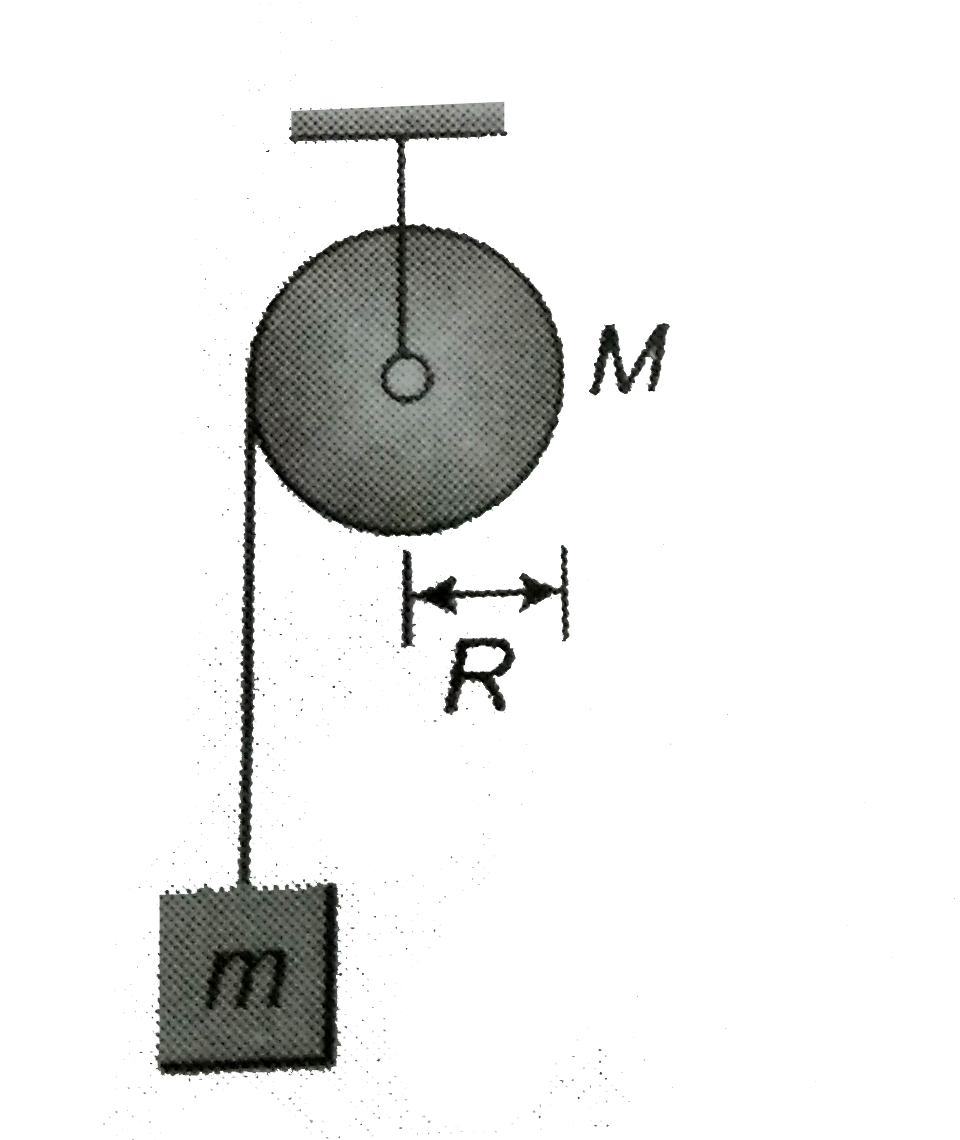
A frictionless pulley has the shape of a uniform solid disc of mass M and radius R. A stone of mass m is attached to a light wire that is wrapped around the rim of the pulley and a system is released from rest. If a stone falls by a distance h, what is the angular speed of pulley at that moment?
View SolutionA uniform disc of mass M and radius R is hinged at its centre C . A force F is applied on the disc as shown . At this instant , angular acceleration of the disc is

View Solution
A uniform disc of mass M and radius R is pivoted about the horizontal axis through its centre C A point mass m is glued to the disc at its rim, as shown in figure. If the system is released from rest, find the angular velocity of the disc when m reaches the bottom point B.
View SolutionIn Q.137, if we hang a body of mass m from the cord, the tangential acceleration of the disc is :
View SolutionA uniform disc of mass M and radius R is hinged at its centre C. A force F is applied on the disc as shown. At this instant, the angular acceleration of the disc is

View Solution
