A uniform rod of mas m and length l is kept vertical with the lower end clamed. It is slightly pushed to let it fall down under gravity. Find its angular speed when the rod is passing through its lowest positon. Neglect any friction at the clamp. What will be the linear speed of the free end at this instant?
To solve the problem of finding the angular speed of a uniform rod when it is passing through its lowest position and the linear speed of the free end at that instant, we can follow these steps:
Step 1: Understand the System
A uniform rod of mass m and length l is clamped at one end and allowed to fall under the influence of gravity. When it falls, it rotates about the clamp at the bottom.
Step 2: Apply the Work-Energy Theorem
According to the work-energy theorem, the work done by gravity on the rod will equal the change in kinetic energy of the rod. The work done by gravity can be calculated as:
W=mgh
where h is the vertical distance the center of mass of the rod falls. For a uniform rod of length l, the center of mass is at a distance l2 from the clamp. Thus, the height h is:
h=l2
So, the work done by gravity becomes:
W=mg(l2)=mgl2
Step 3: Calculate the Moment of Inertia
The moment of inertia I of a uniform rod about one end is given by:
I=13ml2
Step 4: Set Up the Energy Equation
The change in kinetic energy of the rod when it reaches the lowest position can be expressed as:
ΔKE=12Iω2−0
where ω is the angular speed at the lowest position. Therefore, we have:
mgl2=12(13ml2)ω2
Step 5: Simplify the Equation
We can cancel m from both sides (assuming m≠0):
gl2=16l2ω2
Multiplying both sides by 6:
3gl=l2ω2
Now, divide both sides by l2:
ω2=3gl
Step 6: Solve for Angular Speed
Taking the square root of both sides gives:
ω=√3gl
Step 7: Calculate the Linear Speed of the Free End
The linear speed v of the free end of the rod (which is at a distance l from the pivot) can be calculated using the relation:
v=ωl
Substituting the expression for ω:
v=√3gl⋅l=l√3gl=√3gl
Final Answers
1. The angular speed ω of the rod when it is passing through its lowest position is:
ω=√3gl
2. The linear speed v of the free end at this instant is:
v=√3gl
A uniform rod of mass m and length l is clamped at one end and allowed to fall under the influence of gravity. When it falls, it rotates about the clamp at the bottom.
According to the work-energy theorem, the work done by gravity on the rod will equal the change in kinetic energy of the rod. The work done by gravity can be calculated as:
W=mgh
where h is the vertical distance the center of mass of the rod falls. For a uniform rod of length l, the center of mass is at a distance l2 from the clamp. Thus, the height h is:
h=l2
So, the work done by gravity becomes:
W=mg(l2)=mgl2
The moment of inertia I of a uniform rod about one end is given by:
I=13ml2
The change in kinetic energy of the rod when it reaches the lowest position can be expressed as:
ΔKE=12Iω2−0
where ω is the angular speed at the lowest position. Therefore, we have:
mgl2=12(13ml2)ω2
We can cancel m from both sides (assuming m≠0):
gl2=16l2ω2
Multiplying both sides by 6:
3gl=l2ω2
Now, divide both sides by l2:
ω2=3gl
Taking the square root of both sides gives:
ω=√3gl
The linear speed v of the free end of the rod (which is at a distance l from the pivot) can be calculated using the relation:
v=ωl
Substituting the expression for ω:
v=√3gl⋅l=l√3gl=√3gl
1. The angular speed ω of the rod when it is passing through its lowest position is:
ω=√3gl
2. The linear speed v of the free end at this instant is:
v=√3gl
Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - HC VERMA ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Objective -1
26 Videos
- ROTATIONAL MECHANICSBOOK - HC VERMA ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Objective -2
14 Videos
- ROTATIONAL MECHANICSBOOK - HC VERMA ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Questions for short Answer
21 Videos
- REST AND MOTION : KINEMATICSBOOK - HC VERMA ENGLISHCHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Question for short Answer
13 Videos
- SIMPLE HARMONIC MOTIONBOOK - HC VERMA ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Question for short Answer
15 Videos
Similar Questions
A uniform rod of mass m and length l is suspended about its end. Time period of small angular oscillations is

View SolutionA rod of mass M and length L is falling vertically with speed v. Suddenly its one end gets stuck in a friciitonless hook. Find the angular velocity of the rod just after its end gets struck.

View SolutionA thin rod of mass m and length l is suspended from one of its ends. It is set into oscillation about a horizontal axis. Its angular speed is ω while passing through its mean position. How high will its centre of mass rise from its lowest position ?
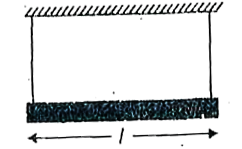
View SolutionA uniform rod of mass and length l is suspended by two strings at its ends shown. When one of the strings is cut, the rod starts falling with an initial angular acceleration.
View SolutionA uniform rod of length 1.00 m is suspended through an end and is set into oscillation with small amplitude under gravity. Find the time period of oscillation.
View SolutionA thin rod of mass m and length l is hinged at the lower end to a level floor and stands vertically. Then its upper end will strike the floor with a velocity given by:
View SolutionA uniform rod of mass 20 Kg and length 1.6 m is piovted at its end and swings freely in the vertical plane. Angular acceleration of the rod just after the rod is relased from rest in the horizontal position is

View SolutionA thin uniform rod AB of mass m and length l is hinged at one end to the level floor and stands vertically. If it s allowed to fall, with what angular velocity will it strike the floor?
View SolutionA uniform rod AB of mass m and length 2a is falling freely without rotation under gravity with AB horizontal. Suddenly the end A is fixed when the speed of the rod is v. The angular speed which the rod begains to rotate is
View SolutionA uniform rod of length l is kept vertically on a rough horizontal surface at x = 0 . It is rotated slightly and released . When the rod finally falls on the horizontal surface , the lower end will remain at
View Solution
