A uniform sphere of mass m and radius r rolls without sliding over a horizontal plane, rotating ahout a horizontal axle OA. In the process, the centre of the sphere moves with a veocity v along a circle of radius R. Find the kinetic energy of the sphere.
Here the motion of the body is oribital and spin combined. The sphere is spining about a horizontal axis and orbiting about a vertical axis.
We write total kinetic energy of the sphere i.e.,
KE=Korbital+Kspin
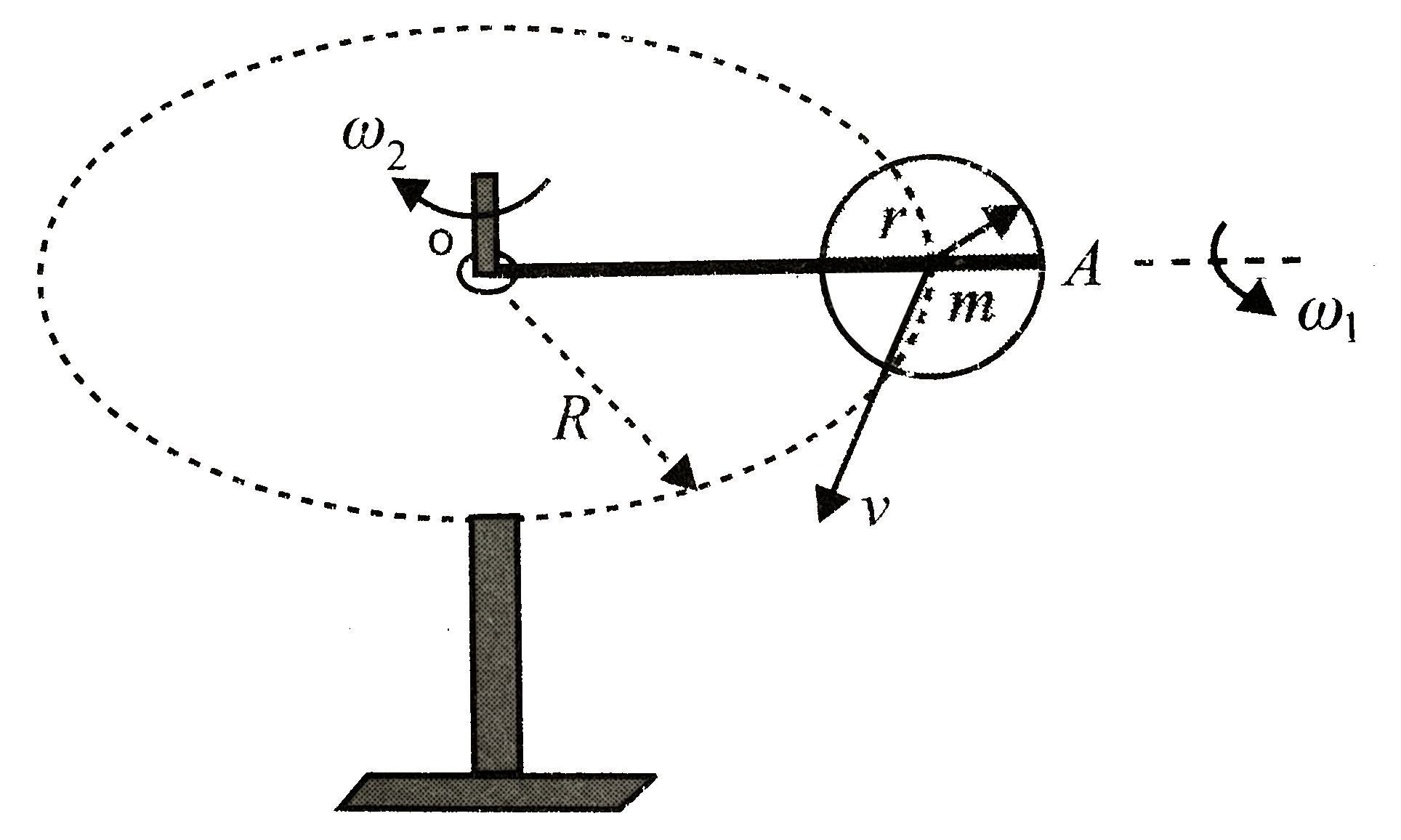
The kinetic energy of the sphere due to its rotation about its own axis nad its motion along circular path with velocity v.
KE=12I1ω21+12I2ω22
where I1=25mr2 and I2=(25mr2+mR2)
ω1=vr and ω2=vR
Substituting these values in the above equation, we get
KE=7m10v2[1+2r27R2]
Topper's Solved these Questions
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Solved Examples
12 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Exercise 3.1
11 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer
11 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICSCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer
16 Videos
Similar Questions
A uniform sphere of mass m and radius R starts rolling without slipping down an inclined plane at an angle α to the horizontal. Find the time dependence of the angular momentum of the sphere relative to the point of contact at the initial moment. How will the obtained result change in the case of a perfectly smooth inclined plane?
View SolutionA solid sphere of mass m rolls down an inclined plane a height h. Find rotational kinetic energy of the sphere.
View Solution
Knowledge Check
A solid sphere of mass m and radius R rolls without slipping on a horizontal surface such that vc.m.=v0.
AThe kinetic energy of rotation is 15mv20.BThe total kinetic energy is 710mv20.CThe mechanical energy (assume the ground as reference) is mgR+710mv20.DAll options are correct.A solid sphere of mass 1 kg and radius 10 cm rolls without slipping on a horizontal surface, with a velocity of 20 cm/s. The total kinetic energy of the sphere is
A0.014 JB0.028 JC14 JD28 JA solid sphere of mass 1 kg and radius 10 cm rolls without slipping on a horizontal surface, with a velocity of 20 cm/s. The total kinetic energy of the sphere is
A0.014 JB0.028 JC14 JD28 J
A uniform sphere of mass m and radius R rolls without slipping down an inclined plane set at an angle θ to the horizontal. Find
(a) the friction coefficient at which slipping is absent,
(b) the kinetic energy of the sphere t seconds after the beginning of motion.
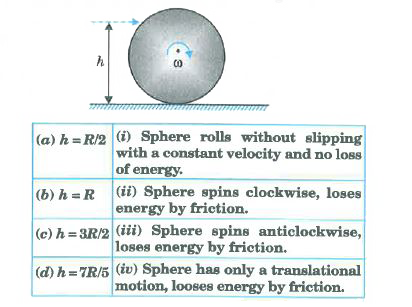
View SolutionA uniform sphere of mass m and radius R is placed on a rough horizontal surface. The sphere is struck horizontally at a heighth from the floor. Match the following:
View SolutionA uniform sphere of mass m and radius r rolls without slipping down a inclined plane, inclined at an angle 45∘ to the horizontal . Find the magnitude of frictional coefficient at which slipping is absent :
View SolutionIf a solid sphere of mass 1kg and radius 0.1m rolls without slipping at a unifrom velocity of 1ms−1 along a stright line on a horizontal floor, the kinetic energy of the sphere is
View SolutionIf a solid sphere of mass 1 kg and radius 0.1 m rolls without slipping at a uniform velocity of 1 m/s along a straight line on a horizontal floor, the kinetic energy is
View Solution
