A weight of 3 kg is placed on the pan of mass 1kg of a spring balance. IF the weight stretches the spring by 12 cm, find the frequency with which the empty pan oscillates.
To solve the problem step by step, we will follow these steps:
Step 1: Understand the System
We have a spring balance with a pan of mass mp=1kg and a weight of mw=3kg placed on it. The total weight acting on the spring is mw+mp=3kg+1kg=4kg.
Step 2: Calculate the Force Acting on the Spring
The total force due to the weight acting on the spring can be calculated using the formula:
F=m⋅g
where g is the acceleration due to gravity (g≈9.8m/s2).
F=4kg⋅9.8m/s2=39.2N
Step 3: Relate Force to Spring Constant
The force exerted by the spring when it is stretched is given by Hooke's Law:
F=k⋅x
where k is the spring constant and x is the extension of the spring. Given that the spring stretches by x=12cm=0.12m, we can set up the equation:
k⋅0.12=39.2
From this, we can solve for k:
k=39.20.12=326.67N/m
Step 4: Calculate the Time Period of Oscillation
The time period T of a mass-spring system is given by the formula:
T=2π√mk
In this case, the mass m that will oscillate is just the mass of the pan, mp=1kg:
T=2π√1326.67
Step 5: Calculate the Frequency of Oscillation
The frequency f is the reciprocal of the time period:
f=1T=12π√km
Substituting the values we have:
f=12π√326.671=12π⋅18.1≈2.878Hz
Final Answer
The frequency with which the empty pan oscillates is approximately 2.878Hz.
---
We have a spring balance with a pan of mass mp=1kg and a weight of mw=3kg placed on it. The total weight acting on the spring is mw+mp=3kg+1kg=4kg.
The total force due to the weight acting on the spring can be calculated using the formula:
F=m⋅g
where g is the acceleration due to gravity (g≈9.8m/s2).
F=4kg⋅9.8m/s2=39.2N
The force exerted by the spring when it is stretched is given by Hooke's Law:
F=k⋅x
where k is the spring constant and x is the extension of the spring. Given that the spring stretches by x=12cm=0.12m, we can set up the equation:
k⋅0.12=39.2
From this, we can solve for k:
k=39.20.12=326.67N/m
The time period T of a mass-spring system is given by the formula:
T=2π√mk
In this case, the mass m that will oscillate is just the mass of the pan, mp=1kg:
T=2π√1326.67
The frequency f is the reciprocal of the time period:
f=1T=12π√km
Substituting the values we have:
f=12π√326.671=12π⋅18.1≈2.878Hz
The frequency with which the empty pan oscillates is approximately 2.878Hz.
Topper's Solved these Questions
- OSCILLATIONS BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - PRACTICE PROBLEMS
15 Videos
- OSCILLATIONS BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - PRACTICE PROBLEMS 2
20 Videos
- MOTION IN A STRAIGHT LINE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - CHAPTER PRACTICE TEST
16 Videos
- PHYSICAL WORLD BOOK - MODERN PUBLICATIONCHAPTER - PHYSICAL WORLD EXERCISE - Revision exercises (Long answer questions)
6 Videos
Similar Questions
The pan attached to a spring balance has a mass of 1 kg. A weight of kg when placed on the pan stretches the spring by 10 cm. What is the frequency with which the empty pan will oscillate ?
View SolutionA pan of 2 kg is attached to a spring balance. When a weight of 3 kg is placed on the pan. The spring is stretched by 12 cm. Calculate the frequency with which the empty pan will oscillate.
View Solution
Knowledge Check
A body of mass m falls from a height h onto the pan of a spring balance. The masses of the pan and spring are negligible. The force constant of the spring is k. The body sticks to the pan and oscillates simple harmonically. The amplitude of oscillation is
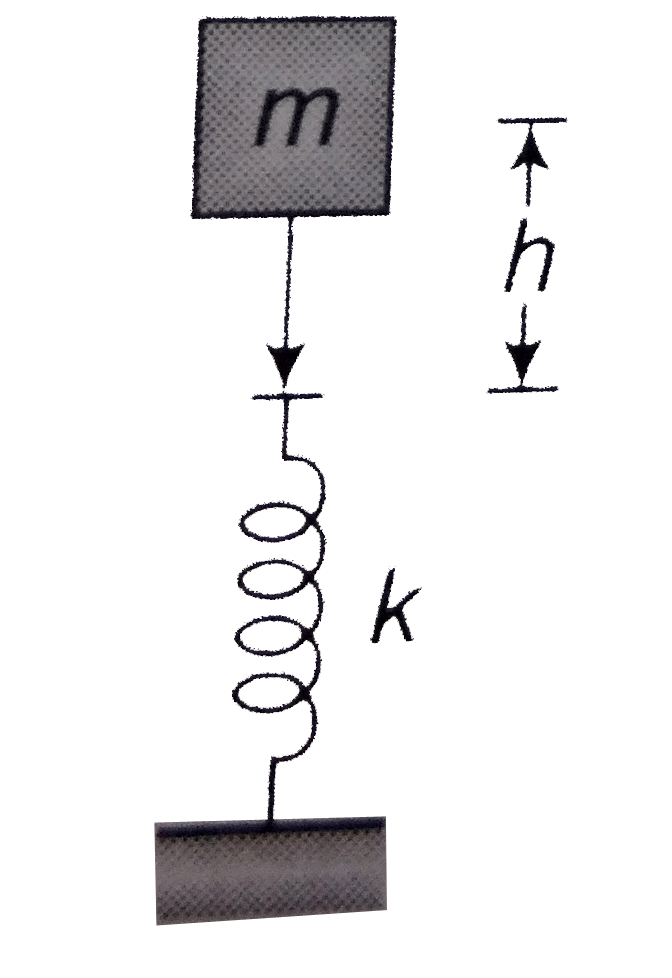 AmgkBmgk√1+2hkmgCmgk(1+√1+2hkmg)Dmgk√1+(2hkmg)−1
AmgkBmgk√1+2hkmgCmgk(1+√1+2hkmg)Dmgk√1+(2hkmg)−1A body weighs 6gms when placed in one pan and 24gms when placed on the other pan of a false balance. If the beam is horizontal when both the pans are empty, the true weight of the body is :
A13 gmB12 gmC15.5 gmD15 gmA mass of 1kg is hanged through a spring. The spring is stretched through 1cm. What is the energy stared in spring?
A0.449JB0.049JC4.9JD49J
When a weight of 2kg is suspended from a spring, its length increases by 3 cm. If the weight is further pulled down by 12 cm and then released, it executes S.H.M. What will be the period of oscillation of the arrangement ? Also calculate the potential energy of the spring .
View SolutionA ball of mass m falls from a certain height on the pan of mass M(M>>m) of a spring balance. The rigidity of the s prin is k. Determine the displacement Δx of the point about which the pointer of the balance will oscillate, assuming that the collisions of the ball with the pan are erfectly elastic.
View SolutionOn compressing a spring by 0.5 m, a restoring force of 20 N is developed. When a body of mass 5 kg is placed on the spring, then calculate the (a) force constant of the spring.
(b) the distance through which the spring is depressed under the weight of the body.
(c ) the period of oscillation of the spring if the the body is pushed down and then released.
View SolutionA student took a spring balance calibrated in gf. He then suspended an object of mass 1 kg to the spring balance. Determine what would be the reading of the spring balance.
View SolutionA mass of 1 kg is hanged through a spring. The spring is stretched through 1cm. What is the energy stared in spring?
View Solution
