A weight w is suspended from the midpoint of a rope, whose ends are at the same level. In order to make the rope perfectly horizontal, the force applied to each of its ends must be
less than w
equal to w
equal to 2w
infinitely large
The correct Answer is:D
To solve the problem of determining the force applied to each end of a rope in order to make it perfectly horizontal when a weight w is suspended from its midpoint, we can follow these steps:
1. Understanding the Setup:
- We have a rope with a weight w hanging from its midpoint. The ends of the rope are at the same level.
- When the weight is suspended, the rope forms an angle θ with the horizontal at both ends.
2. Identifying Forces:
- The weight w acts downward at the midpoint of the rope.
- The tension T in the rope acts along the rope, directed away from the weight at both ends.
3. Components of Tension:
- The tension T can be resolved into two components:
- A vertical component: Tsin(θ) (acting upward)
- A horizontal component: Tcos(θ) (acting horizontally)
4. Equilibrium Condition:
- For the weight to be in equilibrium, the sum of the vertical components of the tension must equal the weight:
2Tsin(θ)=w
- Here, 2Tsin(θ) accounts for the upward force from both sides of the rope.
5. Solving for Tension:
- Rearranging the equation gives:
T=w2sin(θ)
6. Condition for Horizontal Rope:
- To make the rope perfectly horizontal, the angle θ must be 90∘.
- At θ=90∘, sin(90∘)=1.
7. Substituting the Angle:
- Substituting θ=90∘ into the tension equation:
T=w2⋅1=w2
8. Conclusion:
- The force applied to each end of the rope must be w2 to make the rope perfectly horizontal.
Final Answer:
The force applied to each end of the rope must be w2.
---
- We have a rope with a weight w hanging from its midpoint. The ends of the rope are at the same level.
- When the weight is suspended, the rope forms an angle θ with the horizontal at both ends.
- The weight w acts downward at the midpoint of the rope.
- The tension T in the rope acts along the rope, directed away from the weight at both ends.
- The tension T can be resolved into two components:
- A vertical component: Tsin(θ) (acting upward)
- A horizontal component: Tcos(θ) (acting horizontally)
- For the weight to be in equilibrium, the sum of the vertical components of the tension must equal the weight:
2Tsin(θ)=w
- Here, 2Tsin(θ) accounts for the upward force from both sides of the rope.
- Rearranging the equation gives:
T=w2sin(θ)
- To make the rope perfectly horizontal, the angle θ must be 90∘.
- At θ=90∘, sin(90∘)=1.
- Substituting θ=90∘ into the tension equation:
T=w2⋅1=w2
- The force applied to each end of the rope must be w2 to make the rope perfectly horizontal.
The force applied to each end of the rope must be w2.
Topper's Solved these Questions
- NEWTONS LAWS OF MOTION AND FRICTIONBOOK - BITSAT GUIDECHAPTER - NEWTONS LAWS OF MOTION AND FRICTIONEXERCISE - BITSAT Archives
23 Videos
- MOTION IN !,2,2 DIMENSIONS AND POJECTILE MOTIONBOOK - BITSAT GUIDECHAPTER - MOTION IN !,2,2 DIMENSIONS AND POJECTILE MOTIONEXERCISE - All Questions
86 Videos
- ROTATIONAL MOTIONBOOK - BITSAT GUIDECHAPTER - ROTATIONAL MOTIONEXERCISE - BITSAT Archives
9 Videos
Similar Questions
A weight mg is suspended from the middle of a rope whose ends are at the same level. The rope is no longer horizontal. Find the minimum tenstion required to completely straighten the rope.
View SolutionA 10kg body is suspended by a rope is pulled by means of a horizontal force to make 30∘ by rope to vertical.The horizontal force is
View Solution
Knowledge Check
A weight w is sasponded from the mid -point of a tope whose ends are at the same level In after in make the rope perfectly horizontalthe force applied to each of its ends must be
Aless then wBequal to wCequal to 2wDinfinitly largeA heavy rope is suspended from a rigid support. A wave pulse is set up at the lower end, then
Athe pulse will travel with uniform speedBthe pulse will travel with increasing speedCthe pulse will travel with decreasing speedDthe pulse cannot travel through the ropeA heavy rope is suspended from a rigid support A wave pulse is set up at the lower end, then
AThe pulse will travel with uniform speedBThe pulse will travel with increasing speedCThe pulse will travel with decreasing speedDThe pulse cannot travel through the rope
A 10kg body is suspended by a rope is pulled by means of a horizontal force to make 60∘ by rope to vertical.The horizontal forces is
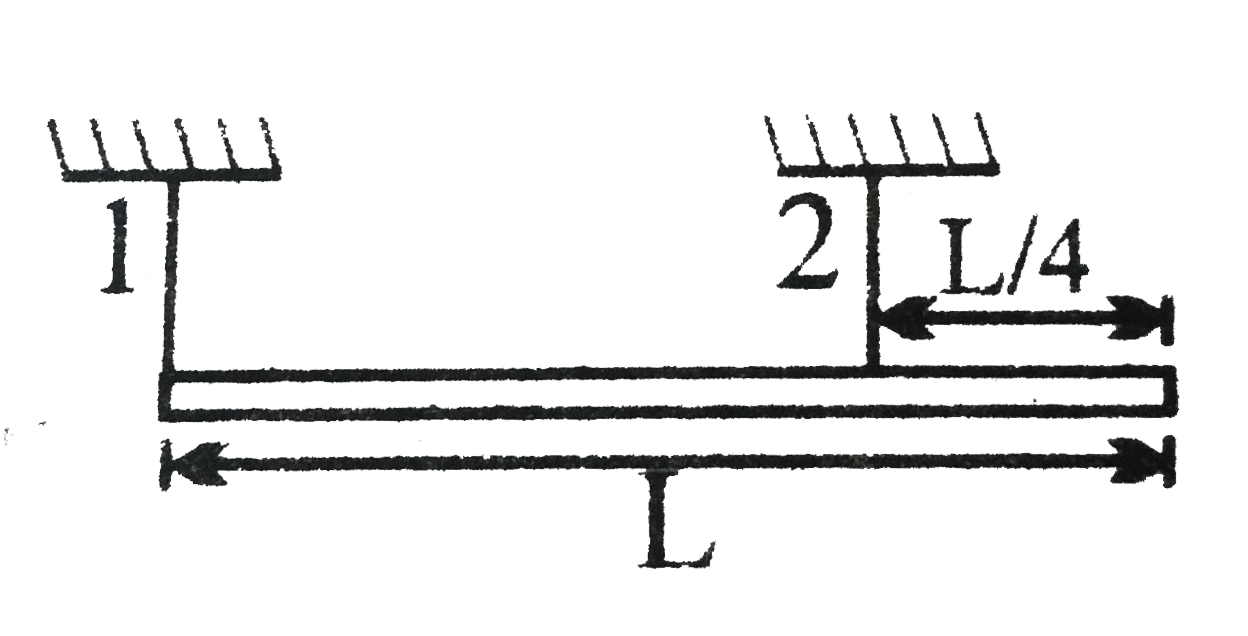
View SolutionA uniform rod of length L and weight W is suspended horizontally by two vertical ropes as shown. The first rope is attached to the left end of the rod while the second is attached to the left end of the rod while the second rope is attached a distance L/4 from the right end. The tension in the second rope is.
 .
.
View SolutionOne end of a uniform bar of weight w1 is suspended from the roof and a weight w2 is suspended from the other end, the area of cross-section is A. What is the stress at the midpoint of the rod ?
View SolutionOne end of a uniform bar of weight w1 is suspended from the roof and a weight w2 is suspended from the other end, the area of cross-section is A. What is the stress at the midpoint of the rod ?
View SolutionA string of length L and radius r elongated by x due to its own weight, when suspended from the ceiling from its one end. If another string of same material, same length and radius double the initial is suspended from its one end then elongation due to its own weight will be
View Solution
