A wheel starts rolling up a rough inclined plane and another identical wheel starts rolling up a smooth plane having same inclination with the horizontal. If initial velocity of both the wheels is same then:
stops ascending earlier than
kinetic energy of never becomes zero
maximum height ascended by is less than that, by
friction acting on remains constant during the round trip
The correct Answer is:B, D
To solve the problem, we will analyze the motion of both wheels A and B as they roll up their respective inclined planes.
Step 1: Understanding the System
We have two identical wheels:
- Wheel A is rolling up a rough inclined plane.
- Wheel B is rolling up a smooth inclined plane.
Both wheels start with the same initial linear velocity v0 and angular velocity ω0.
Step 2: Forces Acting on the Wheels
For Wheel A (on the rough plane):
- The forces acting on it include:
- Gravitational force component along the incline: mgsinθ
- Normal force: N=mgcosθ
- Frictional force: f (acting up the incline since it opposes the motion)
For Wheel B (on the smooth plane):
- The forces acting on it include:
- Gravitational force component along the incline: mgsinθ
- Normal force: N=mgcosθ
- There is no frictional force acting on Wheel B because the surface is smooth.
Step 3: Equations of Motion
For Wheel A, the net force acting on it as it rolls up the incline can be expressed as:
maA=−mgsinθ−f
Where aA is the linear acceleration of Wheel A.
For Wheel B, since there is no friction, the equation simplifies to:
maB=−mgsinθ
Where aB is the linear acceleration of Wheel B.
Step 4: Rolling Condition
For Wheel A, it will eventually achieve pure rolling motion, which means:
aA=αR
Where α is the angular acceleration and R is the radius of the wheel.
For Wheel B, since there is no friction, it will not experience any torque, and thus its angular velocity will remain constant while its linear velocity decreases.
Step 5: Energy Considerations
Using conservation of energy:
- For Wheel A:
12Iω2+12mv2=mghA
Where hA is the height reached by Wheel A.
- For Wheel B:
12mv2=mghB
Where hB is the height reached by Wheel B.
Step 6: Comparing Heights
Since Wheel A loses both translational and rotational kinetic energy while Wheel B only loses translational kinetic energy, we can conclude that:
hA>hB
This means Wheel A will ascend to a greater height than Wheel B.
Conclusion
- Wheel A will stop ascending later than Wheel B because it can convert both its translational and rotational kinetic energy into potential energy.
- The maximum height ascended by Wheel A is greater than that of Wheel B.
We have two identical wheels:
- Wheel A is rolling up a rough inclined plane.
- Wheel B is rolling up a smooth inclined plane.
For Wheel A (on the rough plane):
- The forces acting on it include:
- Gravitational force component along the incline: mgsinθ
- Normal force: N=mgcosθ
- Frictional force: f (acting up the incline since it opposes the motion)
- The forces acting on it include:
- Gravitational force component along the incline: mgsinθ
- Normal force: N=mgcosθ
- There is no frictional force acting on Wheel B because the surface is smooth.
For Wheel A, the net force acting on it as it rolls up the incline can be expressed as:
maA=−mgsinθ−f
Where aA is the linear acceleration of Wheel A.
maB=−mgsinθ
Where aB is the linear acceleration of Wheel B.
For Wheel A, it will eventually achieve pure rolling motion, which means:
aA=αR
Where α is the angular acceleration and R is the radius of the wheel.
Using conservation of energy:
- For Wheel A:
12Iω2+12mv2=mghA
Where hA is the height reached by Wheel A.
12mv2=mghB
Where hB is the height reached by Wheel B.
Since Wheel A loses both translational and rotational kinetic energy while Wheel B only loses translational kinetic energy, we can conclude that:
hA>hB
This means Wheel A will ascend to a greater height than Wheel B.
- Wheel A will stop ascending later than Wheel B because it can convert both its translational and rotational kinetic energy into potential energy.
- The maximum height ascended by Wheel A is greater than that of Wheel B.
Topper's Solved these Questions
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Linked Comprehension
71 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Integer
7 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Single Correct
142 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer
11 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer
16 Videos
Similar Questions
Knowledge Check
When a body starts to roll on an inclined plane, its potential energy is converted into
ATranslational kinetic energy onlyBTranslational and rotational kinetic energyCRotational energy onlyDNoneA solid cylinder rolls up an inclined plane of inclination θ with an initial velocity v. How far does the cylinder go up the plane ?
A3v22gsinθBv24gsinθC3v2gsinθD3v24gsinθ
The velocity of a sphere rolling down an inclined plane of height h at an inclination θ with the horizontal, will be :
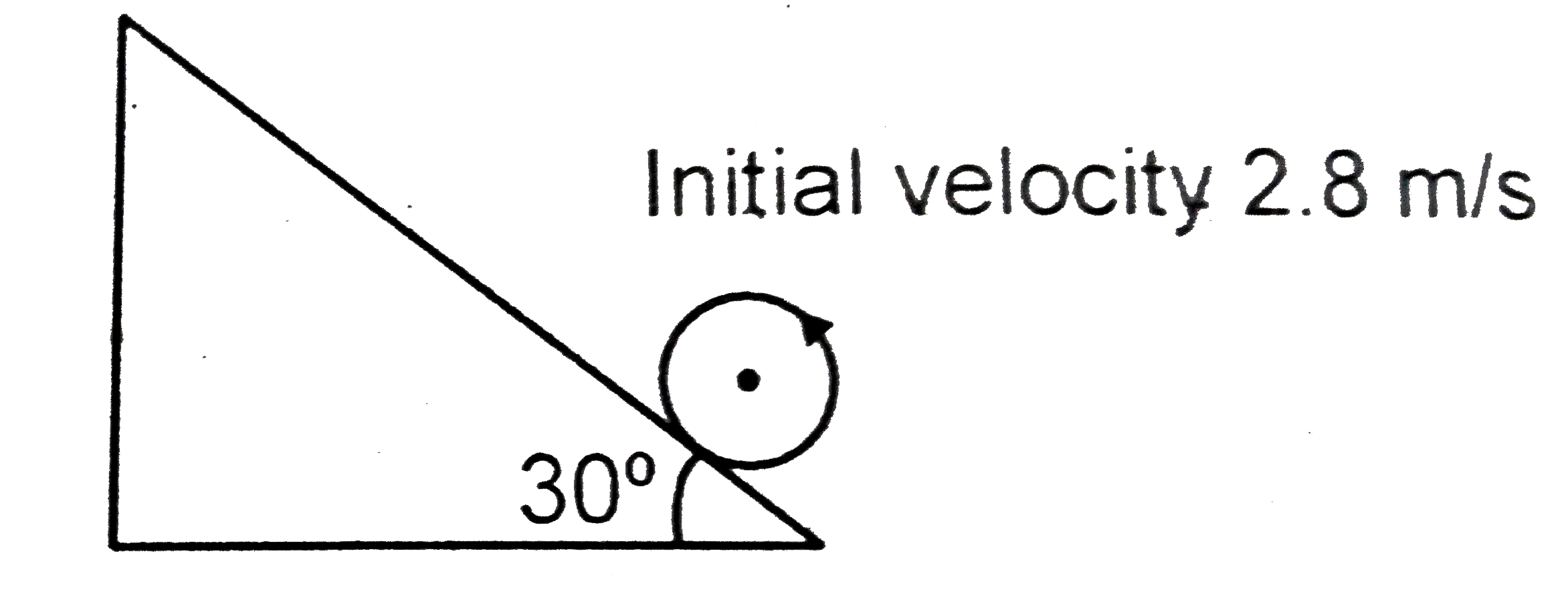
View SolutionA Sphere pure rolls on a rough inclined plane with initial velocity 2.8 m/s. Find the maximum distance on the inclined plane
View SolutionWhen a body rolls without sliding up an inclined plane the frictional force is :
View SolutionStarting from rest, the time taken by a body sliding down on a rough inclined plane at 45∘ with the horizontal is twice the time taken to travel on a smooth plane of same inclination and same distance. Then, the coefficient of kinetic friction is
View SolutionA block is pushed up a rough inclined plane of 45°. If the time of descent is twice the time of ascent, the coefficient of friction is
View SolutionA block slides down a rough inclined plane of slope angle θ with a constnat velocity. It is then projected up the same plane with an intial velocity v the distance travelled by the block up the plane coming to rest is .
View Solution
