A wire is suspended from the ceiling and stretched under the action of weight F suspended from its other end. The force exerted by the ceiling on it is equal and opposite to the weight.
Tensile stress at any cross section A of the wire is FA.
Tensile stress at any cross section is zero.
Tensile stress at any cross section A of the wire is 2FA.
Tension at any cross section A of the wire is 2F.
The correct Answer is:A
To solve the problem step by step, let's analyze the situation described:
Step 1: Understand the Setup
We have a wire suspended from the ceiling with a weight F hanging from its end. The wire experiences tension due to the weight, and the ceiling exerts an equal and opposite force on the wire.
Hint: Draw a diagram to visualize the wire, the weight, and the forces acting on the wire.
Step 2: Identify Forces Acting on the Wire
1. The weight F acts downward due to gravity.
2. The tension T in the wire acts upward.
3. According to Newton's third law, the force exerted by the ceiling on the wire is equal and opposite to the weight F.
Hint: Remember that in equilibrium, the sum of forces acting on the wire must equal zero.
Step 3: Establish the Relationship Between Forces
Since the wire is in equilibrium:
T=F
where T is the tension in the wire and F is the weight acting downward.
Hint: Use the equilibrium condition to relate the forces acting on the wire.
Step 4: Calculate the Tensile Stress
Tensile stress (σ) is defined as the force per unit area. The formula for tensile stress is:
σ=TA
Substituting T with F:
σ=FA
where A is the cross-sectional area of the wire.
Hint: Recall the definition of tensile stress and ensure you use the correct area for calculations.
Step 5: Analyze the Cross-Sectional Area
At any cross-section A of the wire, the tensile stress is uniform and is given by:
σ=FA
Hint: Make sure to consider that the area A is constant along the length of the wire if it is uniform.
Step 6: Conclusion
The tensile stress at any cross-section A of the wire is FA, and the tension in the wire is equal to the weight F.
Final Result:
- Tensile Stress at any cross-section A: FA
- Tension in the wire: F
We have a wire suspended from the ceiling with a weight F hanging from its end. The wire experiences tension due to the weight, and the ceiling exerts an equal and opposite force on the wire.
1. The weight F acts downward due to gravity.
2. The tension T in the wire acts upward.
3. According to Newton's third law, the force exerted by the ceiling on the wire is equal and opposite to the weight F.
Since the wire is in equilibrium:
T=F
where T is the tension in the wire and F is the weight acting downward.
Tensile stress (σ) is defined as the force per unit area. The formula for tensile stress is:
σ=TA
Substituting T with F:
σ=FA
where A is the cross-sectional area of the wire.
At any cross-section A of the wire, the tensile stress is uniform and is given by:
σ=FA
The tensile stress at any cross-section A of the wire is FA, and the tension in the wire is equal to the weight F.
- Tensile Stress at any cross-section A: FA
- Tension in the wire: F
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - HOOKE S LAW
3 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - STRESS-STRAIN CURVE
5 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - ELASTIC BEHAVIOR OF SOLIDS
2 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - NCERT Exemplar
5 Videos
- MOTION IN A PLANE BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOTION IN A PLANE EXERCISE - Assertion And Reason
15 Videos
Similar Questions
A body of weight w1 is suspended from the ceiling of a room through as chain of weight w2. The ceiling pulls the chain by a force
View Solution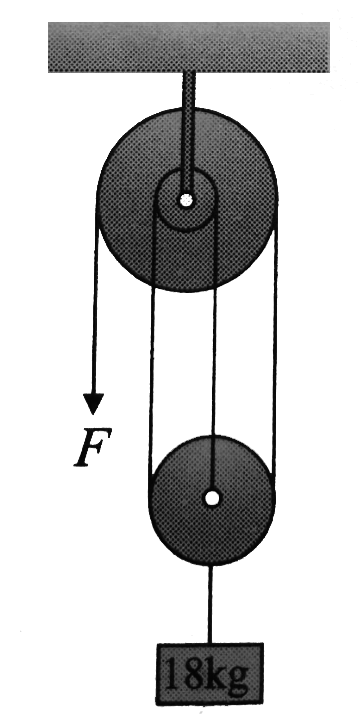
In the figure, at the free end of the light string, a force F is supplied to keep the suspended mass of 18 kg at rest. Then the force exerted by the ceiling on the system (assume that the string segments are vertical and the pulleys are light and smooth) is:(g=10ms2)
View SolutionA string is suspended from the ceiling. A wave train is produced at the bottom at regular interval. As the wave moves upwards

View Solution
A block of mass m is suspended from one end of a light spring as shown. The origin O is considered at distance equal to natural length of the spring from the ceiling and vertical downwards direction as positive y-axis. When the system is in equilibrium a bullet of mass m3 moving in vertical up wards direction with velocity v0 strikes the block and embeds into it. As a result, the block (with bullet embedded into it) moves up and start oscillating. Based on the given information, answer the following question:
Q. The time taken by the block bullet system to move from y=mgk (initial equilibrium position) to y=0 (natural length of spring) is (A represents the amplitude of motion)
View SolutionA block of mass 0.2 kg is suspended from the ceiling by a light string. A second block of mass 0.3 kg is suspended from the first block through another string. Find the tensions in the two strings. Take g=10ms2.
View SolutionA steel wire 2m long is suspended from the ceiling. When a mass is hung from its lower end, the increase in length recorded is 1cm. Determine the strain in the wire.
View SolutionOne end of a wire of length L and weight w is attached rigidly to a point in roof and a weight w1 is suspended from its lower end. If A is the area of cross-section of the wire then the stress in the wire at a height 3L4 from its lower end is
View SolutionA mass m is suspended from a wire . Change in length of the wire is Δl. Now the same wire is stretched to double its length and the same mass is suspended from the wire. The change in length in this case will become (it is suspended that elongation in the wire is within the proportional limit)
View SolutionA mass m is suspended from a wire . Change in length of the wire is Δl. Now the same wire is stretched to double its length and the same mass is suspended from the wire. The change in length in this case will become (it is suspended that elongation in the wire is within the proportional limit)
View SolutionSteel wire of length 'L' at 40∘C is suspended from the ceiling and then a mass 'm' is hung from its free end. The wire is cooled down from 40∘C→30∘C to regain its original length 'L'. The coefficient of linear thermal expansion of the steel is 10−5/∘C, Young's modulus of steel is 1011N/m2 and radius of the wire is 1mm. Assume that L>> diameter of the wire. Then the value of 'm' in kg is nearly
View Solution
