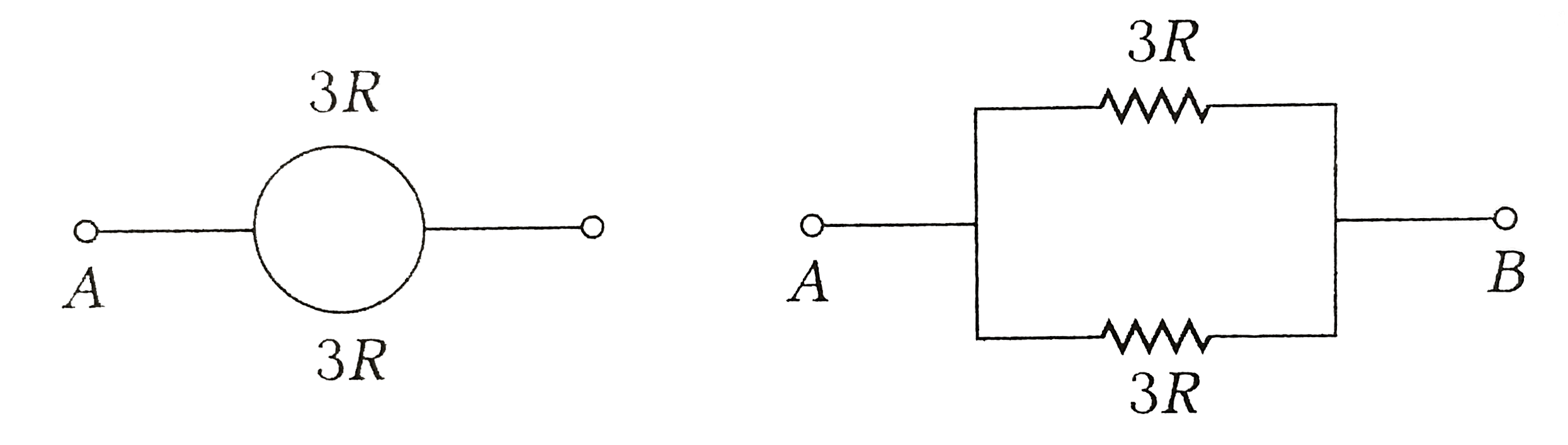
A wire of resistance 16Ω is bent in the form of a circle. What is the effective resistance between diametrically opposite points?
1Ω
2Ω
4Ω
8Ω
The correct Answer is:C
To find the effective resistance between diametrically opposite points on a circular wire of resistance 16 Ω, we can follow these steps:
1. Understand the Configuration:
- A wire of total resistance R=16Ω is bent into a circular shape.
- We need to find the effective resistance between two points that are diametrically opposite on the circle.
2. Divide the Wire:
- When the wire is bent into a circle, the total resistance is evenly distributed along the circumference.
- Since we are interested in the resistance between two diametrically opposite points, we can visualize the circle being divided into two equal halves.
3. Calculate Resistance of Each Half:
- The total resistance of the wire is 16Ω.
- Each half of the wire will have a resistance of:
Rhalf=R2=16Ω2=8Ω
4. Set Up the Parallel Resistance:
- The two halves of the wire (each with resistance 8Ω) are connected in parallel when considering the effective resistance between the two diametrically opposite points.
- Let R1=8Ω and R2=8Ω.
5. Use the Parallel Resistance Formula:
- The formula for two resistors in parallel is given by:
1Reffective=1R1+1R2
- Substituting the values:
1Reffective=18+18=28=14
6. Calculate the Effective Resistance:
- Taking the reciprocal gives us:
Reffective=4Ω
Final Answer:
The effective resistance between the diametrically opposite points is 4Ω.
- A wire of total resistance R=16Ω is bent into a circular shape.
- We need to find the effective resistance between two points that are diametrically opposite on the circle.
- When the wire is bent into a circle, the total resistance is evenly distributed along the circumference.
- Since we are interested in the resistance between two diametrically opposite points, we can visualize the circle being divided into two equal halves.
- The total resistance of the wire is 16Ω.
- Each half of the wire will have a resistance of:
Rhalf=R2=16Ω2=8Ω
- The two halves of the wire (each with resistance 8Ω) are connected in parallel when considering the effective resistance between the two diametrically opposite points.
- Let R1=8Ω and R2=8Ω.
- The formula for two resistors in parallel is given by:
1Reffective=1R1+1R2
- Substituting the values:
1Reffective=18+18=28=14
- Taking the reciprocal gives us:
Reffective=4Ω
The effective resistance between the diametrically opposite points is 4Ω.
Topper's Solved these Questions
Similar Questions
Knowledge Check
What is the effective resistance between points A and B?
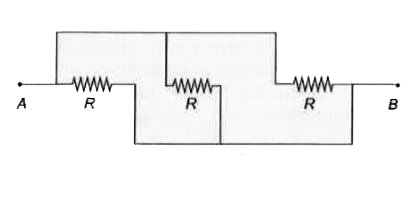 AR3B4R3C2R3DR2
AR3B4R3C2R3DR2What is the effective resistance between points P and Q?
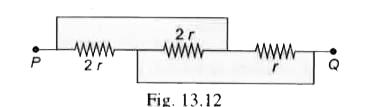 Ar2Br3C2R3D4r3
Ar2Br3C2R3D4r3What is the effective resistance between points P and Q?
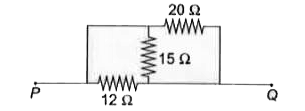 A5ΩB10ΩC15ΩD20Ω
A5ΩB10ΩC15ΩD20Ω
A wire of resistance 8R is bent in the form of a circle. What is the effective resistance between the ends of a diameter AB?
View SolutionA wire of resistance 8R is bent in the form of a circle. What is the effective resistance between the ends of a diameter AB ?
View Solution(a) Justify the following statements
(i) Tungsten is used exclusively for filament by electric lamps
(ii) We do not use series arrangement for domestic circuits.
(b) A wire of resistance 8Ω is bent in the form of a closed circle. What is the effective resistance between two points A and B at the ends of any diameter of the circle ?
View SolutionA wire of resistance 5 Ohms is bent in the form of a closed circle. What is the effective resistance between the two points at ends of any diameter of the circle?

View SolutionA wire of resistance 4 R is bent in the form of a circle [Fig 4.4]. What is the effective resistance between the ends of the diameter ?

View Solution