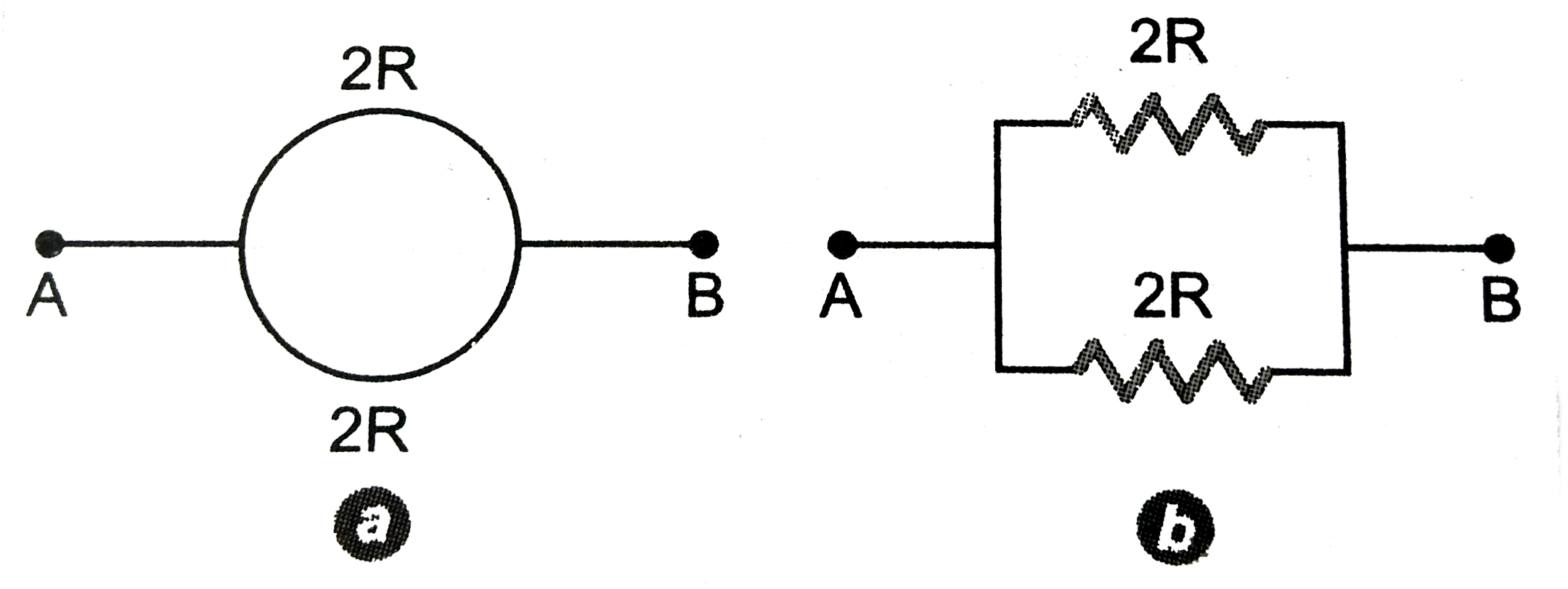
A wire of resistance 8R is bent in the form of a circle. What is the effective resistance between the ends of a diameter AB?
To find the effective resistance between the ends of a diameter AB of a wire bent in the form of a circle with a total resistance of 8R, we can follow these steps:
1. Understand the Configuration:
- The wire is bent into a circle, and we need to find the resistance between two points (A and B) that are at the ends of a diameter.
2. Divide the Circle:
- When the wire is bent into a circle, the total resistance of the wire is 8R. The wire can be thought of as being divided into two equal halves when considering the diameter AB.
- Each half of the wire will have a resistance of 8R2=4R.
3. Identify the Resistance in Parallel:
- The two halves of the wire (from A to the midpoint and from B to the midpoint) are in parallel when considering the effective resistance between A and B.
- Therefore, we have two resistances of 4R each in parallel.
4. Calculate the Equivalent Resistance:
- The formula for the equivalent resistance Req of two resistors R1 and R2 in parallel is given by:
1Req=1R1+1R2
- Here, R1=4R and R2=4R:
1Req=14R+14R=24R=12R
- Therefore, the equivalent resistance Req is:
Req=2R
5. Final Answer:
- The effective resistance between the ends of the diameter AB is 2R.
- The wire is bent into a circle, and we need to find the resistance between two points (A and B) that are at the ends of a diameter.
- When the wire is bent into a circle, the total resistance of the wire is 8R. The wire can be thought of as being divided into two equal halves when considering the diameter AB.
- Each half of the wire will have a resistance of 8R2=4R.
- The two halves of the wire (from A to the midpoint and from B to the midpoint) are in parallel when considering the effective resistance between A and B.
- Therefore, we have two resistances of 4R each in parallel.
- The formula for the equivalent resistance Req of two resistors R1 and R2 in parallel is given by:
1Req=1R1+1R2
- Here, R1=4R and R2=4R:
1Req=14R+14R=24R=12R
- Therefore, the equivalent resistance Req is:
Req=2R
- The effective resistance between the ends of the diameter AB is 2R.
Topper's Solved these Questions
- BOOK - XII BOARDS PREVIOUS YEARCHAPTER - XII BOARDSEXERCISE - SET-II35videos
XII BOARDS
- BOOK - XII BOARDS PREVIOUS YEARCHAPTER - XII BOARDSEXERCISE - SET-III25videos
XII BOARDS
- BOOK - XII BOARDS PREVIOUS YEARCHAPTER - XII BOARDSEXERCISE - SET III9videos
XII BOARDS
- BOOK - XII BOARDS PREVIOUS YEARCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION D6videos
SAMPLE PAPER 2019
Similar Questions
Knowledge Check
- Question 1 - Select One
A uniform wire of resistance 2R is bent in the form of a circle. The effective resistance between the ends of any diameter of the circle is
A2RBRCR2DR4 - Question 2 - Select One
A wire of resistance 16Ω is bent in the form of a circle. What is the effective resistance between diametrically opposite points?
A1ΩB2ΩC4ΩD8Ω - Question 3 - Select One
A Wire of resistance 12 ohm is bent in the form of a circular ring. The effective resistance between the two points on any diameter of the circle is :
A24ΩB12ΩC6ΩD3Ω
A wire of resistance 4 R is bent in the form of a circle [Fig 4.4]. What is the effective resistance between the ends of the diameter ?
 View Solution
View SolutionA wire of resistance 5 Ohms is bent in the form of a closed circle. What is the effective resistance between the two points at ends of any diameter of the circle?
 View Solution
View Solution(a) Justify the following statements
(i) Tungsten is used exclusively for filament by electric lamps
(ii) We do not use series arrangement for domestic circuits.
(b) A wire of resistance 8Ω is bent in the form of a closed circle. What is the effective resistance between two points A and B at the ends of any diameter of the circle ?View SolutionA resistor of 24Ω resistance is bent in the form of a circle as
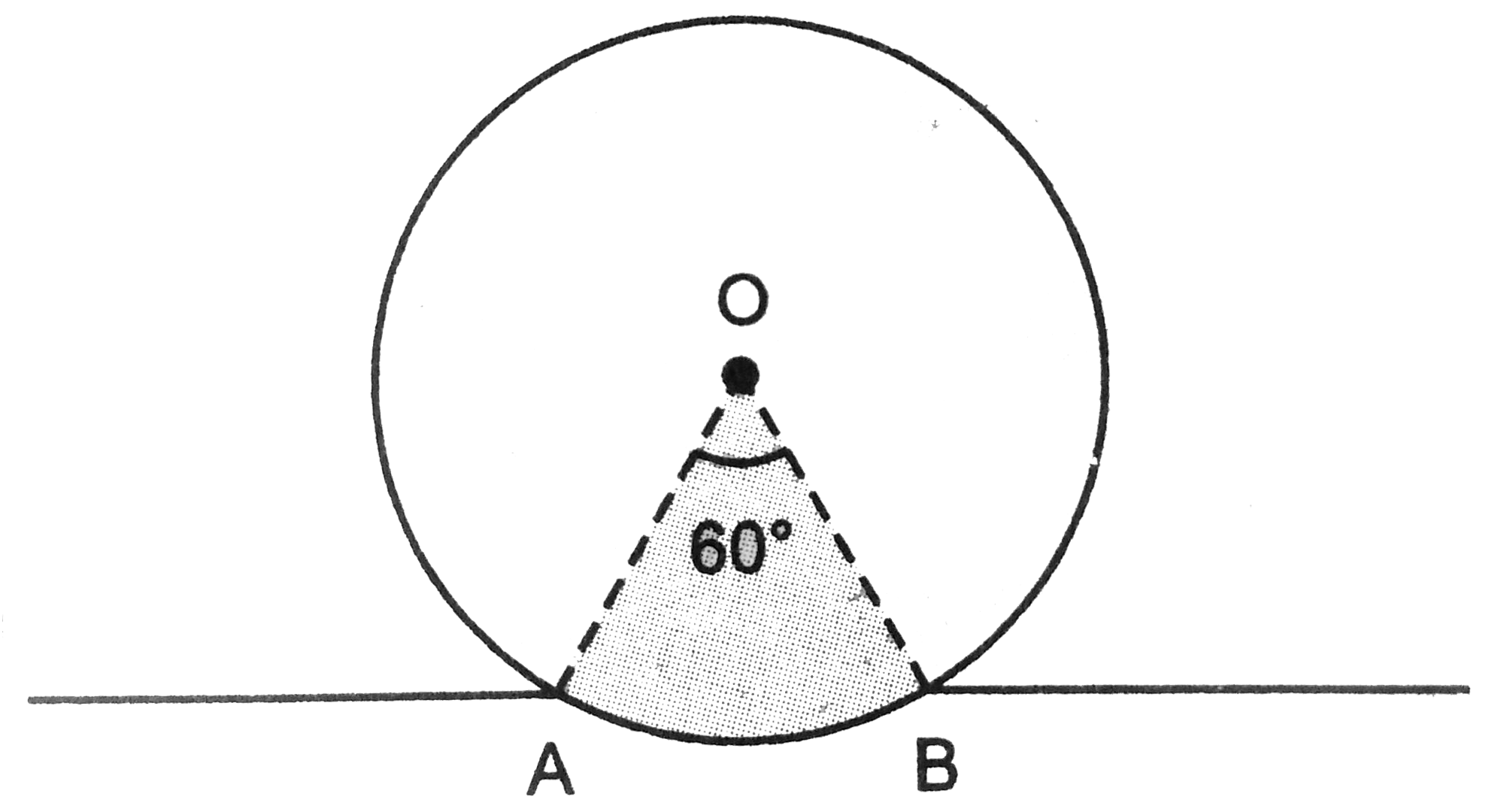 .
.
What is the effective resistance between points A and B?View SolutionA resistance of 10Ω is bent in the from of a closes circle. What is the effective resistance between the two at the ends of any diameter of this circle ?
View Solution