ABC is a triangular framwork of three uniform rods each of mass m and length 2l. It is free to rotate in its own plane about a smooth horizontal axis through A which is perpendicular to ABC. If it is released from rest when AB is horizontal and C is above AB. Find the maximum velocity of C in the subsequent motion.
IA=IAB+IAC+IBC
=43ml2+43ml2+[13ml2+m(l√3)2]
=6ml2
if ω is the angular velocity in the second position. Then using conservation of mechanical energy have

FOR COM hi=+√3l3
and hf=−2√33l
3mg(l√33)=12(ml2)ω2+3mg(−2/√33)
or ω=√g√3l
Now, velocity of C at this instant is 2lω or 2√gl√3 and maximum
Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - DC PANDEYCHAPTER - ROTATIONAL MECHANICSEXERCISE - Subjective Questions
2 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEYCHAPTER - ROTATIONAL MECHANICSEXERCISE - Level 2
1 Videos
- ROTATIONBOOK - DC PANDEYCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises
39 Videos
- ROTATIONAL MOTIONBOOK - DC PANDEYCHAPTER - ROTATIONAL MOTIONEXERCISE - Integer Type Questions
17 Videos
Similar Questions
A uniform rod of mass m and length l can rotate in a vertical plane abota smooth horizontal axis hinged at point H. Find the force exerted by the hinge just after rod is released from rest, from initial horizontal position?

View SolutionThree thin, uniform, indentical rods each or mass M and length L are joined as shown. Find the M.I. about an axis passing through O and perpendicular to the plane.
View Solution
Knowledge Check

A uniform rod of legth L is free to rotate in a vertica plane about a fixed horizontal axis through B. The rod begins rotating from rest. The angular velocity ω at angle θ is given asA√(6gL)sin(θ2)B√(6gL)cos(θ2)C√(6gL)sinθD√(6gL)cosθA right triangular plate ABC of mass m is free to rotate in the verticle plane about a fixed horizontal axis through A. It is supported by a string such that the side AB is horizontal. The reaction at the support A is
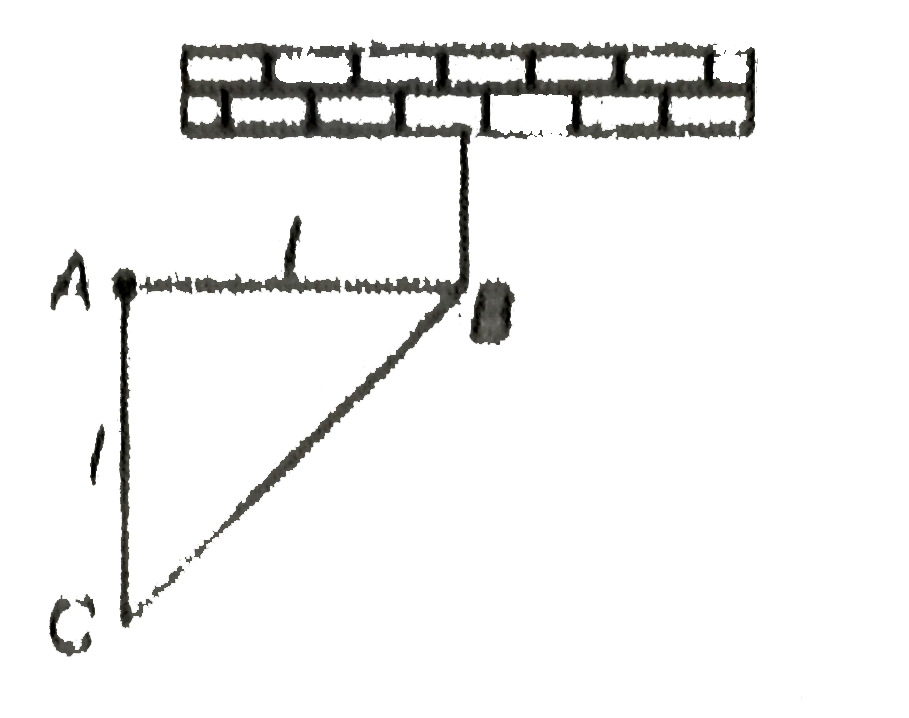 Amg3B2mg3Cmg2Dmg
Amg3B2mg3Cmg2DmgA right triangular plate ABC of mas m is free to rotate in the vertical plane about a fixed horizontal axis through A. It is supported by a string such that the side AB is horizontal. The reaction at the support A is :
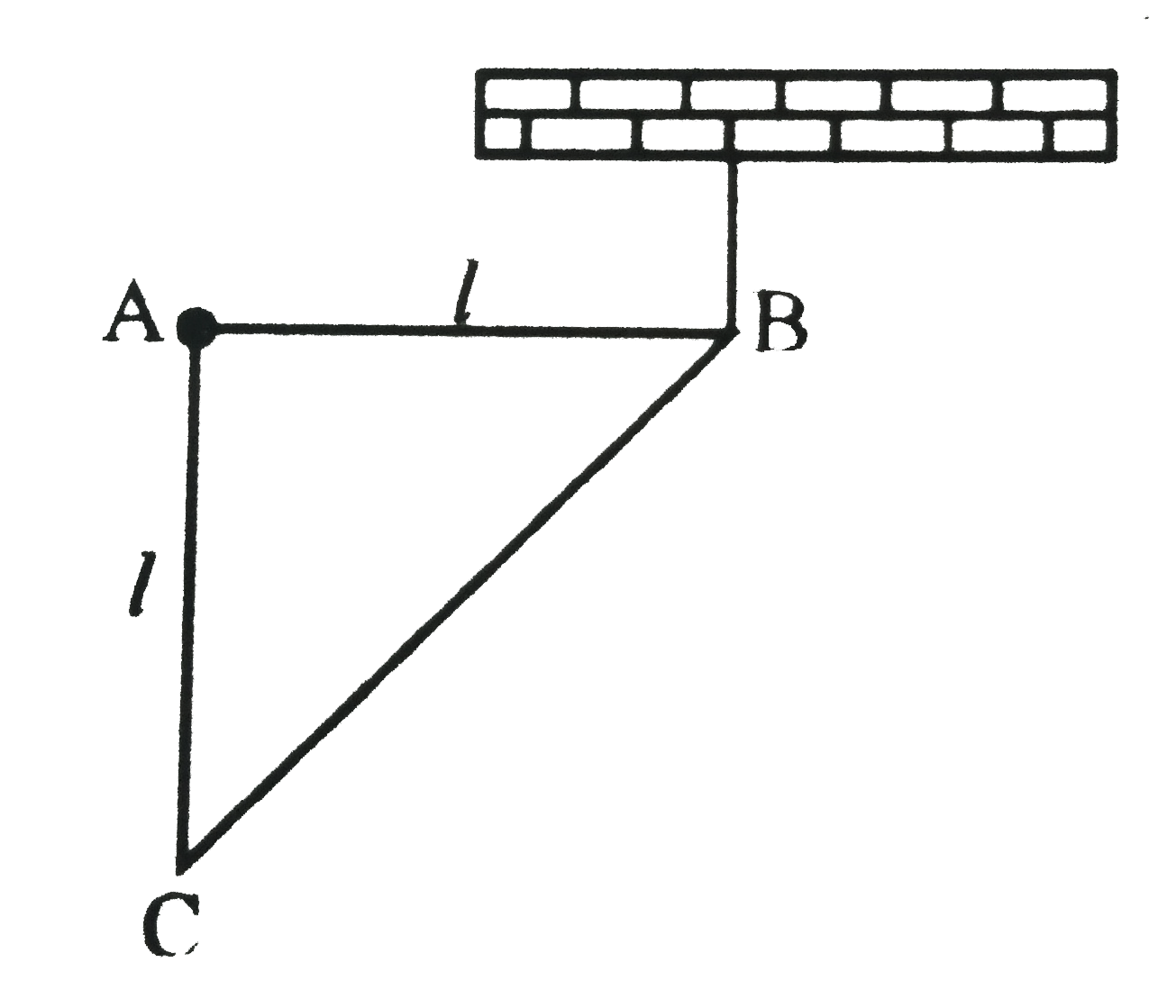 .Amg3B2mg3Cmg2Dmg
.Amg3B2mg3Cmg2Dmg
A uniform rod of mass m and length l can rotate in vertical plane about a smooth horizontal axis hinged at point H. Find angular acceleration α of the rod just after it is released from initial position making an angle of 37∘ with horizontal from rest?
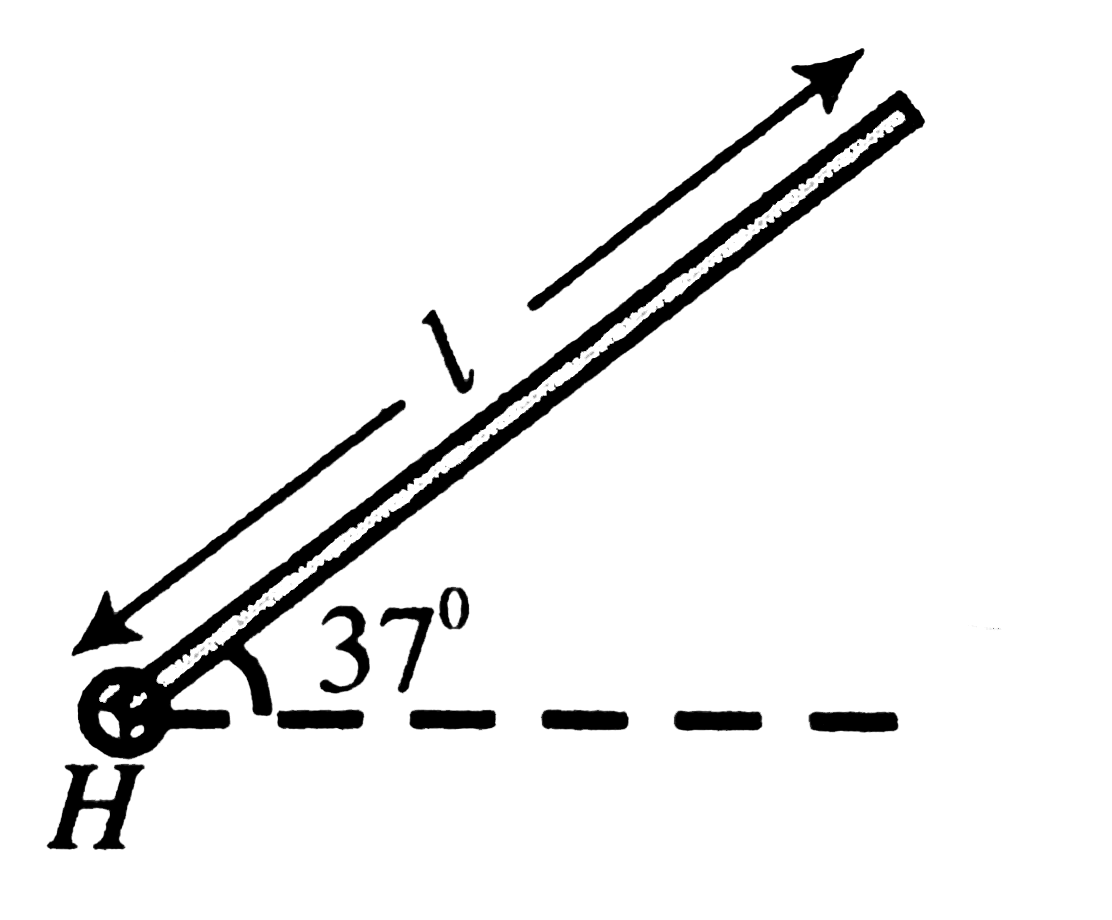
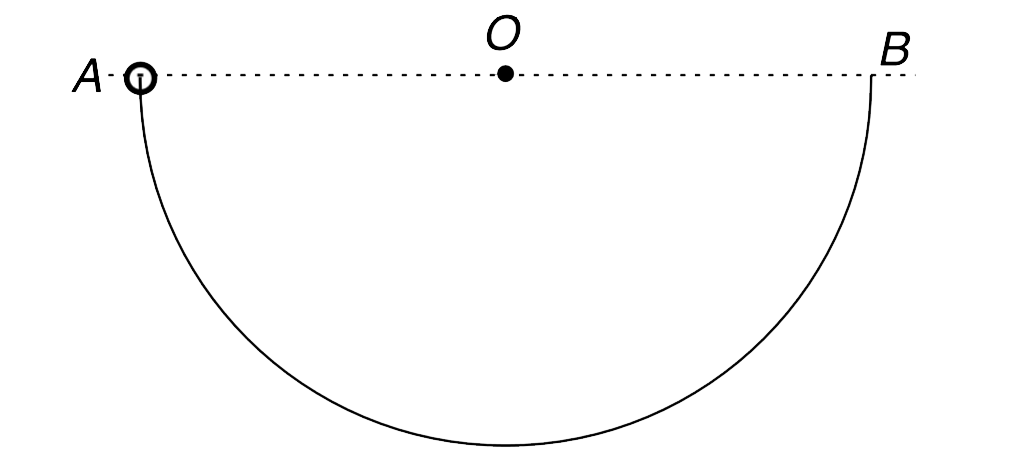
View SolutionA uniform semicircular wire is hinged at ‘A’ so that it can rotate freely in vertical plane about a horizontal axis through ‘A’. The semicircle is released from rest when its diameter AB is horizontal Find the hinge force at ‘A’ immediately after the wire is released.
View SolutionA uniform rod of mass m and length l can rotate in a vertical plane about a smooth horizontal axis point H. a. Find angular acceleration α of the rod. just after it is released from initial horizontal position from rest'? b. Calculate the acceleration (tangential and radial) , point A at this moment.
View SolutionA uniform rod AB of mass 3m and length 4l, which is free to turn in a vartical plane about a smooth horizontal axis through A, is released from rest when horizontal When the rod first impulse exerted by the peg on the rod it.
(a). The rod is brought to rest by the peg.
(b). The rod rebounds and next comes to instantaneous rest inclined to the downward vertical at an angle π3 radian.
View SolutionA uniform rod of mass m and length l rotates in a horizontal plane with an angular velocity ω about a vertical axis passing through one end. The tension in the rod at a distance x from the axis is
View Solution
