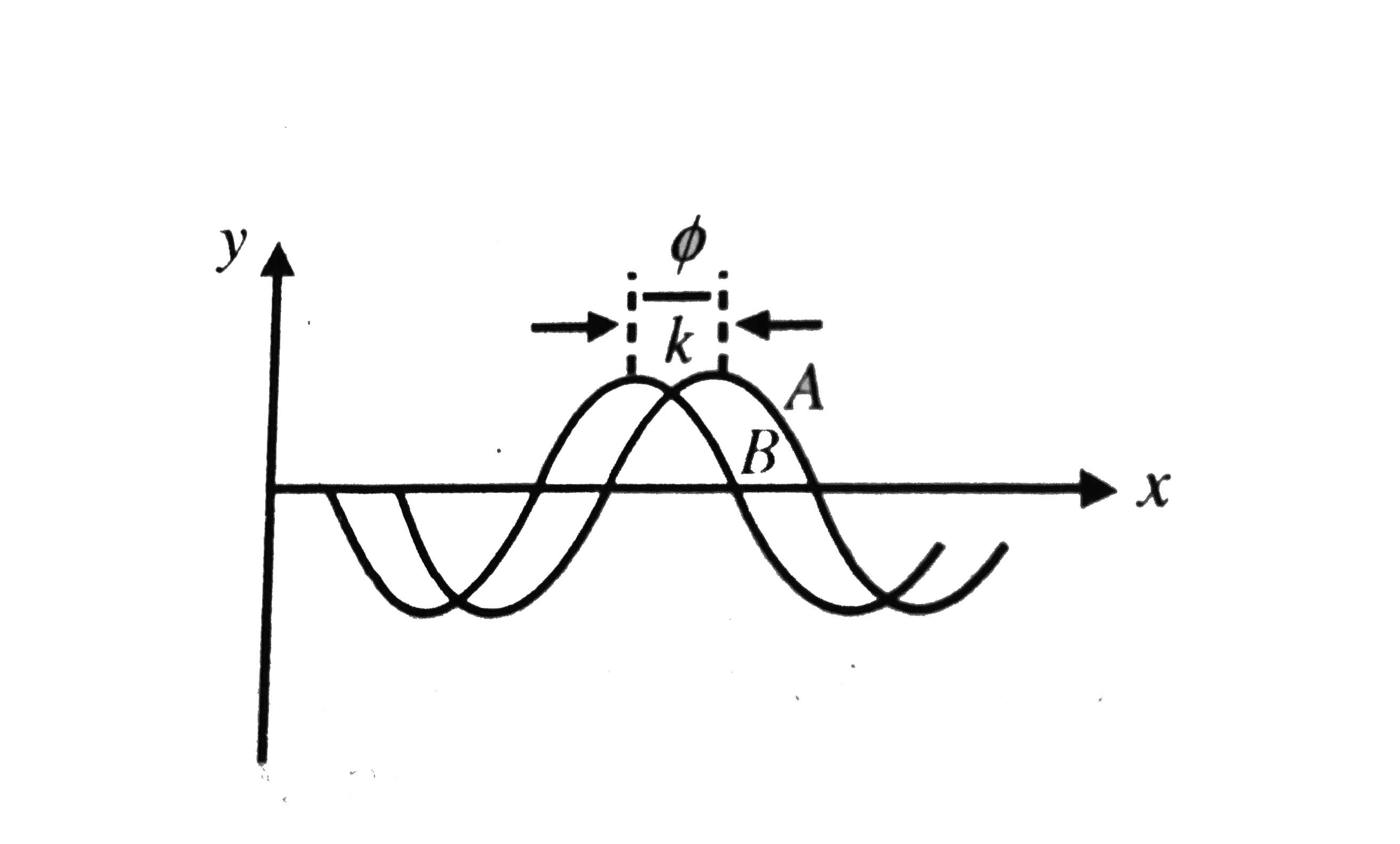
Adjoining figure shows the snapshot of two waves A and B at any time t. the equation for A is y=Asin(kx−ωt−ϕ), and for B it is y=Asin(kx−ωt). It is clearly shown in the figure that wave A is ahead of B by a distance ϕ/k.

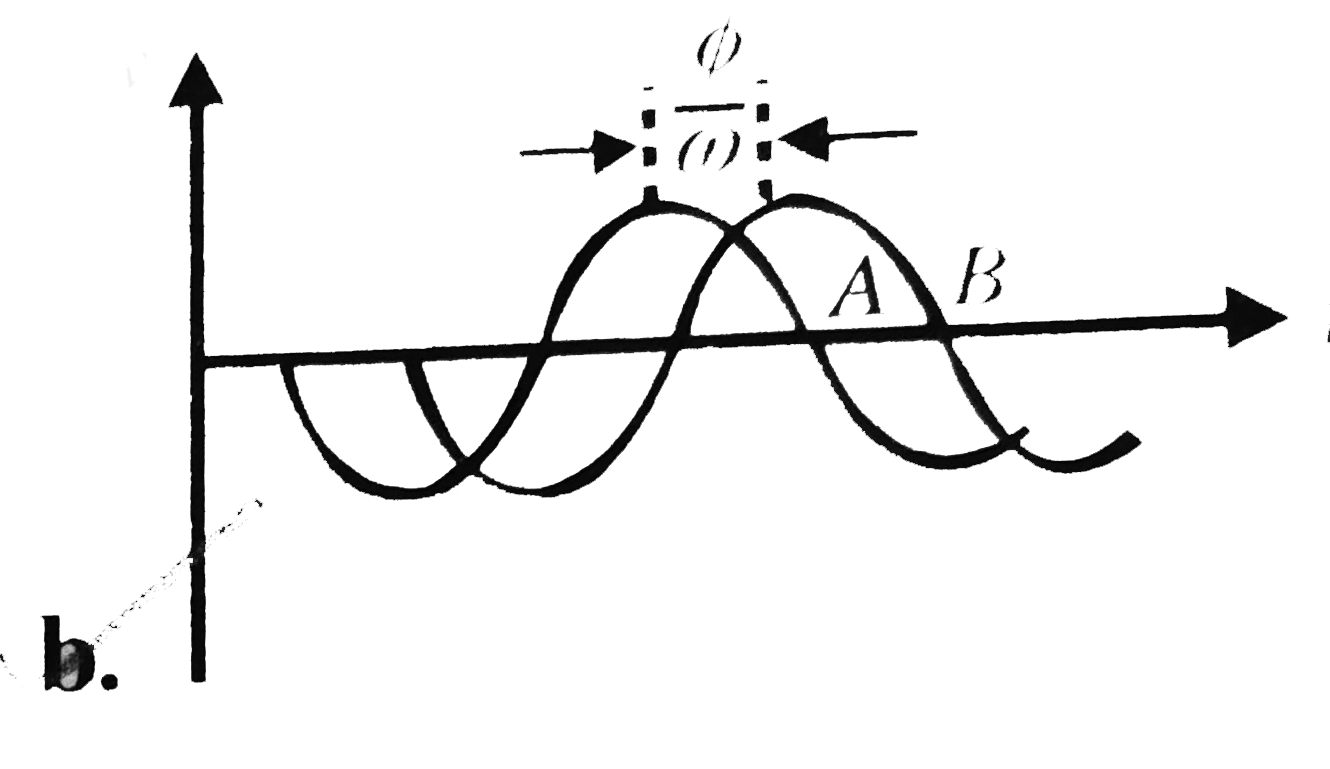


The correct Answer is:b
The equation for wave A can be rewritten as
y=Asin[kx−ωt−ϕ]
=Asin[k(x−ϕ/k)−ωt]
Asin[kx−ω(t+ϕk)]
while equation of wave B is y=Asin(kx−ωt).
Comparing above equation, we can easily conclude that A is at a distance ahead of ϕ/k from B or wave A is ahead of B by a time difference of ϕ/ω.So, (b) is the correct option.
Remember ! In y versust'ahead of means to the left of' while in y versus x 'ahead of means to the right of' if the wave travels in positive x−direction and vice versa.
Topper's Solved these Questions
- TRAVELLING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Single Correct Answer
1 Videos
- TRAVELLING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Multiple Correct
25 Videos
- TRAVELLING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Subjective
16 Videos
- TRANSMISSION OF HEATBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRANSMISSION OF HEATEXERCISE - Single correct
9 Videos
- VECTORSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
Figure shows a snapshot for a travelling wave. The equation of wave at t=0 will be
_PHY_E06_334_Q01.png)
View SolutionThe equation y=Asin2(kx−ωt) represents a wave motion with
View SolutionIf there are equations of two waves y=Asin(ωt−kx) and z=Asin(ωt−kx), then on superposition of the two waves, the amplitude of resultant wave is
View SolutionIn a wave motion y=asin(kx−ωt),y can represent :-
View SolutionTwo sinusoidal waves are superposed. Their equations are
y1=Asin(kx−ωt+π6)andy2=Asin(kx−ωt−π6)
the equation of their resultant is
View SolutionThe equation of a light wave is written as y=Asin(kx−ωt). Here y represents
View SolutionA wave is propagating in positive x−direction. A time t=0 its snapshot is taken as shown. If the wave equation is y=Asin(ωt−Kx+ϕ), then ϕ is
_2019_CBT7_E01_013_Q01.png)
View SolutionA wave is propagating in positive x−direction. A time t=0 its snapshot is taken as shown. If the wave equation is y=Asin(ωt−Kx+ϕ), then ϕ is
_2019_CBT7_E01_013_Q01.png)
View SolutionA progressive wave travelling along the positive x-direction is represented by y(x,t)=Asin(kx−ωt+ϕ). Its snapshot at t=0 is given in the figure.
For this wave, the phase is:
View SolutionA progressive wave travelling along the positive x-direction is represented by y(x,t)=Asin(kx−ωt+ϕ). Its snapshot at t=0 is given in the figure.
For this wave, the phase is:

View Solution
