An air masked eye placed inside water refractive index, μ sees the outside world. The vertex angle of the cone in which the eye will see the outside world is
2sin−1(1/μ)
2cos−1(1/μ)
2sin−1√(μ2−1)
2cos−1√(μ2−1)
The correct Answer is:A

μsinC=1×sin90∘
sinC=1/μ⇒C=sin−1(1/μ)
Vertex angle =2C=2sin−1(1/μ)
Topper's Solved these Questions
- REFRACTION OF LIGHT BY PLANE SURFACESBOOK - CP SINGHCHAPTER - REFRACTION OF LIGHT BY PLANE SURFACESEXERCISE - Exercises
110 Videos
- REFRACTION AT SPHERICAL SURFACESBOOK - CP SINGHCHAPTER - REFRACTION AT SPHERICAL SURFACESEXERCISE - EXERCISES
105 Videos
- SEMICONDUCTORSBOOK - CP SINGHCHAPTER - SEMICONDUCTORSEXERCISE - Exercises
140 Videos
Similar Questions
The refractive index of water is 4/2. Determine the angle of the cone within which the entire outside view will be confined for a fish under water.
View SolutionThe refractive index of water is 4/3. Determine the angle of the cone within which the entire outside view will be confined for a fish under water.
View Solution
Knowledge Check
For an eye kept at a depth h inside water is refractive index μ, and viewed outside, the radius of circle through which the outer objects can be seen will be
Ah√μ2−1BhμCh√μ2+1D(√μ2−1μ)hThe refractive index of water is 1.33. The direction in which a man under water should look to see the setting sun is
A49 to the horizantalB90 with the verticalC49 to the verticalDAlong the horizontalA ray of light is incident on the hypotenuse of a right-angled prism after travelling parallel to the base inside the prism. If μ is the refractive index of the material of the prism, the maximum value of the base angle for which light is totally reflected from the hypotenuse is
Asin−1(Iμ)Btan−1(1μ)Csin−1(μ−1μ)Dcos−1(1μ)
A thin equiconvex glass lens (μg=1.5) is beign placed on the top of a vessel of height h=20cm as shown figure. A luminous point source is beign placed at the bottom of vessel on the principal axis of the lens. When the air is on both the side of the lens the image of luminous source is formed at a distance of 20cm from the lens out side the vessel. When the air inside the vessel is being replaced by a liquid of refractive index μl the image of the same source is being formed at a distance 30cm from the lens outside the vessel. Find the μl.
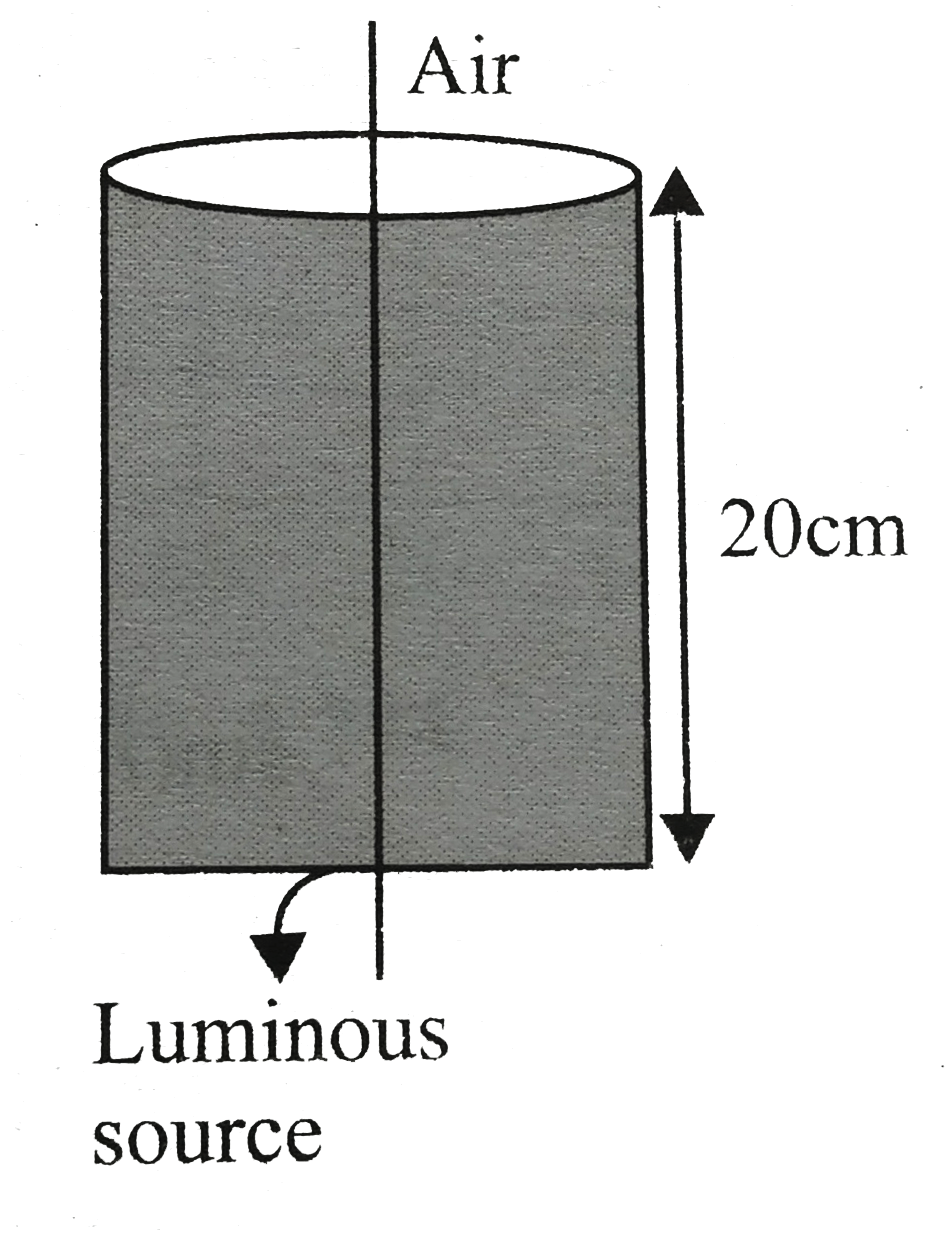
View SolutionA fish in a water tank sees the outside world as if it (the fish) is at the vertex of cone such that the circular base of the cone coincides with the surface of water. Given the depth of water where fish is located being h and the critical angle for water - air interface being iC, find out by drawing a suitable ray diagram the relationship between the radius of the cone and the height h.
View SolutionA fish in a water tank sees the outside world as if it (the fish) is at the vertex of cone such that the circular base of the cone coincides with the surface of water. Given the depth of water where fish is located being h and the critical angle for water-air interface being ic, find out by drawing a suitable ray diagram the relationship between the radius of the cone and the height h.
View SolutionThere is a small air bubble at the centre of a solid glass sphere of radius 'r' and refractive index μ. What wil be the apparent distance of the bubble from the centre of the sphere, when viewed from outside?
View SolutionWhen the surface of a lake is calm , a fish submerged in water will see the entire outside world within an inverted cone whose vertex is situated at the eye of the fish . What is the vertex angle of the cone ?
(anw=43) and sin49∘=0.75
View Solution
