An aluminium wire of cross-sectional area (10−6)m2 is joined to a steel wire of the same cross-sectional area. This compound wire is stretched on a sonometer pulled by a weight of 10kg. The total length of the compound wire between the bridges is 1.5m of which the aluminium wire is 0.6m and the rest is steel wire. Transverse vibrations are setup in the wire by using an external source of variable frequency. Find the lowest frequency of excitation for which the standing waves are formed such that the joint in the wire is a node. What is the total number of nodes at this frequency? The density of aluminium is 2.6×(103)kg/m3 and that of steel is 1.04×104kg/m2(g=10m/s2)
Now as in case of composite wire, the whole wire will vibrate with fundamental frequency.
f=nAfA=ncfc
nA2×0.6√TA×2.6×10−3=nc2×0.9√TA×1.0401×104
i.enAne=23√2.610.4=23×12=13
So that for fundamental frequency of composite string, n = 1 and n = 3, i.e., aluminium string will vibrate in first harmonic and copper wire at second, overtone as shown in figure.
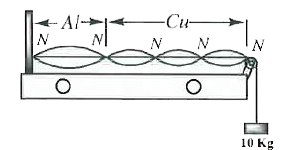
∴f=fA=3fc
This in turn implies that total number of nodes in the string will be 5 and so number of nodes excluding the nodes at the ends =5-2=3 and
f=fA=12×0.6√0×9.810−6×2.6×103≈161.8Hz(=3fc)
Topper's Solved these Questions
- WAVESBOOK - AAKASH SERIESCHAPTER - WAVESEXERCISE - EXERCISE-IA (Theoretical questions :)
85 Videos
- WAVESBOOK - AAKASH SERIESCHAPTER - WAVESEXERCISE - EXERCISE-IA (Statement type questions:)
18 Videos
- WAVE OPTICSBOOK - AAKASH SERIESCHAPTER - WAVE OPTICSEXERCISE - PROBLEMS (LEVEL - II)
33 Videos
- WAVES OPTICSBOOK - AAKASH SERIESCHAPTER - WAVES OPTICSEXERCISE - EXERCISE -III (POLARISITION)
10 Videos
Similar Questions
A wire of cross-sectional area A breaks due to its own weight when length of the wire is l. If area of cross-section of the wire is made 3A then maximum length of the wire can be hung without breaking is
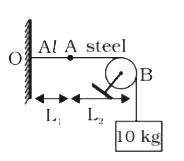
View SolutionAn aluminium wire and a steel wire of same cross-sectional of 10−2cm2 were connected together. A 10 kg block loaded on the compound wire as shown in figure. Waves are set up in the compound wire. The minimum frequency of excitation for which standing waves are observed with point A as a node is [L1=60cm,L2=86.6cm] density of aluminium =2.6g/cm3 and of steel=7.8g/cm3]
View Solution
Knowledge Check
A wire of length L and cross-sectional area A is made of a material of Young's modulus Y. IF the wire is stretched by an amount x, the workdone is
AYAx22LBYAx2L2CYAx2LDYAx2LA wire of length L and area of cross-section A, is stretched by a load. The elongation produced in the wire is I. If Y is the Young's modulus of the material of the wire, then the force constant of the wire is
AYLABYlACYALDYAl
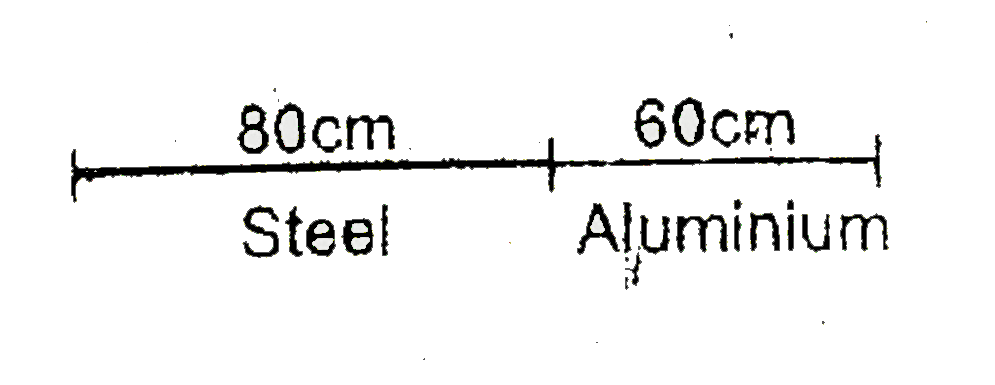
Figure shows an aluminium wire of length 60cm joined to a steel wire of length 80cm and stretched between two fixed supports. The tension produced is 40N. The cross-sectional area of the steel wire is 1.0mm2 and that of the aluminimum wire is 3.0mm2 The minimum frequency of a tuning fork which can produce standing waves in the system with the joint as a node is 10P (in Hz) the find P. Given density of aluminimum is 2.6g/cm3 and that of steel is 7.8g/cm3.
View SolutionA sonometer wire is under a tension of 10 N and the length between the bridges is 2m. A metre long wire of sonometer has mass of 1.0 gm. Deduce the speed of transverse wave and the frequency of 1st hormonic.
View SolutionA steel wire of length 50√3cm is connected to an aluminium wire of length 60cm and stretched between two fixed supports. The tension produced is 104N, if the cross section area of each wire is 1mm2. If a transverse wave is set up in the wire, find the lowest frequency for which standing waves with node at the joint are produced . (density of aluminimum =2.6gm/cm3 and density of steel =7.8gm/cm3).
View SolutionA steel wire of length 2L and cross-section area A is stretched, wetl within elastic limit horizontally between two pillars, a mass m is suspended from the mid-point of wire such that depression at the middle is x. Strain in the wire is proportional to
View SolutionTwo wires of different densities but same area of cross section are soldered together at one end and are stretched to a tension T. The velocity of a transverse wave in the first wire is double of that in the second wire. Find the ration of the denstiy of the first wire to that of the second wire.
View SolutionTwo wires of different densities but same area of cross-section are soldered together at one end and are stretched to a tension T. The velocity of a transverse wave in the first wire is double of that in the second wire. Find the ratio of density of the first wire to that of the second wire.
View Solution
