An arm making an angle of 120∘ at the center of ring of mass m and radius r is cut from the ring. The arc is made to rotate about z-axis perpendicular to its plane and passing through the center of the ring. The moment of inertia of the arc about the z-axis is
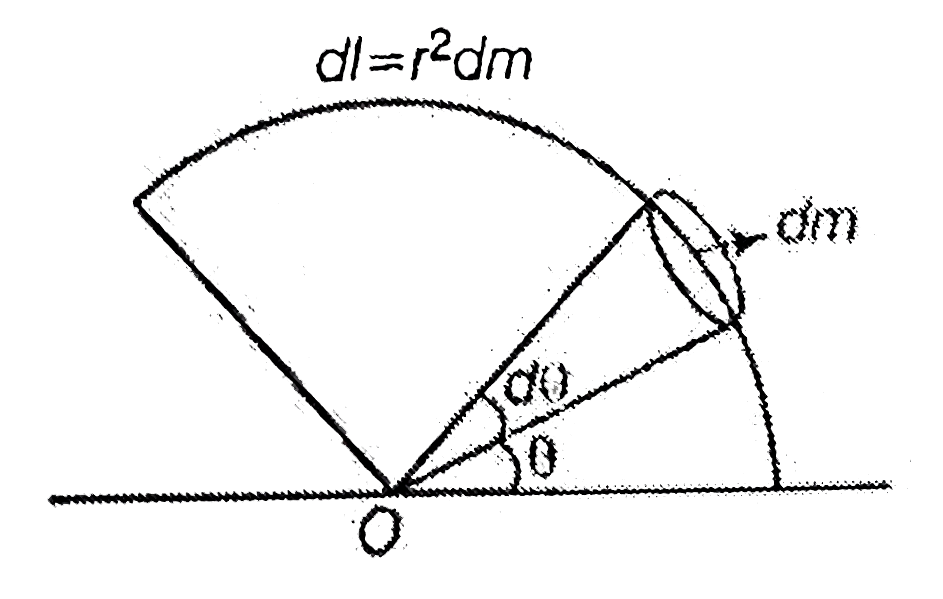
mr2
mr23
mr22
(mr24
b) The mass of ring of per unit angle is λ=m2π
∴ dm-= (m)/(2pi) dtheta<br>thereforeThemomentof∈ertiaofconsrede≤mentaboutz−aξspassingthroughp∮Ois<br>dl=r^(2)dm<br>thereforethemomentof∈ertiaofthearcis<br>I = r^(2) = (mr^(2)/3 int_(theta=0)^(theta=(2pi//3)))dm=(mr^(2)/3) 
Topper's Solved these Questions
- BOOK - BITSAT GUIDECHAPTER - ROTATIONAL MOTIONEXERCISE - BITSAT Archives9videos
ROTATIONAL MOTION
- BOOK - BITSAT GUIDECHAPTER - NEWTONS LAWS OF MOTION AND FRICTIONEXERCISE - BITSAT Archives23videos
NEWTONS LAWS OF MOTION AND FRICTION
- BOOK - BITSAT GUIDECHAPTER - SCALARS AND VECTORSEXERCISE - All Questions34videos
SCALARS AND VECTORS
Similar Questions
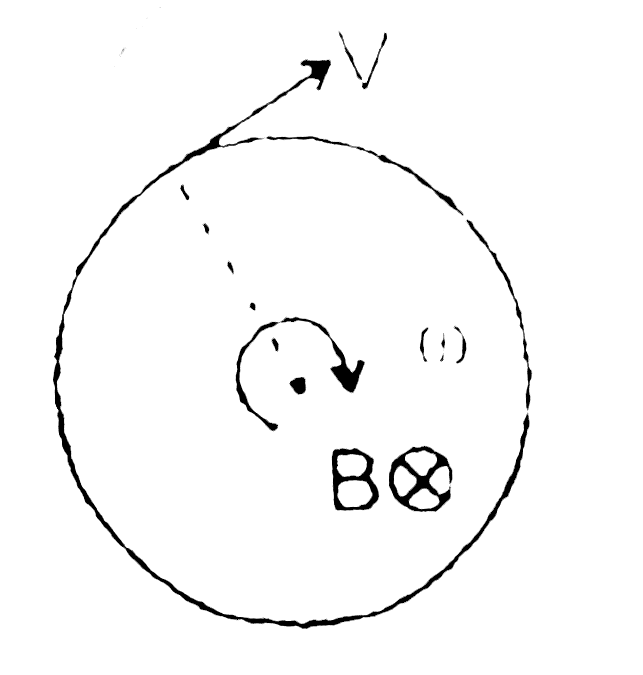
A ring rotates with angular velocity ω about an axis perpendicular to the plane of the ring passing through the center of the ring.A constant magnetic field B exists parallel to the axis.Find the emf induced in the ring.
 View Solution
View SolutionA circular ring of radius 10cm is made of a wire of mass 0.02g/cm. Calculate its radius of gyration and moment of inertia about an axis passing perpendicular to its plane through the centre of the ring.
View Solution
Knowledge Check
- Question 1 - Select One
A wire of mass m and length l is bent in the form of circular ring. The moment of inertia of the ring about its axis is
AMl4π2BMl2πCMl4πDMl24π2 - Question 2 - Select One
One quarter sector is cut from a uniform circular disc of radius R. This sector has mass M. it is made to rotate about a line perpendicular to its plane and passing through the center of the original disc. Its moment of inertia about the axis of rotation is
 A12MR2B14MR2C18MR2D√2MR2
A12MR2B14MR2C18MR2D√2MR2 - Question 3 - Select One
There is a ring or mass m and radius R is pivoted at a point O on its periphery. It is free to rotate about an axis perpendicular to its plane. What is the period of ring?
AT=2π((Rg))BT=2π√(2Rg)CT=π√(2Rg)DT=2π√(3Rg)
Calculate the moment of inertia of a ring of mass 2 kg and radius 50 cm about an axis passing through its centre and perpendicular to its plane
View SolutionA wire of mass m and length l is bent in the form of circular ring. The moment of inertia of the ring about its axis is
View SolutionWhat is the ratio of the moments of inertia of two rings radii r and nr about an axis perpendicular to their plane and passing through their centres?
View SolutionA wire of mass 'm' and length 'l' is bent in the form of a circular ring. The moment of inertia of the ring about its axis is-
View SolutionFrom a complete ring of mass M and radius R, a 30∘sector is removed. The moment of inertia of the incomplete ring about an axis passing through the centre of the ring and perpendicular to the plane of the ring is
 ,View Solution
,View Solution

