What is the ratio of the moments of inertia of two rings radii r and nr about an axis perpendicular to their plane and passing through their centres?
1:n2
1:n
1:2n
n2:1
The correct Answer is:A
I1I2=Mr2m(nr)2=1:n2
Topper's Solved these Questions
- ROTATIONAL MOTION Book:CBSE COMPLEMENTARY MATERIALChapter:ROTATIONAL MOTION Exercise:ROTATIONAL MOTION (5 MARKS)
Explore 2 Videos
- PROPERTIES OF MATTERBook:CBSE COMPLEMENTARY MATERIALChapter:PROPERTIES OF MATTERExercise:MULTIPLE CHOICE QUESTIONS (MCQs)
Explore 20 Videos
- THERMODYNAMICS Book:CBSE COMPLEMENTARY MATERIALChapter:THERMODYNAMICS Exercise:MULTIPLE CHOICE QUESTIONS (MCQs)
Explore 20 Videos
Similar Questions
If I1 is the moment of inertia of a thin rod about an axis perpendicular to its length and passing through its centre of mass and I2 is the moment of inertia of the ring about an axis perpendicular to plane of ring and passing through its centre formed by bending the rod, then
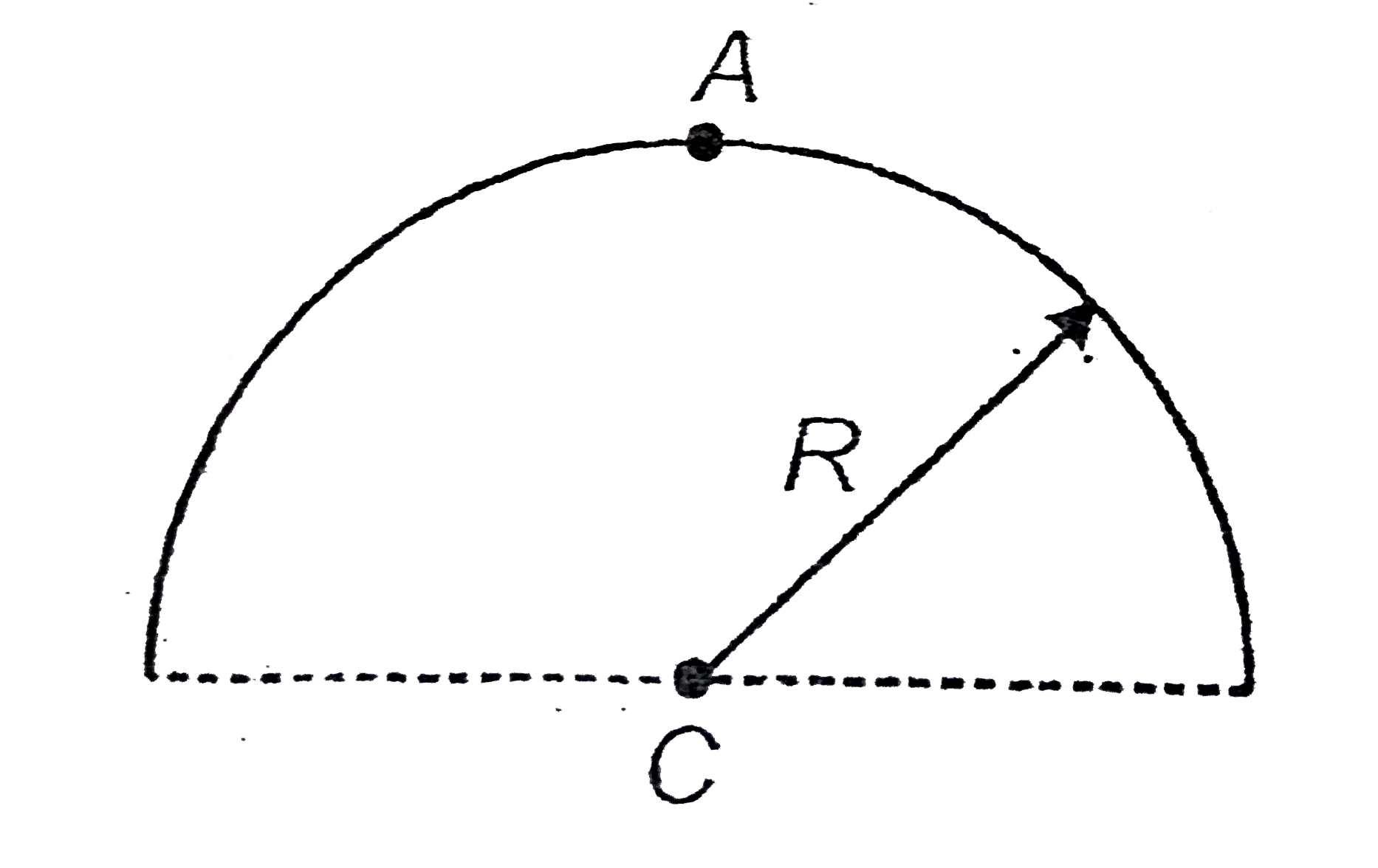
View SolutionMoment of inertia of a half ring of mass m and radius R about an axis passing through point A perpendicular to the plane of the paper is IA. If IC is the moment of inertia of the ring about an axis perpendicular to the plane of paper and passing through point C, then
View Solution
Knowledge Check
The moment of inertia of NaCl molecule with bond length r about an axis perpendicular to the bond and passing through the centre of mass is
A(mNa+mCI)r2BmNa+mCImNa−mCIr2CmNa×mCImNa+mCIrDmNa+mCImNa−mCIr2Moment of inertia of a thin circular plate of mass M, radius R about an axis passing through its diameter is I. The moment of inertia of a circular ring of mass M, radius R about an axis perpendicular to its plane and passing through its centre is
A2IBI2C4IDI4If I moment of inertia of a thin circular plate about an axis passing through tangent of plate in its plane. The moment of inertia of same circular plate about an axis perpendicular to its plane and passing through its centre is
A4I5B2I5C4I3D2I3
Moment of inertia of a half ring of mass m and radius R about an axis passing through point A perpendicular to the plane of the paper is IA. If IC is the moment of inertia of the ring about an axis perpendicular to the plane of paper and passing through point C, then

View SolutionFind the moment of inertia of a uniform half-disc about an axis perpendicular to the plane and passing through its centre of mass. Mass of this disc is M and radius is R.
View SolutionI is moment of inertia of a thin square plate about an axis passing through opposite corners of plate. The moment of inertia of same plate about an axis perpendicular to the plane of plate and passing through its centre is
View SolutionI is moment of inertia of a thin circular ring about an axis perpendicular to the plane of ring and passing through its centre. The same ring is folded into 2 turns coil. The moment of inertia of circular coil about an axis perpendicular to the plane of coil and passing through its centre is
View SolutionIf I1 is the moment of inertia of a thin rod about an axis perpendicular to its length and passing through its centre of mas and I2 is the moment of inertia of the ring about an axis perpendicular to plane of ring and passing through its centre formed by bending the rod, then
View Solution
