An electron enters a region of uniform electric field with an initial velocity of 30 km/s in the same direction as the electric field, which has magnitude E = 50 N/C. (a) What is the speed of the electron 1.5 ns after entering this region? (b) How far does the electron travel during the 1.5 ns interval ?
To solve the problem step by step, we will break it down into parts (a) and (b) as per the question.
Given Data:
- Initial velocity of the electron, u=30km/s=30×103m/s
- Electric field strength, E=50N/C
- Charge of the electron, q=−1.6×10−19C
- Mass of the electron, m=9.1×10−31kg
- Time interval, t=1.5ns=1.5×10−9s
Part (a): Find the speed of the electron after 1.5 ns.
1. Calculate the force acting on the electron:
F=qE
Since the charge of the electron is negative, the force will act in the opposite direction to the electric field.
F=(−1.6×10−19C)(50N/C)=−8.0×10−18N
2. Calculate the acceleration of the electron:
a=Fm=−8.0×10−18N9.1×10−31kg≈−8.79×1012m/s2
(The negative sign indicates that the acceleration is opposite to the direction of the initial velocity.)
3. Use the kinematic equation to find the final velocity:
v=u+at
Substituting the values:
v=(30×103m/s)+(−8.79×1012m/s2)(1.5×10−9s)
v=30×103−13.185≈16.815×103m/s
Part (b): Find the distance traveled by the electron during the 1.5 ns interval.
1. Use the kinematic equation to find the distance:
s=ut+12at2
Substituting the values:
s=(30×103m/s)(1.5×10−9s)+12(−8.79×1012m/s2)(1.5×10−9s)2
s=45×10−6−0.5×8.79×1012×2.25×10−18
s=45×10−6−0.5×8.79×2.25×10−6
s≈45×10−6−9.86×10−6≈35.14×10−6m
Final Answers:
(a) The speed of the electron after 1.5 ns is approximately 16.815km/s.
(b) The distance traveled by the electron during the 1.5 ns interval is approximately 35.14μm.
- Initial velocity of the electron, u=30km/s=30×103m/s
- Electric field strength, E=50N/C
- Charge of the electron, q=−1.6×10−19C
- Mass of the electron, m=9.1×10−31kg
- Time interval, t=1.5ns=1.5×10−9s
F=qE
Since the charge of the electron is negative, the force will act in the opposite direction to the electric field.
F=(−1.6×10−19C)(50N/C)=−8.0×10−18N
a=Fm=−8.0×10−18N9.1×10−31kg≈−8.79×1012m/s2
(The negative sign indicates that the acceleration is opposite to the direction of the initial velocity.)
v=u+at
Substituting the values:
v=(30×103m/s)+(−8.79×1012m/s2)(1.5×10−9s)
v=30×103−13.185≈16.815×103m/s
s=ut+12at2
Substituting the values:
s=(30×103m/s)(1.5×10−9s)+12(−8.79×1012m/s2)(1.5×10−9s)2
s=45×10−6−0.5×8.79×1012×2.25×10−18
s=45×10−6−0.5×8.79×2.25×10−6
s≈45×10−6−9.86×10−6≈35.14×10−6m
(a) The speed of the electron after 1.5 ns is approximately 16.815km/s.
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDSBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Practice Questions (Single Correct Choice Type)
45 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Practice Questions (More than one correct Type )
3 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Check points
9 Videos
- ELASTICITYBOOK - RESNICK AND HALLIDAYCHAPTER - ELASTICITYEXERCISE - PRACTICE QUESTIONS (Integer Type)
3 Videos
- ELECTRIC POTENTIALBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - (PRACTICE QUESTIONS) Integer Type
1 Videos
Similar Questions
An electron is released from rest in a uniform electric field of magnitude 2.00×104N/C . (a) Calculate the acceleration of the electron. (Ignore gravitational) (b) How much time does the electron take to reach 1.00 % of the speed of light ?
View SolutionAn electron is accelerated in an electric field of intensity E=3.0×106 N /C. Find the speed of the electron after 1.0 ns. What would be the speed of the electron, if 'its mass is independent of speed?
View Solution
Knowledge Check
A uniform electric field and a uniform magneitc field exist in a region in the same direction An electron is projected with velocity pointed in the same direction the electron will
Aturn to its rightBturn to its leftCkeep moving in the same direction but its speed will increaseDkeep moving in the same direction but its speed will decreaseAn electron enters a region where magnetic field (B) and electric field (E ) are mutually perpendicular, then
Ait will always move in the direction of BBit will always move in the direction of ECit always possess circular motionDit can go undeflected alsoIn a uniform magnetic field of 5T, an electron is moving with constant velocity of 15m/s. The force on the electron is
A75NB2.4×10−18NC1Dzero
An electron moving in a gravitational free space enters a unifrom electric field E with an initial velocity shown in fig.
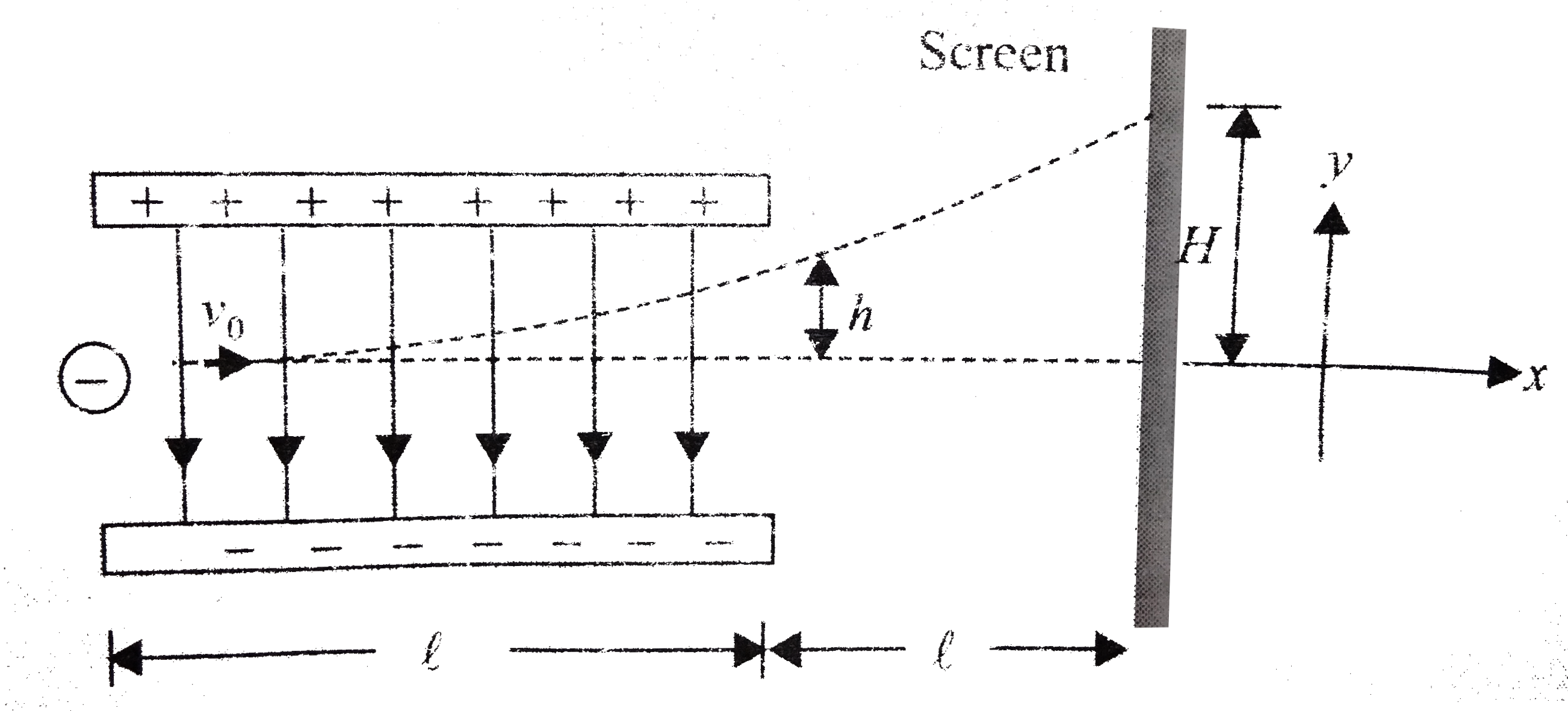 .
.
(a) Find the deflection distance h in the field .
(b) Find an expression for the velocity of electron when it just emerage from the field.
(c ) Find an expression for the total deflection distance H at a vertical screen placed at a distance l from the region of uniform field .(Assume that the field abruptly ends outside the field. ).
View SolutionA uniform electric field and a uniform magnetic field exist in a region in the same direction. An electron is projected with a velocity pointed I the same direction. Then the electron will:
View SolutionA uniform electric field and a uniform magnetic field are acting along the same direction in a certain region. If an electron is projected in the same direction then the electron
View SolutionAn electron traveling in a uniform electric field passes from a region of potential V1 to a region of higher potential V2. Then

View SolutionA mass m=20g has a charge q=3.0mC. It moves with a velocity of 20 m/s and enters a region of electric field of 80 N/C in the same direction as the velocity of the mass. The velocity of the mass after 3 s in this region is
View Solution
