An ideal gas undergoes a thermodynamic process as shown in Fig. The process consists of two isobaric and two isothermal steps. Show that the network done during the whole process is
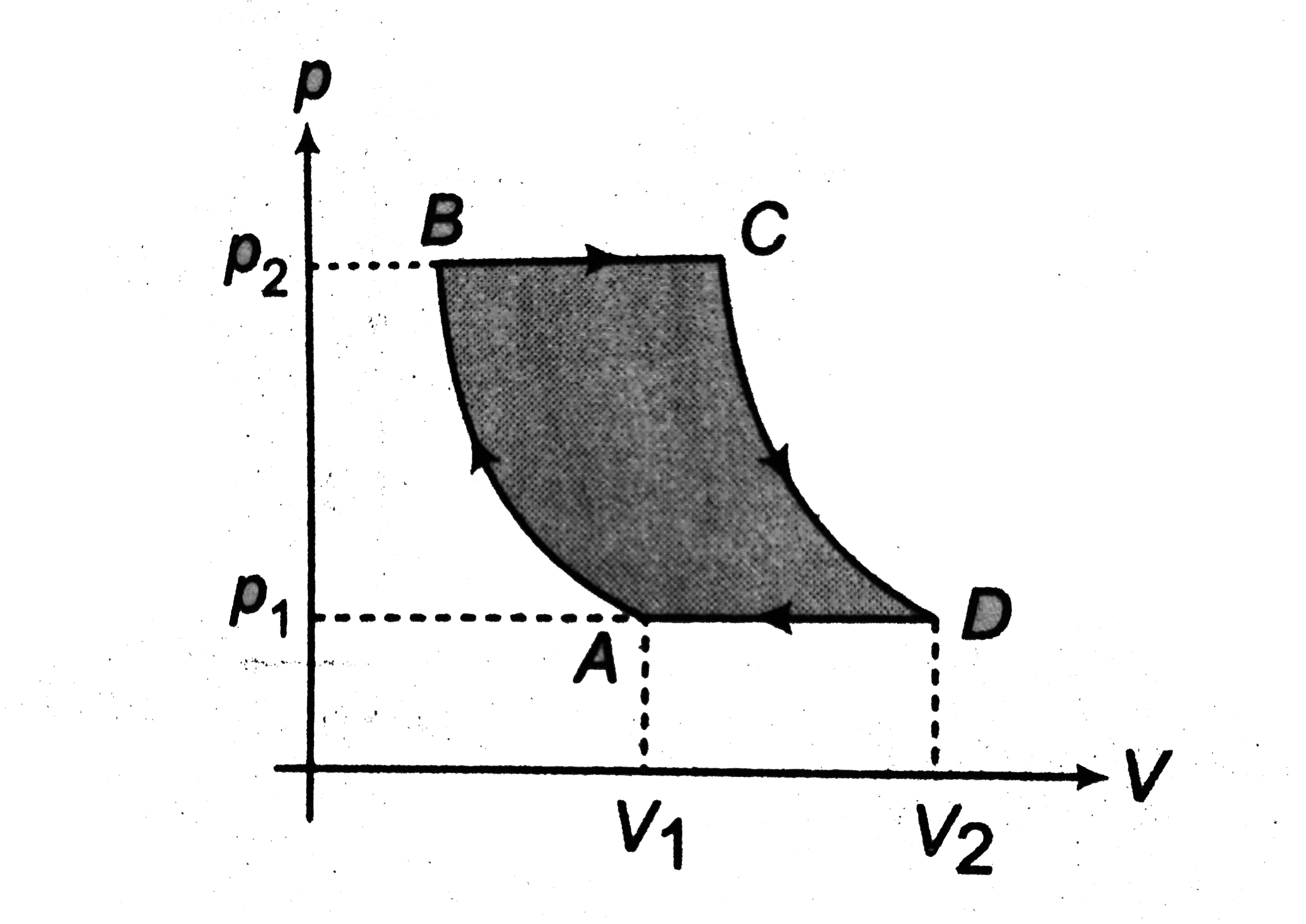
W(Net)=P1(V2−V1)logeP2P1
Wnct=P2(VB−VA)+P1P2loge(V2/VB)+P1(V1−V2)+P1V1log(VA/V1),P2VB=P1V2,P2VA=P1V1W≠t=P2VB−P2VA+P1V2loge(P2/P1)+P1V1−P1V2−P1V1log(P1/P1)=P1(V2−V1)loge(P2/P1)
Topper's Solved these Questions
- INTERNAL ENERGY BOOK - ICSECHAPTER - INTERNAL ENERGY EXERCISE - SELECTED PROBLEMS (FROM HEAT ENGINES)
21 Videos
- INTERNAL ENERGY BOOK - ICSECHAPTER - INTERNAL ENERGY EXERCISE - SELECTED PROBLEMS (FROM ISOTHERMAL AND ADIABATIC PROCESSES )
10 Videos
- GRAVITATIONBOOK - ICSECHAPTER - GRAVITATIONEXERCISE - FROM THE HUBBLE TELESCOP
2 Videos
- MOTION IN FLUIDS BOOK - ICSECHAPTER - MOTION IN FLUIDS EXERCISE - SELECTED PROBLEMS (FROM POISEUILLE.S FORMULA)
19 Videos
Similar Questions
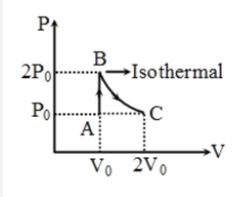
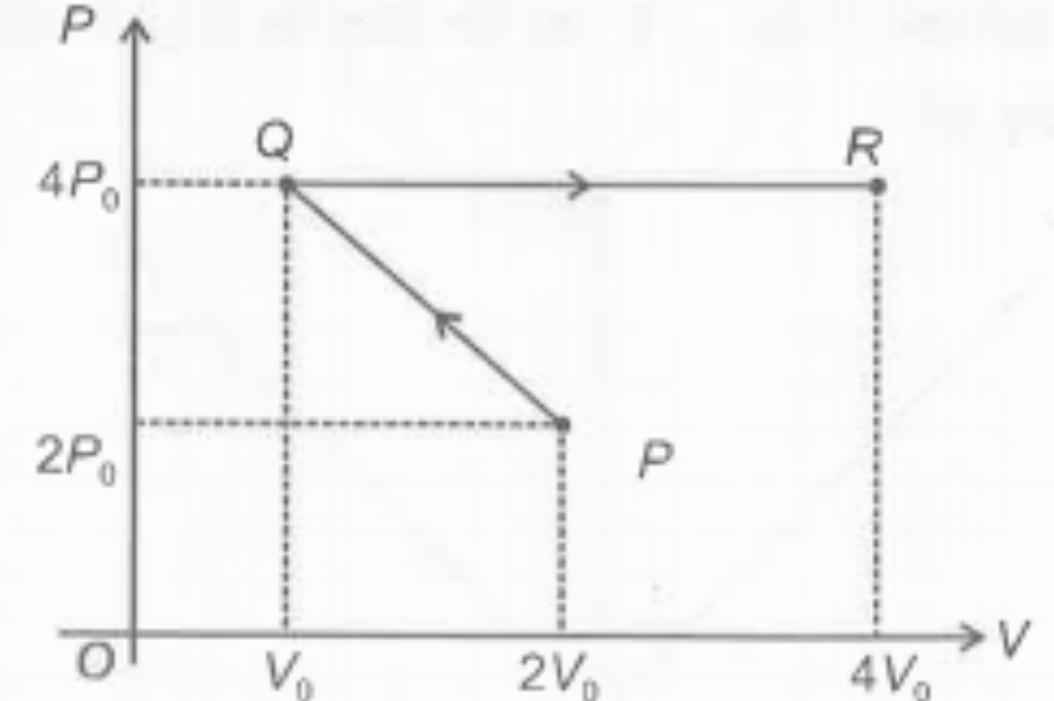
An ideal diatomic gas undergoes a thermodynamic process as shown in the P−V diagram. The process AB is isochoric while the process BC is isothermal. The total heat gein to the gas in the process is nearly (use ln2=0.7)
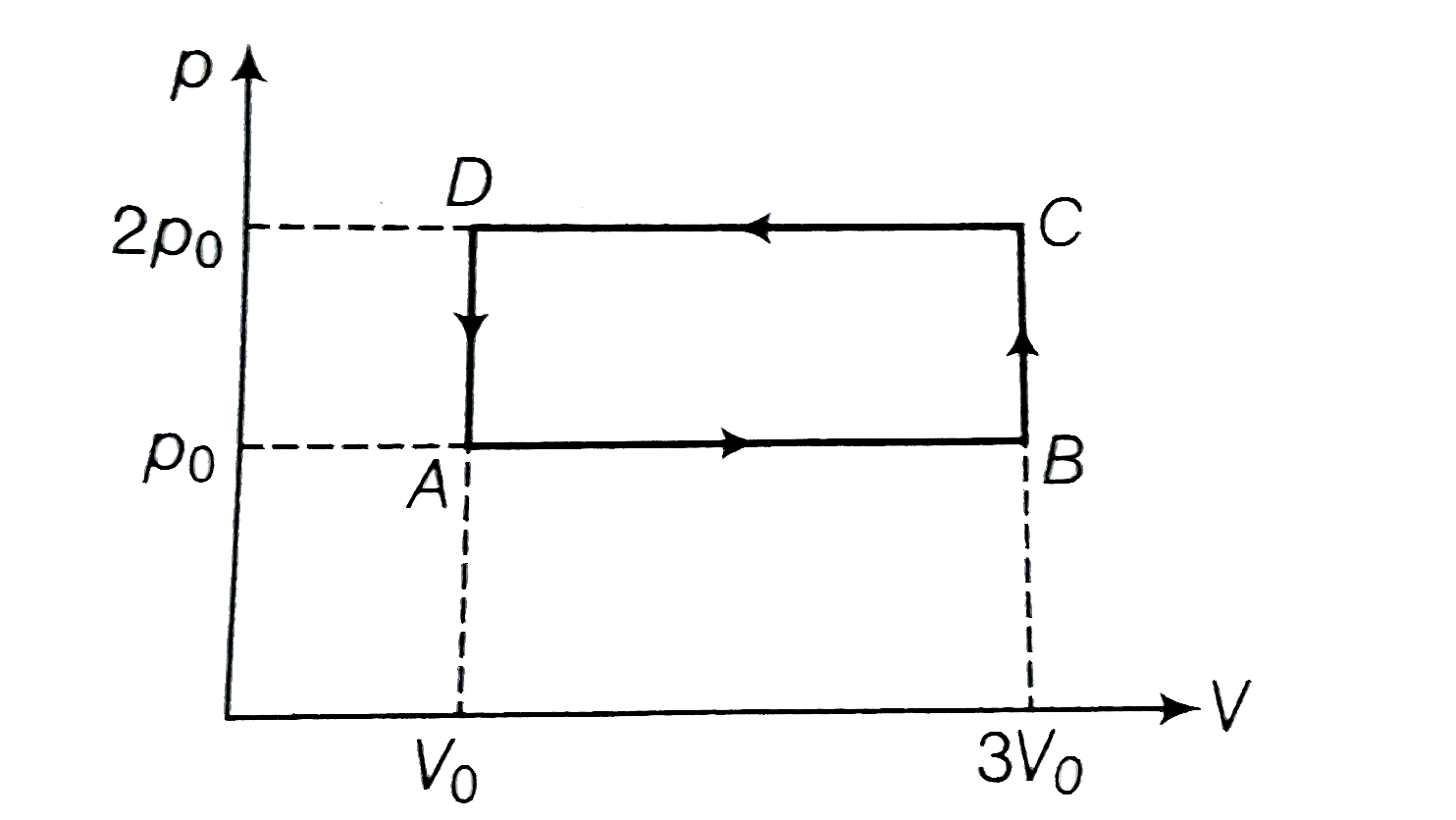
View SolutionAn ideal gas undergoes cyclic process ABCDA as shown in givend p−V diagram. The amount of work done by the gas is
View Solution
Knowledge Check
An ideal gas undergoes cyclic process ABCDA as shown in given p−V diagram. The amount of work done by the gas is
 A6P0V0B−2P0V0C+2P0V0D+4P0V0
A6P0V0B−2P0V0C+2P0V0D+4P0V0
An ideal gas is carried through a thermodynamic cycle consisting of two isobaric and two isothermal processes as shown in figure. Show that the net work done in the entire cycle is given by the equation. Wnet=p1(V2−V1)Inp2p1
View SolutionAn ideal monoatomic gas follows a thermodynamic process as shown in pressure-volume (P-V) plot. The work done in process AB is

View SolutionAn ideal gas undergoes a cyclic process as shown in the given P-T diagram, where AC is adiabatic. The process is also represented by:
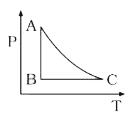
View SolutionAn ideal gas undergoes a process P→Q→R as shown in pressure (p) - volume (v) diagram.The work done by the gas is
View SolutionOne mole of an ideal monoatomic gas undergoes a cyclic process as shown in figure . Temperature at point 1 =300K and process 2−3 is isothermal .
_S01_113_O01.png) .
.
Net work done by gas in complete cycle is
View SolutionAn ideal gas (γ=1.5) undergoes a thermodynamic process in which the temperature and pressure of the gas are related as T−1P2= constant. The molar heat capacity of the gas during the process is
View SolutionOne mole of a diatomic gas undergoes a thermodynamic process, whose process equation is P∝V2. The molar specific heat of the gas is
View Solution
