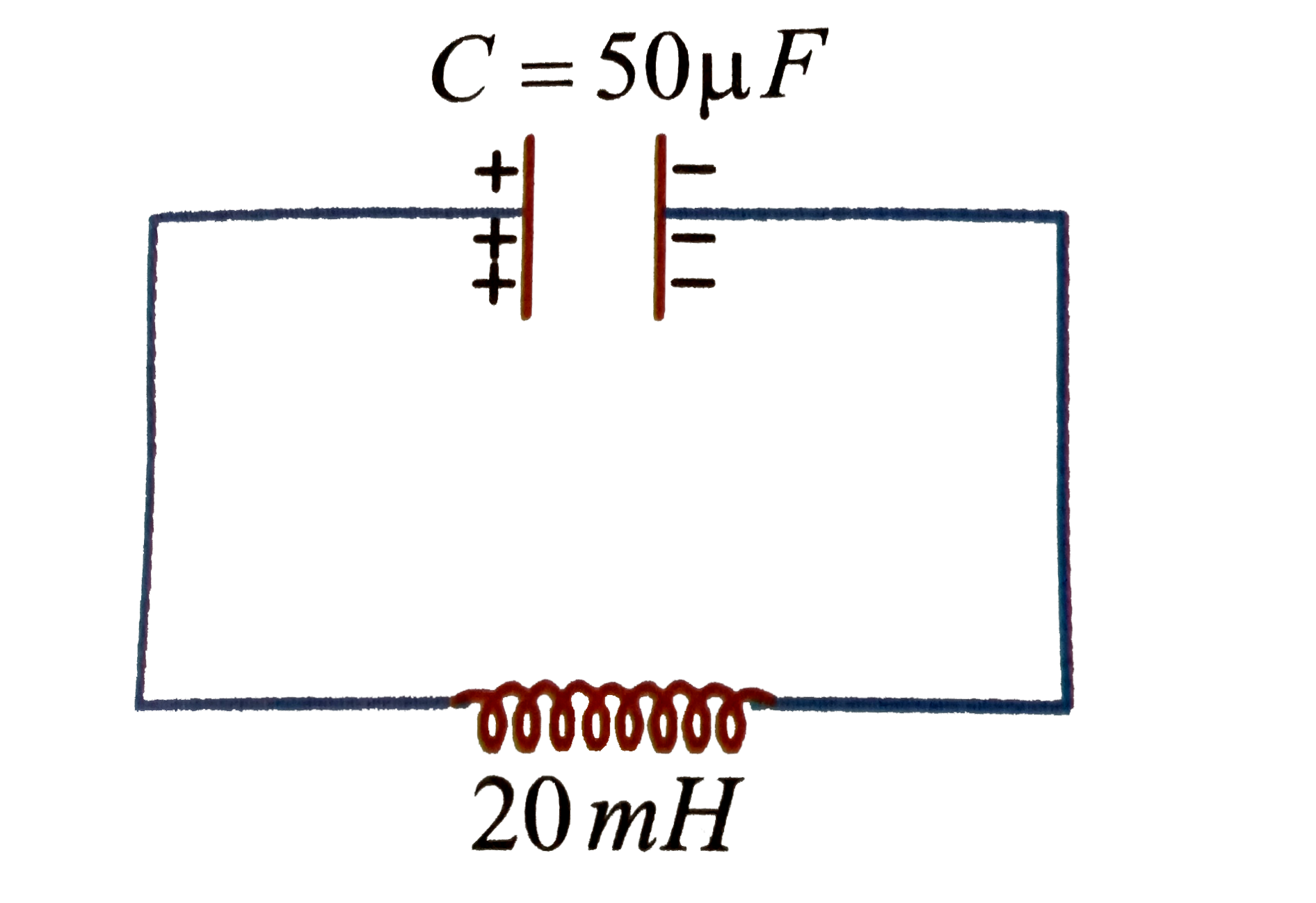
An LC circuit contains a 20 mH inductor and a 50 uF capacitor with an initial charge of 10 mC. The resistance of the circuit is negligible. Let the instant, the circuit is closed be t = 0.
(a) What is the total energy stored initially ? Is it conserved during LC oscillations ?
(b) What is the natural frequency of the circuit ?
(c) At what time is the energy stored
(i) completely electrical (i.e., stored in the capacitor)?
(ii) completely magnetic (i.e., stored in the inductor)?
(d) At what times is the total energy shared equally between the inductor and the capacitor ?
(e) If a resistor is inserted in the circuit, how much energy is eventually dissipated as heat ?

Text Solution

Verified by Experts

Here, L = 20 mH $= 20 \times 10^{- 3} H, C = 50 μ C = 50 \times 10^{- 6}$ F and $q_{m} = 10 m C = 10 \times 10^{- 3} C = 0.01 C$ , when t=0.
(a) $∴$ Total energy stored initially $U = \frac{1}{2} \frac{q_{0}^{2}}{C} = \frac{{(0.01)}^{2}}{2 \times 50 \times 10^{- 6}} = 1 J$
Yes, the energy remains conserved during LC oscillations provided that resistance R of the circuit is zero.
(b) Natural frequency of the circuit.
$v_{0} = \frac{1}{2 π \sqrt{L C}} = \frac{1}{2 \times 3.14 \times \sqrt{20 \times 10^{- 3} \times 50 \times 10^{- 6}}} = 159 H z$ and natural frequency $ω_{0} = 2 π v_{0} = 2 π \times 159 = 1000 r a d s^{- 1}$
( c) Let time period of oscillations be T, where T= $\frac{1}{v_{0}} = \frac{1}{159} = 6.3 \times 10^{- 3} s = 6.3 r m s$ then, (i) at times t=0, $\frac{T}{2}, T, \frac{3 t}{2}, 2 T$ .......... the energy stored is completely electrical and (ii) at times t = $\frac{T}{4}, \frac{3 T}{4}, \frac{5 T}{4}, \frac{7 T}{4}$ , ......... the energy stored is completely magnetic.
(d) Let at time t the electrical and magnetic energies are equal i.e.,
$\frac{q^{2}}{2 C} = \frac{1}{2} L i^{2} = \frac{1}{2} [(\frac{q_{0}^{2}}{2 C})]$ i.e. at time t, q = $\pm \frac{q_{0}}{\sqrt{2}}$
$∴ q = q_{0} cos ω$
$\Rightarrow \pm \frac{q_{0}}{\sqrt{2}} = q_{0} cos ω t$ or $cos ω t = \pm \frac{1}{\sqrt{2}}$ or $ω t = \frac{π}{4}, \frac{3 π}{4}, \frac{5 π}{4}$ .....
substituting $ω = \frac{2 π}{T}$ , we have $t = \frac{T}{8}, \frac{3 T}{8}, \frac{5 T}{8}, \frac{7 T}{8}$ ,.........
e) If a resistor is inserted in the circuit, continuously some of the initial energy (U=1) will be dissipated as heat energy. As a result, amplitude of LC oscillations gradually decreases with time and finally the oscillations stop altogether.

Updated on:21/07/2023

An $L C$ circuit contains a $20 m H$ inductor asn a $50 μ F$ capacitor with initial change of $10 m C$ . The resistance of the circuit is negligible. Let the instant the circuit is closed be $t = 0$ .

AEnergy stored in the circuit in completely electrical at $t = \frac{n π}{2000}$
BEnergy stored in the circuitin completely magnetic at $t = \frac{(2 n + 1) π}{2000}$
CEnergy stored in the circuit in shared equally between the inductor and capacitor at $t = \frac{(2 n + 1) π}{4000}$
DEnergy stored in the circuit is shared euqally between the inductor and capacitor at $t = \frac{n π}{2000}$
An LC circuit contains a 20 mH inductor and a $50 μ F$ capacitor with an initial charge of 10 mC. The resistance of the circuit is negligible. Let the instant at which the circuit which is closed be t=0. At what time the energy stored is completely magnetic ?
At = 0
B $t = 1.54 m s$
C $t = 3.14 m s$
D $t = 6.28 m s$
An LC circuit contains a 20 mH inductor and a $50 μ F$ capacitor with an initial charge of 10 mC. The resistance of the circuit is negligible. Let the instant at which the circuit which is closed be t=0. At what time the energy stored is completely magnetic ?
At = 0
B $t = 1.54 m s$
C $t = 3.14 m s$
D $t = 6.28 m s$