Question

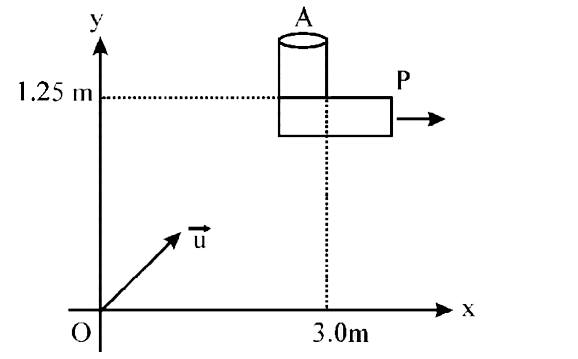
An object $A$ is kept fixed at the point $x=3m$ and $y=1.25m$ on a plank $p$ raised above the ground . At time $t=0$ the plank starts moving along the $+x$ direction with an acceleration $1.5m/s^2$. At the same instant a stone is projected from the origin with a velocity $\overrightarrow{u}$ as shown . A stationary person on the ground observes the stone hitting the object during its downward motion at an angle ${45}^{\circ }$ to the horizontal . All the motions are in the $X-Y$plane . Find $\overrightarrow{u}$ and the time after which the stone hits the object . Take $g=10m/s$

Answer

A::B::C

Explanation

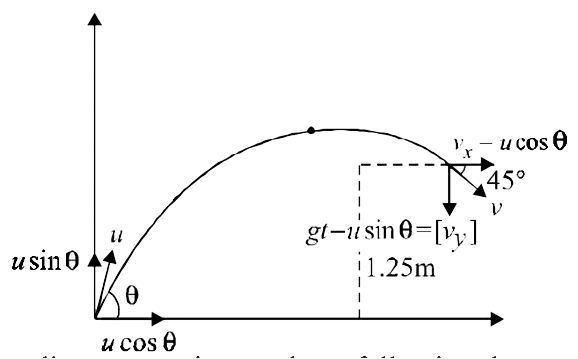
Let `'t'` be the time after which the stone hits the object and `theta` be the angle which the velocity vector `vec(u)` makes with horizontal .
According to question , we have following three conditions .
(i) Vertical displacement of the stone is `1.25 m`.
Therefore , `1.25 = ( u sin theta )t - 1/2 gt^(2)`
where ` g = 10 m//s^(2) `
or ` (u sin theta ) t = 1.25 + 5t^(2)` ...(i)
(ii) Horizontal displacement of stone `= 3+ displacement of object A`.
Therefore ,` (u cos theta )t = 3+ 0.75 t^(2) ` .....(ii)
(iii ) Horizontal component of velocity of stone = vertical component ( because velocity vector is inclined at ` 45(@)` with horizontal .)
Therfore `( u cos theta ) = gt - ( u sin theta ) ......(iii)
( The right hand side is written `gt -u sin theta ` because the stone is in its downward motion . Therefore , ` gt gt u sin theta `. In upward motion using ` thetagt gt`). Multiplying equation (iii) with `t` we can write ,
` ( u cos theta )t + ( u sin theta)t = 10 t^(2)` ....(iv)
Now , (iv) -(ii)-(i) gives `4.25 t^(2)- 4.25 = 0` or ` t = 1s `
Substituting `t = 1s` in (i) and (ii) , we get ,
` u sin theta = 6.25 m//s`
or ` u_(y) = 6.25 m//s or u_(y) =6.25 m//s`
and `u cos theta = 3.75 m//s `.
or ` u_(x) = 3.75 m//s` therfore ` vec (u) = u_(x)hat(i) + u_(y)hat(j) `
or ` vec(u) = ( 3.75 hat(i) + 6.25 hat(j) ) m//s `