An object of specific gravity is hung from a thin steel wire. The fundamental frequency for transverse standing waves in the wire is 300 Hz. The object is immersed in water, so that one half of its volume is submerged . The new fundamental frequency (in Hz) is
(a) 300
(b)
(c)
(d)
The correct Answer is:A
We know that
In air,
In water,
=
Therefore,
...(i)
=
and from Eqs (i).
Topper's Solved these Questions
- ARCHIVES 2 VOLUME 6BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ARCHIVES 2 VOLUME 6EXERCISE - Multiple Correct
22 Videos
- ARCHIVES 2 VOLUME 6BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ARCHIVES 2 VOLUME 6EXERCISE - Comprehension
12 Videos
- ARCHIVES 2 VOLUME 6BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ARCHIVES 2 VOLUME 6EXERCISE - True/False
4 Videos
- ARCHIVES 1 VOLUME 6BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ARCHIVES 1 VOLUME 6EXERCISE - Integer
4 Videos
- BASIC MATHEMATICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - BASIC MATHEMATICSEXERCISE - Exercise 2.6
20 Videos
Similar Questions
An object of specific gravity ρ is hung from a thin steel wire. The fundamental frequency for transverse standing waves in wire is 300Hz. The object is immersed in water so that one half of its volume is submerged. The new fundamental frequency in Hz is
View SolutionThe length of a stretched wire is 1 m and its fundamental frequency is 300 Hz.What is the speed of the transverse wave in the wire ?
View SolutionTwo wires of the same dimensions but resistivities ρ1 and ρ2 are connected in series. The equivalent resistivity of the combination is
View SolutionThe wires is of same dimension but resistivities ρ1 and ρ2 are connected in parallel . The equivalent resistivity of the combination is
View SolutionWill an object of density ρ float in a fluid of density 1.5 ρ? If yes what fraction of the object's volume will remain above the fluid?
View SolutionA body of density ρ floats with volume V1 of its total volume V immersed in a liquid of density ρ1 and with the remainder of volume V2 immersed in another liquid of density ρ2 where ρ1>ρ2. Find the volume immersed in two liquids. (V1 and V2).
View SolutionA closed organ pipe of length L and an open organ pipe contain gasses of densities ρ1 and ρ2, respectively. The compressibility of gasses are equal in both the pipes. Both the pipes are vibrating in their first overtone with same frequency . The length of the open organ pipe is
(a) L3
4l3
(c ) 4l3√ρ1ρ2
(d) 4l3√ρ2ρ1
View SolutionWhen two substances of specific gravities d1 and d2 are mixed in equal volumes the specific garvity of mixture is found to be ρ1. When they are mixed in equal masses, the specific gravity of the mixture is found to be ρ2.(d1≠d2)
View SolutionTwo discs have same mass and thickness. Their materials are of densities ρ1 and ρ2. The ratio of their moment of inertia about central axis will be
View SolutionThe velocity of the liquid coming out of a small hole of a vessel containing two different liquids of densities 2ρ and ρ as shown in the figure is
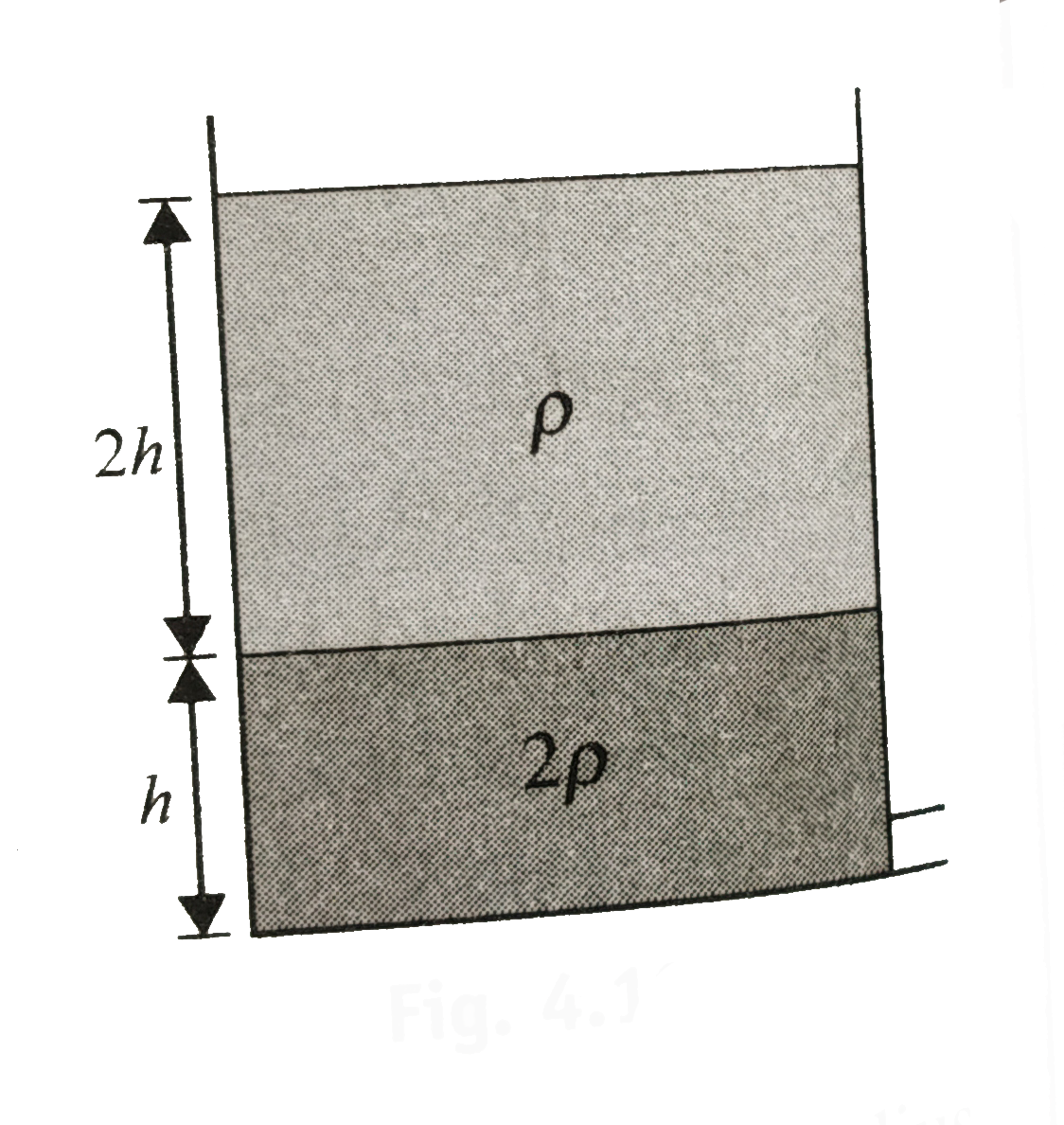
View Solution
