An organ pipe of (3.9π)mlong, open at both ends is driver to third harmonic standing wave. If the amplitude of pressure oscillation is 1% of mean atmospheric pressure [po=105N/m2]. The [Given, velocity of sound =200m/s and density of air =1.3kg/m3]
2.5cm
5cm
1cm
2cm
The correct Answer is:A
Δpmax=BAk ..(i)
ν=√Bρ
:. B=ρν2
l=3λ2
:. λ=2l3
k=2πλ=2π2l/3=3πl
=3π3.9π=11.3m−1

Subsiting in Eq. (i), we have
A=ΔpmaxBK=Δpmaxρν2k
=(0.01×105)1.3×(200)2×(1/1.3)
=0.025m=2.5cm
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - SOUND WAVESEXERCISE - Single correct option1videos
SOUND WAVES
- BOOK - DC PANDEYCHAPTER - SOUND WAVESEXERCISE - Level 2 More Than One Correct5videos
SOUND WAVES
- BOOK - DC PANDEYCHAPTER - SOUND WAVESEXERCISE - Subjective Questions1videos
SOUND WAVES
- BOOK - DC PANDEYCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)38videos
SOLVD PAPERS 2017 NEET, AIIMS & JIPMER
- BOOK - DC PANDEYCHAPTER - SUPERPOSITION OF WAVESEXERCISE - Level 2 Subjective8videos
SUPERPOSITION OF WAVES
Similar Questions
A 3m long organ pipe both at both ends is driven to third harmonic standing wave . If the amplitude of pressure oscillation is 0.1% of the mean atmospheric pressure (P0=105N/m2). Find the amplitude of
i. particle oscillation and
ii. density oscillation.
Speed of sound v=330m/s,densityofairρ0=1.0kg/m3View SolutionA 3m long organ pipe open at both ends is driven to third harmonic standing wave. If the ampulitude of pressure oscillations is 1 per cent of mean atmospheric pressure (po=105Nm2). Find the ampulited of particle displacement and density oscillations. Speed of sound υ=332m/s and density of air ρ=1.03kg/m3.
View Solution
Knowledge Check
- Question 1 - Select One
What is the density of a gas at N.T.P. if the rms velocity of the gas molecules is 400m/s? (Take atmospheric pressure P=1×105N/m2)
A58kg/m3B118kg/m3C158kg/m3D258kg/m2 - Question 2 - Select One
A pipe 30 cm long, is open at both ends. Which harmonic mode of the pipe resonates a 1.1 kHz source? (Speed of sound in air = 330 m s−1)
AFirstBThirdCSecondDFourth - Question 3 - Select One
A sound wave having a frequency of 100 Hz and pressure amplitude of 10 pa, then calculate the displacement amplitude (Given speed od sound in air = 340 m/s and density of air = 1.29 kgm3)
A3.63×10−5mB3×10−5mC4.2×10−5mD6.4×10−5m
Calculate the amplitude of vibrations of air particles when a wavee of intensity 10−3watt/m2 is produced by a tuning fork of frequency 540 Hz. Velocity of sound in air =340ms−1 and density of air =1.3kgm−3
View SolutionThe U-tube acts as a water siphon. The bend in the tube is 1m above the water surface. The tube outlet is 7m below the water surface. The water issues from the bottom of the siphon as a free jet at atmospheric pressure. Determine the speed of the free jet and the minimum absolute pressure of the water in the bend. Given atmospheric pressure =1.01×105N/m2,g=9.8m/s2 and density of water =103kg/m3
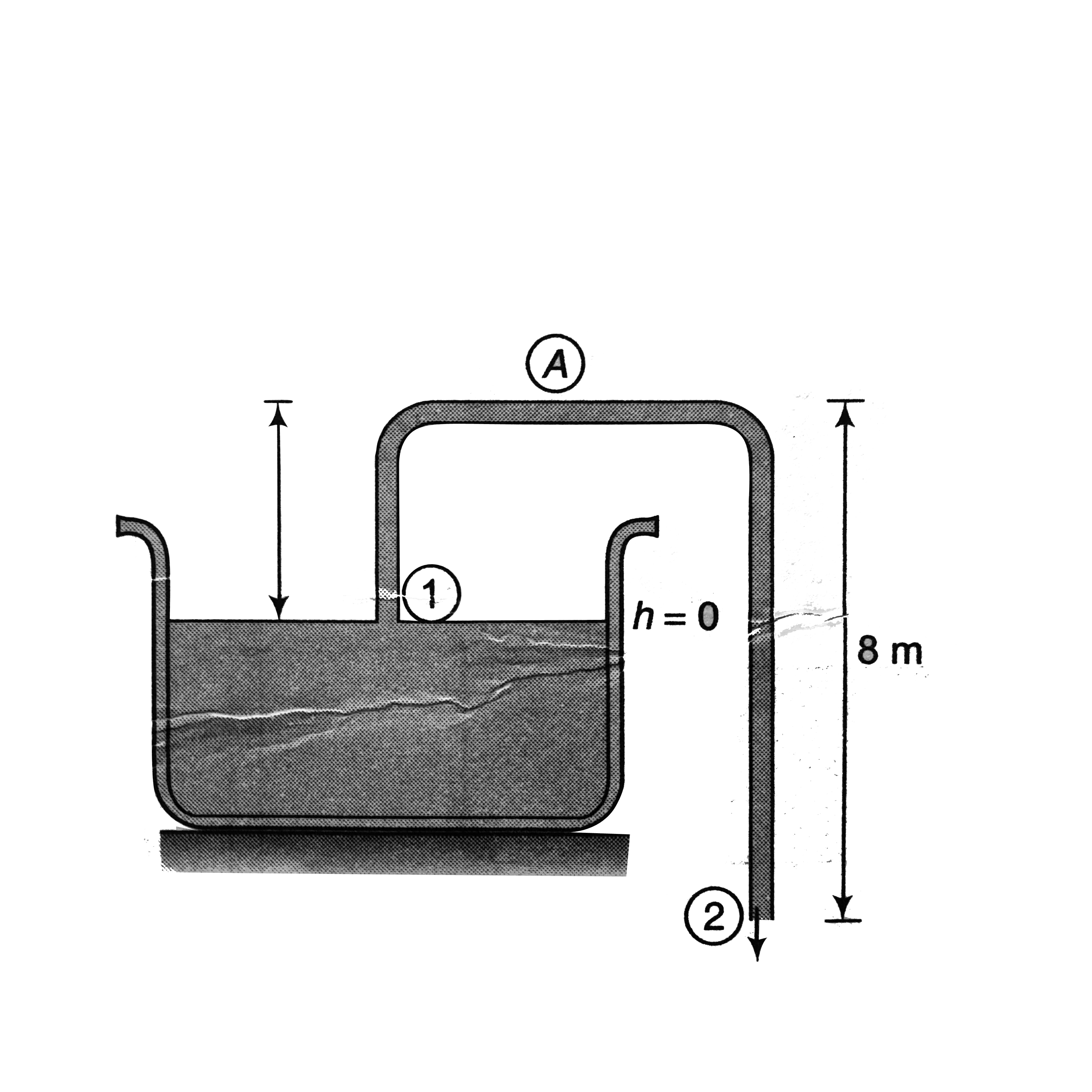 .View Solution
.View SolutionA sound wave in air has a frequency of 300HZ and a displacement ampulitude of 6.0×10−3mm. For this sound waves calculate the (a) Pressure ampulitude (b) intensity (c ) Sound intensity level (in dB)
Speed of sound =344m/s and density of air =1.2kg/m3.View SolutionAn organ pipe is open at both ends. It is producing sound at its third harmonic, the frequency of which is 262 Hz. The speed of sound is 343 m/s. What is the length of the pipe?
View SolutionIf the density of oxygen is 1.44 kg/m3 at pressure of 105N/m2, then the root-mean-square velocity of oxygen molecules in m/s will be
View Solution

