Arrive at the expression for the wavelength of electron in terms of electric potential. Solution in Kannada
We know that the wavelength associated with the accelerating electron,
i.e. λe=h√2emV=12.27√V Å
Hence λe∝1√V where V= is the electric potential.
Topper's Solved these Questions
- BOOK - SUBHASH PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - THREE MARK QUESTIONS WITH ANSWERS5videos
DUAL NATURE OF RADIATION AND MATTER
- BOOK - SUBHASH PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - FIVE MARK QUESTIONS WITH ANSWERS5videos
DUAL NATURE OF RADIATION AND MATTER
- BOOK - SUBHASH PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - NUMERICALS WITH SOLUTIONS21videos
DUAL NATURE OF RADIATION AND MATTER
- BOOK - SUBHASH PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - NUMERICAL PROBLEMS AND ANSWERS29videos
CURRENT ELECTRICITY
- BOOK - SUBHASH PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - NUMERICALS WITH SOLUTIONS21videos
ELECTRIC CHARGES AND FIELDS
Similar Questions
Mention any three properties of a photon.
01:32View SolutionWrite any three experimental observations of photoelectric effect
11:08View SolutionA photo has a wavelength of 5000 Å. Calculate its momentum.
03:02View SolutionIn the given circuit, calculate the effective resistance between A and B.
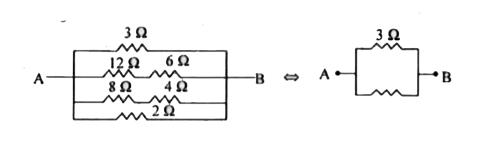 02:19View Solution
02:19View SolutionTwo point charges −2nC and +8nC are separated in air by a distance of 0.10m.Find the position of the neutral point along the line joining the 2 point charges.
03:30View SolutionExpress the unit of electric potential in terms of the basic units of S.I.
02:28View SolutionState the expression of the electrical conductivity of the material of a semiconductor in terms of the mobilities of electrons and holes.
06:36View SolutionWrite an expression for the wavelength acquired by an electron accelerated by a potential of V volts.
03:35View SolutionExpress electrostatic potential energy in terms of electric potential.
02:29View SolutionArrive at the expression for electric current established due to the motion of electron in its orbit.
02:37View SolutionArrive at the expression for the magnetic flux density at the centre of the circular path of an electron in terms of magnetic moment.
02:40View SolutionArrive at the expression for electric potential at a point due to a dipole and hence apply the expression for electric potential at a point on the dipole axis and on the equatorial lines.
02:29View SolutionDefine electric potential due to a point charge and arrive at the expression for the electric potential at a point due to a point charge.
02:29View SolutionWrite the expression for de-Broglie wavelength of electrons interms of electric potential and explain the terms used.
04:21View SolutionExpress electric potential (V) in terms of work done (W).
12:10View Solution

