Assertion A diabatic bulk modulus of an ideal gas in more than its isothermal bulk modulus.
Reason Both the modulii aer proportional to the pressure of gas at that moment.
If both Assertion and Reason are true and Reason is the correct explanation of Assertion.
If both Assertion and Reason are true but Reason is not the correct explanation of Assertion.
If Assertion is true but Reason is false.
If both Assertion and Reason are false.
The correct Answer is:B
To solve the question, we need to analyze the assertion and the reason provided, and then determine their validity.
1. Understanding Bulk Modulus:
- The bulk modulus (B) of a substance measures its resistance to uniform compression. It is defined as:
B=−PΔV/V0
- Here, P is the pressure, ΔV is the change in volume, and V0 is the original volume.
2. Isothermal Process:
- For an isothermal process (constant temperature), the relationship between pressure and volume is given by Boyle's Law:
PV=constant
- From this, we can derive that:
ΔPP+ΔVV=0
- Rearranging gives:
ΔPΔV=−PV
- Substituting this into the bulk modulus formula for isothermal conditions gives:
Biso=−V0(−PV)=P
3. Adiabatic Process:
- For an adiabatic process (no heat exchange), the relationship is given by:
PVγ=constant
- This leads to:
ΔPP+γΔVV=0
- Rearranging gives:
ΔPΔV=−γPV
- Substituting this into the bulk modulus formula for adiabatic conditions gives:
Badi=−V0(−γPV)=γP
4. Comparison of Bulk Moduli:
- From the calculations:
Biso=PandBadi=γP
- Since γ>1 (for any ideal gas), it follows that:
Badi>Biso
- Therefore, the assertion that the adiabatic bulk modulus of an ideal gas is greater than its isothermal bulk modulus is true.
5. Analyzing the Reason:
- The reason states that both moduli are proportional to the pressure of the gas at that moment.
- From our equations:
- Biso=P (proportional to pressure)
- Badi=γP (also proportional to pressure)
- Thus, the reason is also true.
6. Conclusion:
- Both the assertion and the reason are true, but the reason does not correctly explain the assertion. The assertion is about the relationship between the two bulk moduli, while the reason only states their proportionality to pressure.
Final Answer:
- The assertion is true, and the reason is true but does not provide a correct explanation for the assertion. Therefore, the correct option is B.
- The bulk modulus (B) of a substance measures its resistance to uniform compression. It is defined as:
B=−PΔV/V0
- Here, P is the pressure, ΔV is the change in volume, and V0 is the original volume.
- For an isothermal process (constant temperature), the relationship between pressure and volume is given by Boyle's Law:
PV=constant
- From this, we can derive that:
ΔPP+ΔVV=0
- Rearranging gives:
ΔPΔV=−PV
- Substituting this into the bulk modulus formula for isothermal conditions gives:
Biso=−V0(−PV)=P
- For an adiabatic process (no heat exchange), the relationship is given by:
PVγ=constant
- This leads to:
ΔPP+γΔVV=0
- Rearranging gives:
ΔPΔV=−γPV
- Substituting this into the bulk modulus formula for adiabatic conditions gives:
Badi=−V0(−γPV)=γP
- From the calculations:
Biso=PandBadi=γP
- Since γ>1 (for any ideal gas), it follows that:
Badi>Biso
- Therefore, the assertion that the adiabatic bulk modulus of an ideal gas is greater than its isothermal bulk modulus is true.
- The reason states that both moduli are proportional to the pressure of the gas at that moment.
- From our equations:
- Biso=P (proportional to pressure)
- Badi=γP (also proportional to pressure)
- Thus, the reason is also true.
- Both the assertion and the reason are true, but the reason does not correctly explain the assertion. The assertion is about the relationship between the two bulk moduli, while the reason only states their proportionality to pressure.
- The assertion is true, and the reason is true but does not provide a correct explanation for the assertion. Therefore, the correct option is B.
Similar Questions
Knowledge Check
Assertion: All molecules of an ideal gas more with the same speed.
Reason: There is no attraction between the molecules in an ideal gas.AIf both assertion and reason are true and reason is the correct explanation os assertion.BIf both assertion and reason are true but reason is not be correct explanation of assertion.CIf assertion is true but reason is false.DIf both assertion and reason are false.Assertion : Molar volume of an ideal gas at 273 . 15 K and 1 bar is 22.4 L.
Reason : Volume of a gas is inversely proportional to temperature .AIf both assertion and reason are true and reason is the correct explanation of assertion .BIf both assertion and reason are true but reason is not the correct explanation of assertion .CIf assertion is true but reason is false .DIf both assertion and reason are false .
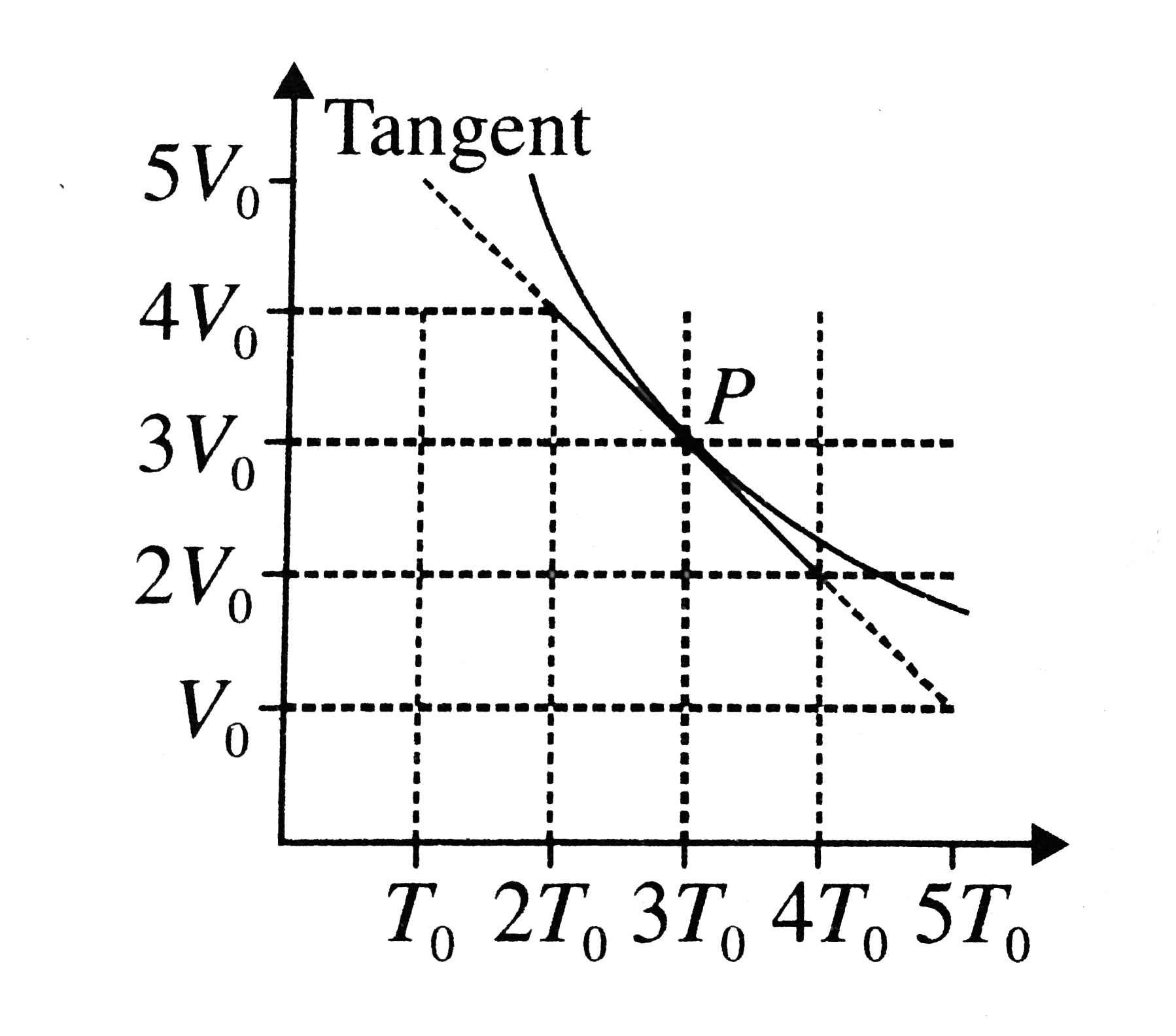
Figure shows the adiabatic curve for n moles of an ideal gas, the bulk modulus for the gas corresponding to the point P will be
View SolutionThe observed pressure of real gas is more than the ideal pressure.
View SolutionA given quantity of a ideal gas is at pressure P and absolute temperature T. The isothermal bulk modulus of the gas is
View SolutionA given quantity of a ideal gas is at pressure P and absolute temperature T. The isothermal bulk modulus of the gas is
View SolutionAssertion : The solubility of a gas in a liquid increases with increase of pressure.
Reason : The solubility of a gas in a liquid is directly proportional to the pressure of the gas.
View SolutionAssertion : Bulk modulus of an incompressible liquid is infinite.
Reason : Compressibility is inverse of bulk modulus.
View Solution
