Assertion: if a glass slab is placed in front of one of the slits, then fringe with will decreases.
Reason: Glass slab with produce an additional path difference.
The correct Answer is:D
To solve the question, we need to analyze both the assertion and the reason provided.
Step 1: Understand the Assertion
The assertion states that if a glass slab is placed in front of one of the slits, then the fringe width decreases.
Hint: Consider what happens to the interference pattern when a medium with a different refractive index is introduced.
Step 2: Analyze the Effect of the Glass Slab
When a glass slab is placed in front of one of the slits, it introduces a change in the optical path length due to its refractive index. The additional path difference created by the glass slab can be calculated using the formula:
Path Difference=(n−1)×t
where n is the refractive index of the glass and t is the thickness of the glass slab.
Hint: Think about how this additional path difference affects the interference pattern.
Step 3: Understand Fringe Width
Fringe width (β) in a double-slit experiment is given by the formula:
β=λDd
where λ is the wavelength of light used, D is the distance from the slits to the screen, and d is the distance between the slits.
Hint: Identify which parameters in the fringe width formula are affected by the introduction of the glass slab.
Step 4: Determine the Impact on Fringe Width
The introduction of the glass slab does not change the wavelength of the light (λ), the distance to the screen (D), or the distance between the slits (d). Therefore, the fringe width remains unchanged despite the introduction of the glass slab.
Hint: Reflect on how the path difference affects the position of the fringes but not their width.
Step 5: Conclusion
Since the assertion claims that the fringe width decreases, and our analysis shows that the fringe width remains unchanged, the assertion is false. The reason states that the glass slab produces an additional path difference, which is true.
Final Answer: The assertion is false, and the reason is true. Therefore, the correct answer is D (Assertion is false, Reason is true).
The assertion states that if a glass slab is placed in front of one of the slits, then the fringe width decreases.
When a glass slab is placed in front of one of the slits, it introduces a change in the optical path length due to its refractive index. The additional path difference created by the glass slab can be calculated using the formula:
Path Difference=(n−1)×t
where n is the refractive index of the glass and t is the thickness of the glass slab.
Fringe width (β) in a double-slit experiment is given by the formula:
β=λDd
where λ is the wavelength of light used, D is the distance from the slits to the screen, and d is the distance between the slits.
The introduction of the glass slab does not change the wavelength of the light (λ), the distance to the screen (D), or the distance between the slits (d). Therefore, the fringe width remains unchanged despite the introduction of the glass slab.
Since the assertion claims that the fringe width decreases, and our analysis shows that the fringe width remains unchanged, the assertion is false. The reason states that the glass slab produces an additional path difference, which is true.
Topper's Solved these Questions
- SOLVED PAPERS 2018Book:DC PANDEY ENGLISHChapter:SOLVED PAPERS 2018Exercise:JIPMER
Explore 22 Videos
- SOLVED PAPERS 2018Book:DC PANDEY ENGLISHChapter:SOLVED PAPERS 2018Exercise:AIIMS
Explore 22 Videos
- SOLVED PAPER 2017Book:DC PANDEY ENGLISHChapter:SOLVED PAPER 2017Exercise:Solved papers 2017(JIPMER)
Explore 32 Videos
- WAVE OPTICSBook:DC PANDEY ENGLISHChapter:WAVE OPTICSExercise:For JEE Advanced E. Integer Type Questions
Explore 13 Videos
Similar Questions
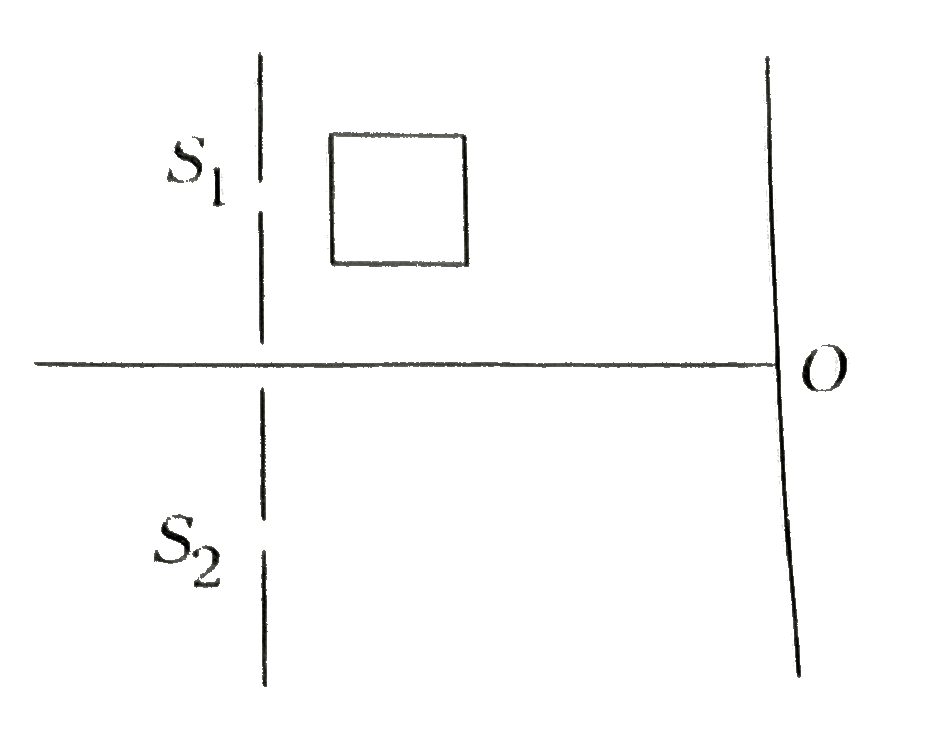
Assertion In the YDSE set up shown in figure a glass slab is inserted in front of the slit S1 as shown in figure Then zero order maxima (where path difference =0) will lie above point O.
Reason Glass slab will produce an extra path difference in the path of the ray passing through S1
View SolutionIn Young's double slit experiment, match the following two coloums.
Column IColumn IIAWhen width of one slit is slightly increasedp. maximum intensity will increaseBWhen one slit is closedq. maximum intensity will decreaseCWhen both the sources are made incoherentr. maximum intensity will remain sameDWhen a glass slab is inserted in front of one of the slitss. fringe pattern will displacement
Note Assume absorption from glass slab to be negligible
View Solution
Knowledge Check
Assertion : When a thin transparent sheet is placed in front of both the slits of Young's experiment, the fringe width will remains same.
Reason : In Young's experiment, the fringe width is directly proportional to wavelength of the source used.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true but reason is false.DIf both assertion and reason are false.
Assertion: White light is used in YDSE. Now, a glass slab is inserted in front of the slit S1. Then, red fringe will shift less (in upward direction) compared to violet.
Reason: Refractive index for violet colour will be more.
View SolutionAssertion In front of a concave mirror, a point object is placed between focus and centre of curvature. If a glass slab is placed between object and mirror, then image from mirror may become virtual.
Reason Glass slab always makes a virtual image of a real object.
View SolutionIn YDSE, both slits produce equal intensities on the screen. A 100% transparent thin film is placed in front of one of the slits. Now, the intensity on the centre becomes 75% of the previous intensity. The wavelength of light is 6000Å and refractive index of glass is 1.5. Thus, minimum thickness of the glass slab is
View SolutionA plane glass slab is placed over various coloured letters. The letter which appears to be raised the least is
View SolutionIn YDSE if a slab whose refractive index can be varied is placed in front of one of the slits. Then, the variation of resultant intensity at mid-point of screen with μ will be best represented by (mu is greater than or equal to 1)
View SolutionIn YDSE, find the thickness of a glass slab (μ=1.5) which
should be placed in front of the upper slit S1 so that the central maximum now
lies at a point where 5th bright fringe was lying earlier (before inserting the
slab). Wavelength of light used is 5000Å.
View SolutionAssertion: Image of an object is of same size by a convex lens. If a glass slab is placed between object and lens, image will become magnified.
Reason: By inserting the slab, image may be real or virtual.
View Solution
