In YDSE, find the thickness of a glass slab (μ=1.5) which
should be placed in front of the upper slit S1 so that the central maximum now
lies at a point where 5th bright fringe was lying earlier (before inserting the
slab). Wavelength of light used is 5000Å.
To find the thickness of a glass slab that should be placed in front of the upper slit S1 in a Young's Double Slit Experiment (YDSE), we can follow these steps:
1. Understanding the Problem:
We need to place a glass slab of refractive index μ=1.5 in front of slit S1 such that the central maximum shifts to the position of the 5th bright fringe.
2. Wavelength of Light:
The wavelength of the light used is given as λ=5000Å=5000×10−10m.
3. Fringe Width Calculation:
The position of the n-th bright fringe in YDSE is given by:
yn=nλDd
where D is the distance from the slits to the screen, and d is the distance between the slits.
4. Shift Due to Glass Slab:
When a glass slab of thickness T is placed in front of slit S1, the effective optical path difference introduced is:
Δ=(μ−1)T
where μ is the refractive index of the glass slab.
5. Setting Up the Equation:
The shift caused by the glass slab should equal the position of the 5th bright fringe:
Δ=5λDd
Therefore, we can equate:
(μ−1)T=5λDd
6. Substituting Values:
Substituting μ=1.5 and λ=5000Å:
(1.5−1)T=5×5000ÅDd
Simplifying gives:
0.5T=5×5000ÅDd
7. Solving for Thickness T:
Rearranging the equation:
T=5×5000Å×2Dd
Since Dd cancels out on both sides, we can focus on the numerical values:
T=5×5000×20.5
T=50,000Å
8. Final Answer:
The thickness of the glass slab T is:
T=50,000Å
Summary:
The thickness of the glass slab that should be placed in front of the upper slit S1 is 50,000Å.
We need to place a glass slab of refractive index μ=1.5 in front of slit S1 such that the central maximum shifts to the position of the 5th bright fringe.
The wavelength of the light used is given as λ=5000Å=5000×10−10m.
The position of the n-th bright fringe in YDSE is given by:
yn=nλDd
where D is the distance from the slits to the screen, and d is the distance between the slits.
When a glass slab of thickness T is placed in front of slit S1, the effective optical path difference introduced is:
Δ=(μ−1)T
where μ is the refractive index of the glass slab.
The shift caused by the glass slab should equal the position of the 5th bright fringe:
Δ=5λDd
Therefore, we can equate:
(μ−1)T=5λDd
Substituting μ=1.5 and λ=5000Å:
(1.5−1)T=5×5000ÅDd
Simplifying gives:
0.5T=5×5000ÅDd
Rearranging the equation:
T=5×5000Å×2Dd
Since Dd cancels out on both sides, we can focus on the numerical values:
T=5×5000×20.5
T=50,000Å
The thickness of the glass slab T is:
T=50,000Å
The thickness of the glass slab that should be placed in front of the upper slit S1 is 50,000Å.
Topper's Solved these Questions
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Example Type 1
2 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Example Type 2
2 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Medical entrance s gallery
59 Videos
Similar Questions
When a mica sheet of thickness 7 microns and μ=1.6 is placed in the path of one of interfering beams in the biprism expriment then the central fringe gets at the position of seventh bright fringe. What is the wavelength of light used?
View SolutionWith two slits spaced 0.2 mm apart and a screen at a distance of 1 m, the third bright fringe is found to be at 7.5 mm from the central fringe. The wavelength of light used is
View SolutionIn YDSE, slab of thickness t and refractive index μ is placed in front of any slit. Then displacement of central maximu is terms of fringe width when light of wavelength λ is incident on system is
View SolutionIn YDSE when slab of thickness t and refractive index μ is placed in front of one slit then central maxima shifts by one fringe width. Find out t in terms of λ and μ.
View SolutionFollowing figure shows the positions of the first bright fringes B1andB2 on either side of the central bright fringe O in Young.s double slit experiment. Find the wavelength of monochromatic light used.
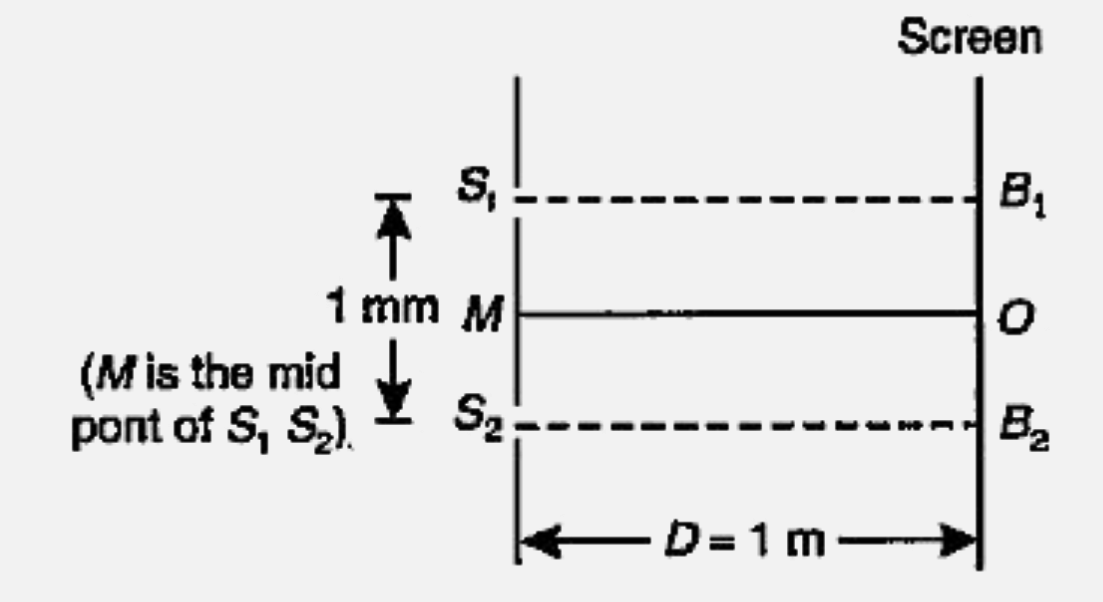
View SolutionOne slit of a double slits experiment is covered by a thin glass plate of refractive index1.4and the other by a thin glass plate of refractive index1.7 .The point on the screen ,where central bright fringe was formed before the introduction of the glass sheets,is now occupied by the 15thbright fringe.Assuming that both the glass plates have same thickness and wavelength of light used in 4800Å ,find the their thickness.
View SolutionA thin plastic of refractive index 1.6 is used to cover one of the slits of a double slit arrangement. The central point omn the screen is now occupied by what would have been the 7th bright fringe before the plastic was used. If the wavelength of light is 600 nm, what is the thickness ( in μm ) of the plastic?
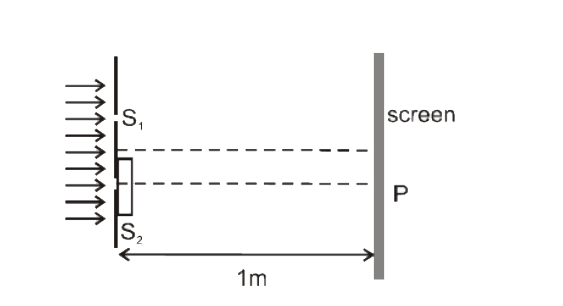
View SolutionIn standard Young's double - slit experiment, visible light λ∈(350nm,750nm) is used in both the slits. Distance between the slits is 2 mm, and the distance of the screen from the slits is 1 m. A thin glass slab (μ=1.5) of thickness 6μm is placed in front of slit S2. Which among the following wavelength is missing at point P, which is directly in front of slit S2? (Neglect dispersion of light)
View SolutionA plane mirror is made of glass slab (μg=1.5) 2.5 cm thick and silvered on back. A point object is placed 5 cm in front of the unsilvered face of the mirror. What will be the position of final image ?
View SolutionIn a Young's double slit experiment, a parallel beam containing wavelengths λ1=4000Å and λ2=5600Å is incident at an angle ϕ=30∘ on a diaphragm having narrow slits at separation d=2mm. The screen is placed at a distance D =40 cm from the slits. a mica slab of thickness t=5mm is placed in front of one of the slits and whole of the apparatus is submerged in water. if the central bright fringe is observed at C, determine.

(i) The refractive index of the slab
(ii) The distance of first black line from C
both wavelength are in air. take μw=43
View Solution
